Suppose We Estimate the Following Regression Using 48 Months of Data

Multiple Choice

Suppose we estimate the following regression using 48 months of data: Y_t = β₀ + β₁X_1t + β₂X_2t + β₃X_3t + β₄X_4t + ε_t
Using the residuals from this regression,we run another regression of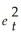
On the predicted values
_t. From this regression we get an R² of 0.14.Let H₀ be that there is no heteroscedasticity.What can you conclude?

A) Reject H₀ at α = 0.05,but not 0.025
B) Reject H₀ at α = 0.01,but not 0.005
C) Reject H₀ at α = 0.005
D) Unable to reject H₀ at α ≤ 0.10