Refer to the Figures Above

Multiple Choice

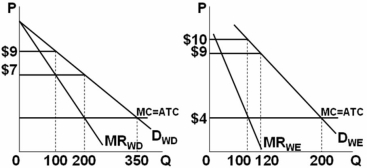Refer to the figures above.Suppose the graphs represent the demand for use of a local golf course for which there is no significant competition (it has a local monopoly) .P denotes the price of a round of golf and Q is the quantity of rounds sold each day.If the left graph represents the demand during weekdays and the right graph the weekend demand,how much economic profit will this profit-maximizing golf course earn over the course of a full seven-day week?

A) $4200
B) $3700
C) $3400
D) $2700
The monopolist should set MC = MR in both markets.That means the weekday output should be 200 with a price of $7 and the weekend output should be 100 with a price of $10.This means 5 days of 100 rounds each and 200 weekend rounds for a total of 1200.Average total cost is $4 per round,so weekday profits are $3 * 200 * 5 = $3000 and weekend profits are $6 * 200 = $1200.Total profits are $4200.