Solved

Which of the Following Is Appropriate to Conduct a Hypothesis

Question 16

Multiple Choice

Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling.


A) In every case.
B) Only if n1 ≥ 30 and n2 ≥ 30.
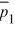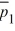C) Only if n1 Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. 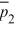≥ 5, n1(1 - Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. ) ≥ 5, n2
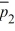Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. ≥ 5, and n2(1 - Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. ) ≥ 5.
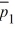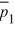D) Only if n1 Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. 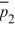≥ 30, n1(1 - Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. ) ≥ 30, n2
Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. ≥ 30, and n2(1 - 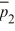Which of the following is appropriate to conduct a hypothesis test for the difference between two population proportions under independent sampling. A) In every case. B) Only if n<sub>1</sub> ≥ 30 and n<sub>2</sub> ≥ 30. C) Only if n<sub>1</sub> <sub> </sub> ≥ 5, n<sub>1</sub>(1 - ) ≥ 5, n<sub>2</sub> <sub> </sub> ≥ 5, and n<sub>2</sub>(1 - ) ≥ 5. D) Only if n<sub>1</sub> <sub> </sub> ≥ 30, n<sub>1</sub>(1 - ) ≥ 30, n<sub>2</sub> <sub> </sub> ≥ 30, and n<sub>2</sub>(1 - ) ≥ 30. ) ≥ 30.

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q11: A recent Health of Boston report suggests

Q12: We use the difference between the sample

Q13: A farmer uses a lot of fertilizer

Q14: A producer of fine chocolates believes that

Q15: A recent study from the University of

Q17: A tutor promises to improve GMAT scores

Q18: For a hypothesis test about the difference

Q19: A particular personal trainer works primarily with

Q20: According to a recent survey, women chat

Q21: A tutor promises to improve GMAT scores