Assume That Each Nurse Works 8 Consecutive Hours at the Beaver

Multiple Choice

Assume that each nurse works 8 consecutive hours at the Beaver Medical Center. The center has the following staffing requirements for each 4-hour work period. ​
Work Period
Nurses Needed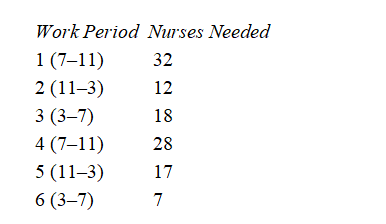

If represents the number of nurses starting in period 1, the number starting in period 2, and so on, write and solve the linear programming problem that will minimize the total number of nurses needed. (Note that the nurses who begin work in period 6 work periods 6 and 1 for their 8-hour shift.)
​

A) The minimum 67 is nurses with 27 nurses starting in period 1, 5 nurses starting in period 2, 18 nurses starting in period 3, 10 nurses starting in period 4, and 7 nurses starting in period 5 (no nurses need to start in period 6) .
B) The minimum is 67 nurses with 27 nurses starting in period 1, 18 nurses starting in period 3, and 10 nurses starting in period 4, 7 nurses starting in period 5, and 5 nurses starting in period 6 (no nurses need to start in period 2) .
C) The minimum is 67 nurses with 32 nurses starting in period 1, 18 nurses starting in period 3, and 10 nurses starting in period 4, and 7 nurses starting in period 5 (no nurses need to start in periods 2 and 6) .
D) The minimum is 72 nurses with 32 nurses starting in period 1, 18 nurses starting in period 3, and 10 nurses starting in period 4, and 12 nurses starting in period 5 (no nurses need to start in periods 2 and 6) .
E) The minimum is 62 nurses with 32 nurses starting in period 1, 13 nurses starting in period 3, and 10 nurses starting in period 4, and 7 nurses starting in period 5 (no nurses need to start in periods 2 and 6) .