The Linear Programming Problem Has an Unusual Characteristic

Multiple Choice

The linear programming problem has an unusual characteristic.Select a graph of the solution region for the problem and describe the unusual characteristic.Find the minimum value of the objective function (if possible) and where it occurs. ​
Objective function:
​
Z = -x + 2y
​
Constraints:
​
X ≥ 0
Y ≥ 0
X ≤ 10
X + y ≤ 8
​

A) ​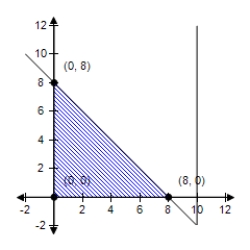 The constraint x ≤ 10 is extraneous.Minimum at (0,8) : 16
B) 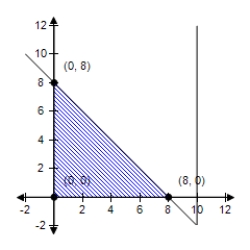The constraint x ≤ 10 is extraneous.Minimum at (8,0) : -8
C) 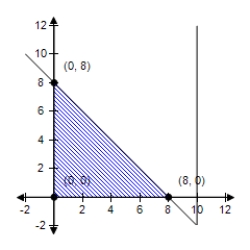The constraint x ≤ 10 is extraneous.No minimum.
D) 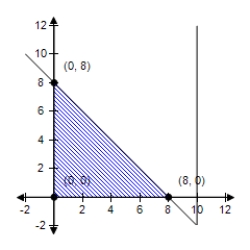The constraint x ≤ 10 is extraneous.Minimum at (8,8) : 8
E) 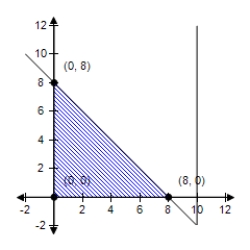The constraint x ≤ 10 is extraneous.Minimum at (0,0) : 0