When Two Railroad Tracks Merge,the Overlapping Portions of the Tracks $$\frac { x } { 2 } = 2 r \sin ^ { 2 } \frac { \theta } { 2 }$$

Multiple Choice

When two railroad tracks merge,the overlapping portions of the tracks are in the shapes of circular arcs (see figure) .The radius of each arc r (in feet) and the angle θ are related by​ $$\frac { x } { 2 } = 2 r \sin ^ { 2 } \frac { \theta } { 2 }$$ ​ Write a formula for x in terms of cos θ.​ ​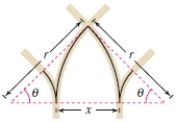

A) ​ $x = r \left( 1 - \cos \frac { \theta } { 2 } \right)$
B) ​ $x = r ( 1 - \cos \theta )$
C) $x = 2 r ( 1 - \cos \theta )$ ​
D) $x = 2 r ( 1 + \cos \theta )$ ​
E) $x = 2 r \left( 1 - \cos \frac { \theta } { 2 } \right)$ ​