Multiple Choice
Find the exact values of the three trigonometric functions sine,cosine,tangent of the real number t. 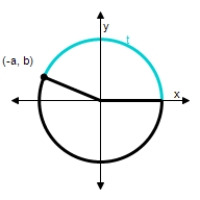
A)
B)
C)
D)
E)
Correct Answer:

Verified
Correct Answer:
Verified
Related Questions
Q1: Evaluate (if possible)the sine,cosec,and tangent of
Q2: Use the value of the trigonometric
Q3: Use the value of the trigonometric
Q4: Find the value of given trigonometric
Q5: Use the value of the trigonometric
Q7: Find the point (x,y)on the unit
Q8: Find the value of given trigonometric
Q9: Evaluate the trigonometric function using its
Q10: Find the value of given trigonometric
Q11: Use the value of the trigonometric