Multiple Choice
A local tire dealer wants to predict the number of tires sold each month.He believes that the number of tires sold is a linear function of the amount of money invested in advertising.He randomly selects 6 months of data consisting of tire sales (in thousands of tires) and advertising expenditures (in thousands of dollars) .Based on the data set with 6 observations,the simple linear regression equation of the least squares line is 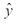 = 3 + 1x.
= 3 + 1x. 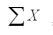 = 24
= 24 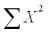 = 124
= 124 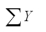 = 42
= 42 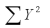 = 338
= 338 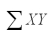 = 196 MSE = 4
= 196 MSE = 4
Using the sums of the squares given above,determine the 95% confidence interval for the slope.
A) (1.951 4.049)
B) (-4.552 6.552)
C) (-.0492 2.0492)
D) (0.259 1.741)
Correct Answer:

Verified
Correct Answer:
Verified
Q78: The standard error of the estimate (standard
Q102: A local tire dealer wants to predict
Q103: A local tire dealer wants to predict
Q104: Consider the following partial computer output from
Q105: A _ is a method or a
Q109: Regression Analysis <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB1737/.jpg" alt="Regression Analysis
Q110: A local tire dealer wants to predict
Q111: Regression Analysis <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB1737/.jpg" alt="Regression Analysis
Q112: Consider the following partial computer output from
Q114: The simple linear regression (least squares method)minimizes:<br>A)The