Solved

Calculate the T Statistic for Testing Equality of Means Where

Question 58

Essay

Calculate the t statistic for testing equality of means where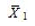
Calculate the t statistic for testing equality of means where = 8.2, = 11.3,s<sub>1</sub><sup>2</sup> = 5.4,s<sub>2</sub><sup>2</sup> = 5.2,n<sub>1</sub> = 6,and n<sub>2</sub> = 7.(Assume equal population variances. )= 8.2,
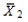Calculate the t statistic for testing equality of means where = 8.2, = 11.3,s<sub>1</sub><sup>2</sup> = 5.4,s<sub>2</sub><sup>2</sup> = 5.2,n<sub>1</sub> = 6,and n<sub>2</sub> = 7.(Assume equal population variances. )= 11.3,s12 = 5.4,s22 = 5.2,n1 = 6,and n2 = 7.(Assume equal population variances. )

Correct Answer:

verifed

Verified

−2.42
s2 = [(6 − 1)(5.4)+ (7 − ...

View Answer

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q9: In testing the equality of population variances,

Q53: Find a 90 percent confidence interval for

Q54: A new company is in the process

Q55: Let p<sub>1</sub> represent the population proportion of

Q61: Assume that we are constructing a confidence

Q62: When comparing the variances of two normally

Q69: Let p<sub>1</sub> represent the population proportion of

Q78: An experiment in which two different measurements

Q89: In testing the difference between two means

Q112: If the limits of the confidence interval