Solved

The Following Function Is a Counterexample for the Converse of the Intermediate

Question 10

Multiple Choice

The following function is a counterexample for the converse of the Intermediate Value Theorem, which states:
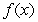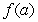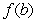If The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. assumes all the values between The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. 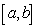and The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. 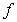in the interval The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. 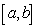, then The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. 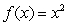is continuous on The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. :


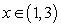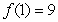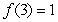
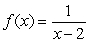A) The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. for The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. 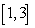, The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. 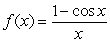, The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct.
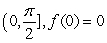
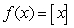B) The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. on The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct.
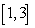
C) The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. on The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct.
D) The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct. on The following function is a counterexample for the converse of the Intermediate Value Theorem, which states: If assumes all the values between and in the interval , then is continuous on : A) for , , B) on C) on D) on E) A and C are correct.
E) A and C are correct.

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q5: Determine the points where the function is

Q6: The greatest integer function is defined by

Q7: The polynomial <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5596/.jpg" alt="The polynomial

Q8: The position of a particle is given

Q9: Let <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5596/.jpg" alt="Let denote

Q11: Determine whether the following statement is correct.

Q12: Show that <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5596/.jpg" alt="Show that

Q13: Compute the following one-sided limits:<br>A) <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5596/.jpg"

Q14: Find <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5596/.jpg" alt="Find ."

Q15: Evaluate the limit <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5596/.jpg" alt="Evaluate the