Multiple Choice
A second order differential equaiton can be arranged to the form 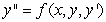 , and one can find the third and higher derivatives of y by simply differentiating this equation. Since a Taylor series expansion of a function y(x) is
, and one can find the third and higher derivatives of y by simply differentiating this equation. Since a Taylor series expansion of a function y(x) is 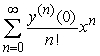 , one can differentiate the rearranged second order differential equation to evaluate coefficients of the Taylor polynomial, if one is either given or can solve for the initial condition y(0) and y'(0) . What does the fourth-degree Taylor polynomial look like for the solution to the equation
, one can differentiate the rearranged second order differential equation to evaluate coefficients of the Taylor polynomial, if one is either given or can solve for the initial condition y(0) and y'(0) . What does the fourth-degree Taylor polynomial look like for the solution to the equation 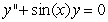 if the initial conditions are
if the initial conditions are 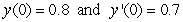 ?
?
A) 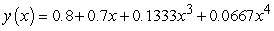
B) 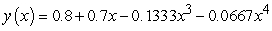
C) 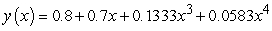
D) 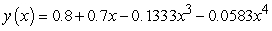
Correct Answer:

Verified
Correct Answer:
Verified
Q12: A series circuit has a 0.1 henry
Q13: Find the general solution of the differential
Q14: Identify the general solution of the the
Q15: Identify the radius of convergence of the
Q16: A pendulum has length 0.22 meter. A
Q18: Consider solutions to the second order differential
Q19: A series circuit has a 0.1 henry
Q20: Find the general solution of <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5869/.jpg"
Q21: Find the recurrence relation for the general
Q22: A 0.15 kg mass hangs on a