Multiple Choice
A box is to be constructed out of 16 square feet of material. Suppose the bottom of the box must be reinforced by doubling up the material (essentially, there are two bottoms of the box) . Find the dimensions l, w, and h that maximizes the volume of the box.
A) 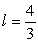 ft,
ft, 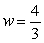 ft,
ft, 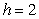 ft
ft
B)  ft,
ft, 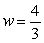 ft,
ft, 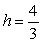 ft
ft
C) 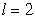 ft,
ft, 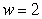 ft,
ft, 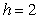 ft
ft
D) 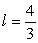 ft,
ft, 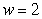 ft,
ft,  ft
ft
Correct Answer:

Verified
Correct Answer:
Verified
Q48: Find the indicated partial derivative. <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5869/.jpg"
Q49: Compute the indicated function value. <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5869/.jpg"
Q50: Find the gradient of the given function.
Q51: Use a graphing utility to sketch the
Q52: Find a function with the given properties.
Q54: Find the gradient of the given function.
Q55: Use the chain rule to find the
Q56: Find an equation of the tangent plane
Q57: Find the total differential of <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5869/.jpg"
Q58: Show that the function <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB5869/.jpg" alt="Show