A Stirred Tank with Volume, V, Has a Feed Stream

Multiple Choice

A stirred tank with volume, V, has a feed stream of concentrated floor cleaner flowing into it at a rate of f . The flow stream has a concentration of c_i. The outlet stream also flows at f but with a concentration of c, the concentration of the floor cleaner solution in the tank. The rate of change of the concentration is proportional to the difference between c_i and c with proportionality constant of f/V: 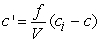. If the tank volume is 2000 L, the flow rate is 6 L/min, the inlet concentration is 0.9, and the initial concentration of the floor cleaner in the tank is 0.0, how long until the concentration in the tank is 0.7?

A) 9.0 minutes
B) 501.4 minutes
C) 333.3 minutes
D) 83.8 minutes