Deck 12: Experimental Design and Analysis of Variance
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/132
Play
Full screen (f)
Deck 12: Experimental Design and Analysis of Variance
1
In a completely randomized (one-way) ANOVA, with other things being equal, as the sample means get closer to each other, the probability of rejecting the null hypothesis decreases.
True
2
When using a randomized block design, the interaction effect between the block and treatment factors cannot be separated from the error term.
True
3
The error sum of squares measures the between-treatment variability.
False
4
In one-way ANOVA, other factors being equal, the further apart the treatment means are from each other, the more likely we are to reject the null hypothesis associated with the ANOVA F test.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
5
In one-way ANOVA, the numerator of the F statistic is an estimate of the population variance based on between-treatment variation.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
6
In a 2-way ANOVA, treatment is considered to be a combination of a level of factor 1 and a level of factor 2.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
7
If sample mean plots look essentially parallel, we can intuitively conclude that there is an interaction between the two factors.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
8
The 95 percent individual confidence interval for μ1 − μ2 (treatment mean 1 − treatment mean 2) will always be smaller than the Tukey 95 percent simultaneous confidence interval for μ1 − μ2.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
9
A one-way analysis of variance is a method that allows us to estimate and compare the effects of several treatments on a response variable.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
10
The experimentwise α for the 95 percent individual confidence interval for μ1 − μ2 (treatment mean 1 − treatment mean 2) will always be smaller than the experimentwise α for a Tukey 95 percent simultaneous confidence interval for μ1 − μ2.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
11
If the factors being studied cannot be controlled, the data are said to be observational.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
12
The ANOVA procedure for a two-factor factorial experiment partitions the total sum of squares into three components, SS first factor, SS second factor, and SSE.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
13
In one-way ANOVA, a large value of F results when the within-treatment variability is large compared to the between-treatment variability.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
14
In one-way ANOVA, as the between-treatment variation decreases, the probability of rejecting the null hypothesis increases.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
15
Experimental data are collected so that the values of the dependent variables are set before the values of the independent variable are observed.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
16
In one-way ANOVA, the numerator degrees of freedom equals the number of samples being compared.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
17
After rejecting the null hypothesis of equal treatments, a researcher decided to compute a 95 percent confidence interval for the difference between the mean of treatment 1 and mean of treatment 2 based on the Tukey procedure. At α = .05, if the confidence interval includes the value of zero, then we can reject the hypothesis that the two population means are equal.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
18
Interaction exists between two factors if the relationship between the mean response and one factor depends on the other factor.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
19
In a 2-way ANOVA, if factor 1 has a levels and factor 2 has b levels, then there is a total of ________ treatments.
A) a + b
B) a × b
C) |a − b|
D) a/b
E) a
A) a + b
B) a × b
C) |a − b|
D) a/b
E) a

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
20
Different levels of a factor are called ________.
A) treatments
B) variables
C) responses
D) observations
A) treatments
B) variables
C) responses
D) observations

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
21
When using a completely randomized design (one-way analysis of variance), the calculated F statistic will decrease as
A) the variability among the groups decreases relative to the variability within the groups.
B) the total variability increases.
C) the total variability decreases.
D) the variability among the groups increases relative to the variability within the groups.
A) the variability among the groups decreases relative to the variability within the groups.
B) the total variability increases.
C) the total variability decreases.
D) the variability among the groups increases relative to the variability within the groups.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
22
ANOVA table 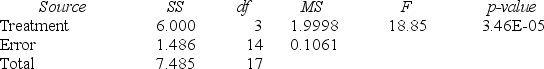 Post hoc analysis
Post hoc analysis
Tukey simultaneous comparison t-values
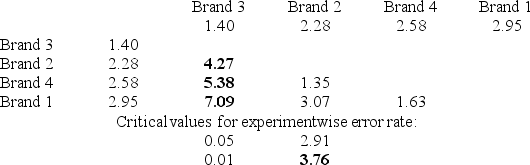 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
At a significance level of .05, we would
A) not be able to reject the null hypothesis of equal population means.
B) reject the null hypothesis of equal population means.
C) reject or fail to reject depending on the value of the t statistic.
D) not be able to decide whether or not to reject the null hypothesis due to insufficient information.
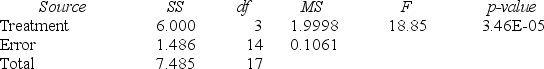 Post hoc analysis
Post hoc analysisTukey simultaneous comparison t-values
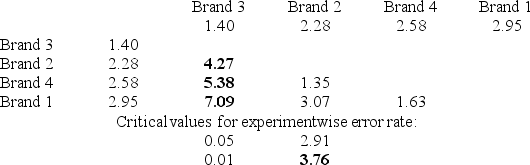 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.At a significance level of .05, we would
A) not be able to reject the null hypothesis of equal population means.
B) reject the null hypothesis of equal population means.
C) reject or fail to reject depending on the value of the t statistic.
D) not be able to decide whether or not to reject the null hypothesis due to insufficient information.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
23
When we compute 100(1 − α) confidence intervals, the value of α is called the
A) comparisonwise error rate.
B) Tukey simultaneous error rate.
C) experimentwise error rate.
D) pairwise error rate.
A) comparisonwise error rate.
B) Tukey simultaneous error rate.
C) experimentwise error rate.
D) pairwise error rate.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
24
________ simultaneous confidence intervals test all of the pairwise differences between means, respectively, while controlling the overall Type I error.
A) Randomized
B) Tukey
C) Covariate
D) Interacting
A) Randomized
B) Tukey
C) Covariate
D) Interacting

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
25
We have just performed a one-way ANOVA on a given set of data and did not reject the null hypothesis for the ANOVA F test. Assume that we are able to perform a randomized block design ANOVA on the same data. For the randomized block design ANOVA, the null hypothesis for equal treatments will ________ be rejected.
A) always
B) sometimes
C) never
A) always
B) sometimes
C) never

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
26
When computing individual confidence intervals using the t statistic, for all possible pairwise comparisons of means, the experimentwise error rate will be
A) equal to α.
B) less than α.
C) greater than α.
A) equal to α.
B) less than α.
C) greater than α.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
27
In the one-way ANOVA, the treatment sum of squares equals
A) SSTO − SS(error) − SS(interaction).
B) SSTO − SS(factor 1) − SSE.
C) SSTO − SS(interaction) − SS(factor 1) − SS(factor 2).
D) SSTO − SS(factor 1) − SS(factor 2).
E) SSTO − SS(error).
A) SSTO − SS(error) − SS(interaction).
B) SSTO − SS(factor 1) − SSE.
C) SSTO − SS(interaction) − SS(factor 1) − SS(factor 2).
D) SSTO − SS(factor 1) − SS(factor 2).
E) SSTO − SS(error).

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
28
ANOVA table 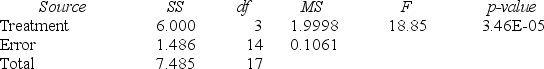 Post hoc analysis
Post hoc analysis
Tukey simultaneous comparison t-values
 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
At a significance level of .05, the null hypothesis for the ANOVA F test is rejected. Analysis of the Tukey simultaneous confidence intervals shows that at the significance level (experimentwise) of .05, we would conclude that
A) all four brands of vacuum cleaners differ from each other in terms of their performance.
B) brand 1 differs from brand 2, and brand 2 differs from brand 3, while the rest of the vacuum cleaner pairs do not differ from each other in terms of their performance.
C) brand 1 differs from brand 2, and brand 3 differs from brands 1, 2, and 4, while the rest of the vacuum cleaner pairs do not differ from each other in terms of their performance.
D) only brand 3 differs from the other three brands (brands 1, 2, and 3), while the rest of the vacuum cleaner pairs do not differ from each other in terms of their performance.
E) none of the four brands of vacuum cleaners differ from each other in terms of their performance.
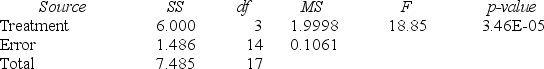 Post hoc analysis
Post hoc analysisTukey simultaneous comparison t-values
 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.At a significance level of .05, the null hypothesis for the ANOVA F test is rejected. Analysis of the Tukey simultaneous confidence intervals shows that at the significance level (experimentwise) of .05, we would conclude that
A) all four brands of vacuum cleaners differ from each other in terms of their performance.
B) brand 1 differs from brand 2, and brand 2 differs from brand 3, while the rest of the vacuum cleaner pairs do not differ from each other in terms of their performance.
C) brand 1 differs from brand 2, and brand 3 differs from brands 1, 2, and 4, while the rest of the vacuum cleaner pairs do not differ from each other in terms of their performance.
D) only brand 3 differs from the other three brands (brands 1, 2, and 3), while the rest of the vacuum cleaner pairs do not differ from each other in terms of their performance.
E) none of the four brands of vacuum cleaners differ from each other in terms of their performance.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
29
In a completely randomized (one-way) analysis of variance problem with c groups and a total of n observations in all groups, the variance between groups is equal to
A) (Total sum of squares) − (Sum of squares within columns).
B) (Sum of squares between columns)/(c − 1).
C) (Total sum of squares) − [(Sum of squares within columns)/(n − c)].
D) [(Total sum of squares)/(n − 1)] − [(Sum of squares between columns)/(c − 1)].
A) (Total sum of squares) − (Sum of squares within columns).
B) (Sum of squares between columns)/(c − 1).
C) (Total sum of squares) − [(Sum of squares within columns)/(n − c)].
D) [(Total sum of squares)/(n − 1)] − [(Sum of squares between columns)/(c − 1)].

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
30
When computing a confidence interval for the difference between two means, the width of the (1 − α) confidence interval based on the Tukey procedure will be ________ the width of the (1 − α) individual confidence interval based on the t statistic.
A) greater than
B) less than
C) the same as
D) sometimes greater than, sometimes less than
A) greater than
B) less than
C) the same as
D) sometimes greater than, sometimes less than

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
31
The advantage of the randomized block design over the completely randomized design is that we are comparing the treatments by using ________ experimental units.
A) randomly selected
B) the same
C) different
D) representative
E) equally timed
A) randomly selected
B) the same
C) different
D) representative
E) equally timed

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
32
A sum of squares that measures the total amount of variability in the observed values of the response variable is referred to as the
A) treatment sum of squares.
B) error sum of squares.
C) sum of squares within-treatment.
D) total sum of squares.
E) interaction sum of squares.
A) treatment sum of squares.
B) error sum of squares.
C) sum of squares within-treatment.
D) total sum of squares.
E) interaction sum of squares.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
33
When computing confidence intervals using the Tukey procedure, for all possible pairwise comparisons of means, the experimentwise error rate will be
A) equal to α.
B) less than α.
C) greater than α.
A) equal to α.
B) less than α.
C) greater than α.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
34
In the randomized block ANOVA, the sum of squares for factor 1 equals
A) SSTO − SS(error) − SS(interaction).
B) SSTO − SS(factor 2) − SSE.
C) SSTO − SS(interaction) − SS(factor 2).
D) SSTO − SS(factor 2).
E) SSTO − SS(error).
A) SSTO − SS(error) − SS(interaction).
B) SSTO − SS(factor 2) − SSE.
C) SSTO − SS(interaction) − SS(factor 2).
D) SSTO − SS(factor 2).
E) SSTO − SS(error).

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
35
In a completely randomized ANOVA, with other things equal, as the sample means get closer to each other, the probability of rejecting the null hypothesis
A) decreases.
B) increases.
C) is unaffected.
A) decreases.
B) increases.
C) is unaffected.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
36
We have just performed a one-way ANOVA on a given set of data and rejected the null hypothesis for the ANOVA F test. Assume that we are able to perform a randomized block design ANOVA on the same data. For the randomized block design ANOVA, the null hypothesis for equal treatments will ________ be rejected.
A) always
B) sometimes
C) never
A) always
B) sometimes
C) never

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
37
After analyzing a data set using the one-way ANOVA model, the same data are analyzed using the randomized block design ANOVA model. SS (Treatment) in the one-way ANOVA model is ________ the SS (Treatment) in the randomized block design ANOVA model.
A) always equal to
B) always greater than
C) always less than
D) sometimes greater than
A) always equal to
B) always greater than
C) always less than
D) sometimes greater than

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
38
A sum of squares that measures the variability among the sample means is referred to as the
A) treatment sum of squares.
B) error sum of squares.
C) sum of squares within-treatment.
D) total sum of squares.
E) interaction sum of squares.
A) treatment sum of squares.
B) error sum of squares.
C) sum of squares within-treatment.
D) total sum of squares.
E) interaction sum of squares.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
39
Which one of the following is not an assumption of one-way analysis of variance?
A) random selection of samples from each population
B) equality of the population variances
C) equality of the population means
D) Samples selected from each treatment population all have normal distributions.
A) random selection of samples from each population
B) equality of the population variances
C) equality of the population means
D) Samples selected from each treatment population all have normal distributions.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
40
Which of the following is not an assumption for one-way analysis of variance?
A) The p populations of values of the response variable associated with the treatments have equal variances.
B) The samples of experimental units associated with the treatments are randomly selected.
C) The experimental units associated with the treatments are independent samples.
D) The number of sampled observations must be equal for all p treatments.
E) The distribution of the response variable is normal for all treatments.
A) The p populations of values of the response variable associated with the treatments have equal variances.
B) The samples of experimental units associated with the treatments are randomly selected.
C) The experimental units associated with the treatments are independent samples.
D) The number of sampled observations must be equal for all p treatments.
E) The distribution of the response variable is normal for all treatments.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
41
What is the degrees of freedom error (within-group variation) of a completely randomized design (one-way) ANOVA test with 4 groups and 15 observations per each group?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
42
A ________ design is an experimental design that compares v treatments by using d blocks, where each block is used exactly once to measure the effect of each treatment.
A) one-way ANOVA
B) two-way ANOVA
C) randomized block
D) balanced complete factorial
A) one-way ANOVA
B) two-way ANOVA
C) randomized block
D) balanced complete factorial

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
43
In performing a one-way ANOVA, ________ measures the variability of the observed values around their respective means by summing the squared differences between each observed value of the response and its corresponding treatment mean.
A) SS Error
B) SS Treatment
C) SS Total
D) SS Treatment/SS Error
A) SS Error
B) SS Treatment
C) SS Total
D) SS Treatment/SS Error

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
44
The ________ units are the entities (objects, people, etc.) to which the treatments are assigned.
A) variable
B) block
C) experimental
D) random
A) variable
B) block
C) experimental
D) random

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
45
In a ________ experimental design, independent random samples of experimental units are assigned to the treatments.
A) one-way ANOVA
B) two-way ANOVA
C) randomized block
D) balanced complete factorial
A) one-way ANOVA
B) two-way ANOVA
C) randomized block
D) balanced complete factorial

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
46
Consider the one-way ANOVA table.
 What is the mean square error?
What is the mean square error?
 What is the mean square error?
What is the mean square error?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
47
Consider the one-way ANOVA table.
 How many groups (treatment levels) are included in the study?
How many groups (treatment levels) are included in the study?
 How many groups (treatment levels) are included in the study?
How many groups (treatment levels) are included in the study?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
48
The variable of interest in an experiment is referred to as the ________ variable.
A) categorical
B) regression
C) response
D) factor
A) categorical
B) regression
C) response
D) factor

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
49
The F test for testing the difference between means is equal to the ratio of Mean Square ________ over Mean Square ________.
A) Treatment; Error
B) Error; Treatment
C) Treatment; Total
D) Error; Total
A) Treatment; Error
B) Error; Treatment
C) Treatment; Total
D) Error; Total

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
50
In one-way ANOVA, the total sum of squares is equal to ________.
A) Treatment SS + Error SS
B) Treatment SS − Error SS
C) Treatment SS × Error SS
D) Treatment SS/Error SS
A) Treatment SS + Error SS
B) Treatment SS − Error SS
C) Treatment SS × Error SS
D) Treatment SS/Error SS

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
51
The dependent variable, the variable of interest in an experiment, is also called the ________ variable.
A) categorical
B) regression
C) response
D) factor
A) categorical
B) regression
C) response
D) factor

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
52
In general, a Tukey simultaneous 100(1 − α) percent confidence interval is ________ the corresponding individual 100(1 − α) percent confidence interval.
A) wider than
B) narrower than
C) no different from
D) two times more than
A) wider than
B) narrower than
C) no different from
D) two times more than

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
53
In a one-way analysis of variance with three treatments, each with five measurements, in which a completely randomized design is used, what are the degrees of freedom for treatments?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
54
Consider the one-way ANOVA table.
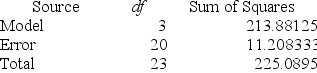 What is the treatment mean square?
What is the treatment mean square?
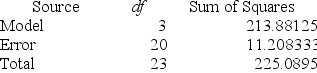 What is the treatment mean square?
What is the treatment mean square?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
55
In performing a one-way ANOVA, the ________ is the between-group variance.
A) MS Error
B) MS Treatment
C) SS Error
D) SS Treatment
A) MS Error
B) MS Treatment
C) SS Error
D) SS Treatment

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
56
Consider the following one-way ANOVA table.
 If there is an equal number of observations in each group, then each group (treatment level) consists of how many observations?
If there is an equal number of observations in each group, then each group (treatment level) consists of how many observations?
 If there is an equal number of observations in each group, then each group (treatment level) consists of how many observations?
If there is an equal number of observations in each group, then each group (treatment level) consists of how many observations?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
57
In a one-way ANOVA table, the ________ the value of MSE, the higher the probability of rejecting the hypothesis that all treatment means are equal.
A) closer to 1
B) closer to 0
C) larger
D) smaller
A) closer to 1
B) closer to 0
C) larger
D) smaller

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
58
In a one-way analysis of variance with three treatments, each with five measurements, in which a completely randomized design is used, what are the degrees of freedom for error?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
59
________ refers to applying a treatment to more than one experimental unit.
A) Randomization
B) Balanced experiment
C) One-way ANOVA
D) Replication
A) Randomization
B) Balanced experiment
C) One-way ANOVA
D) Replication

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
60
If the total sum of squares in a one-way analysis of variance is 25 and the treatment sum of squares is 17, then what is the error sum of squares?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
61
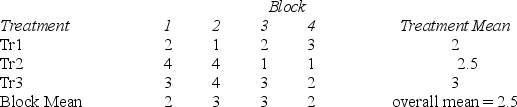 Consider the randomized block design with 4 blocks and 3 treatments given above. What is the treatment mean square?
Consider the randomized block design with 4 blocks and 3 treatments given above. What is the treatment mean square?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
62
What is the degrees of freedom treatment (between-group variation) of a completely randomized design (one-way) ANOVA test with 4 groups and 15 observations per each group?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
63
Consider the following one-way ANOVA table.
 What is the value of the F statistic?
What is the value of the F statistic?
 What is the value of the F statistic?
What is the value of the F statistic?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
64
Looking at four different diets, a researcher randomly assigned 20 equally overweight individuals into each of the four diets. What are the degrees of freedom for the error?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
65
ANOVA table
 Post hoc analysis
Post hoc analysis
Tukey simultaneous comparison t-values
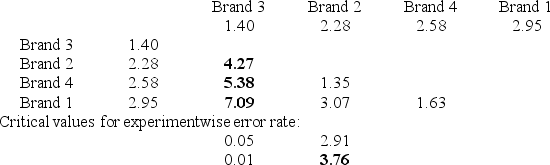 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
Use the information above and determine a Tukey simultaneous 95 percent confidence interval for μ1 − μ2. The mean and sample sizes for brand 1 and brand 2 are as follows: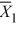 = 2.95,
= 2.95, 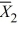 = 2.28, n1 = 4, and n2 = 5.
= 2.28, n1 = 4, and n2 = 5.
 Post hoc analysis
Post hoc analysisTukey simultaneous comparison t-values
 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.Use the information above and determine a Tukey simultaneous 95 percent confidence interval for μ1 − μ2. The mean and sample sizes for brand 1 and brand 2 are as follows:
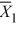 = 2.95,
= 2.95, 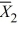 = 2.28, n1 = 4, and n2 = 5.
= 2.28, n1 = 4, and n2 = 5.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
66
 Consider the randomized block design with 4 blocks and 3 treatments given above. What is the block sum of squares?
Consider the randomized block design with 4 blocks and 3 treatments given above. What is the block sum of squares?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
67
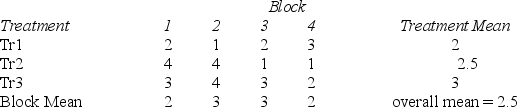 Consider the randomized block design with 4 blocks and 3 treatments given above. What is the total sum of squares?
Consider the randomized block design with 4 blocks and 3 treatments given above. What is the total sum of squares?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
68
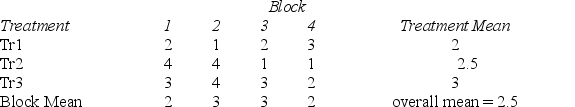 Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for blocks?
Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for blocks?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
69
What is the degrees of freedom error for a randomized block design ANOVA test with 4 treatments and 5 blocks?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
70
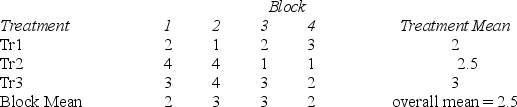 Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for error?
Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for error?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
71
 Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for treatments?
Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for treatments?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
72
 Consider the randomized block design with 4 blocks and 3 treatments given above. What is the treatment sum of squares?
Consider the randomized block design with 4 blocks and 3 treatments given above. What is the treatment sum of squares?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
73
 Consider the randomized block design with 4 blocks and 3 treatments given above. What is the error sum of squares?
Consider the randomized block design with 4 blocks and 3 treatments given above. What is the error sum of squares?
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
74
Find a Tukey simultaneous 95 percent confidence interval for μ1 − μ2, where 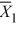 = 33.98,
= 33.98, 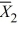 = 36.56, and MSE = .669. There were 15 observations total and 3 treatments. Assume that the number of observations in each treatment is equal.
= 36.56, and MSE = .669. There were 15 observations total and 3 treatments. Assume that the number of observations in each treatment is equal.
 = 33.98,
= 33.98, 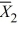 = 36.56, and MSE = .669. There were 15 observations total and 3 treatments. Assume that the number of observations in each treatment is equal.
= 36.56, and MSE = .669. There were 15 observations total and 3 treatments. Assume that the number of observations in each treatment is equal.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
75
ANOVA table
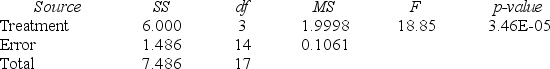 Post hoc analysis
Post hoc analysis
Tukey simultaneous comparison t-values
 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
How many total observations were there in this experiment?
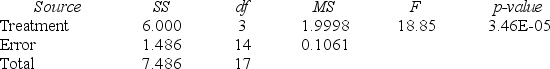 Post hoc analysis
Post hoc analysisTukey simultaneous comparison t-values
 The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.
The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt.How many total observations were there in this experiment?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
76
Looking at four different diets, a researcher randomly assigned 20 equally overweight individuals into each of the four diets. What are the degrees of freedom total?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
77
Find a Tukey simultaneous 95 percent confidence interval for μC − μB, where 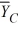 = 51.5,
= 51.5, 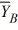 = 55.8, and MSE = 6.125. There were 4 treatments and 24 observations total, and the number of observations were equal in each group.
= 55.8, and MSE = 6.125. There were 4 treatments and 24 observations total, and the number of observations were equal in each group.
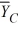 = 51.5,
= 51.5, 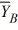 = 55.8, and MSE = 6.125. There were 4 treatments and 24 observations total, and the number of observations were equal in each group.
= 55.8, and MSE = 6.125. There were 4 treatments and 24 observations total, and the number of observations were equal in each group.
Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
78
Looking at four different diets, a researcher randomly assigned 20 equally overweight individuals into each of the four diets. What are the degrees of freedom for the treatments?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
79
Looking at four different diets, a researcher randomly assigned 20 equally overweight individuals into each of the four diets. What are the degrees of freedom for the individual confidence intervals?

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck
80
In a one-way analysis of variance with three treatments, each with five measurements, in which a completely randomized design is used, compute the F statistic where the sum of squares treatment is 17.0493 and the sum of squares error is 8.028.

Unlock Deck
Unlock for access to all 132 flashcards in this deck.
Unlock Deck
k this deck


