Deck 6: Standard Errors
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/10
Play
Full screen (f)
Deck 6: Standard Errors
1
Suppose that in the population of chickens, the average number of feathers per chicken is 1000 with a standard deviation of 200. Suppose that I select a random sample of 64 birds from this population. What is the probability that this sample will have a mean of 950 feathers or fewer?
Standard error:
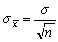
= 200/8 = 25
z = (950-1000)/25 = -2.00
Probability = .0228.
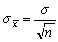
= 200/8 = 25
z = (950-1000)/25 = -2.00
Probability = .0228.
2
Please tell me the definition of "standard error" and then tell me, in your own words, what the standard error of the mean tells you.
A standard error is the average difference between the sample statistic and the population parameter when the samples are of a given size and randomly selected. For example, a standard error of the mean is the average difference between sample means and the population mean when the samples are of a given size and randomly selected.
3
Suppose that I am interested in creating a sampling distribution of the mean. The sample sizes are 40 and the population is undergraduate students. Given this information, tell me how you would create a sampling distribution of the mean for the variable of calories consumed per day.
Select one random sample of 40, calculate the mean for calories consumed per day, then throw that sample back into the population. Now randomly select another sample of the same size, calculate that sample's mean on the same statistic, and throw that sample back into the population. If you keep doing this, you'll eventually create a distribution of sample means, and this is called the sampling distribution of the mean. If randomly selected and the samples are big enough (e.g., 30 or more), the sampling distribution of the mean will form a normal distribution.
4
In your own words, try to explain the meaning and importance of the central limit theorem.

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
5
Take a look at the formulas for calculating the standard error of the mean in Chapter 6. Explain why it makes sense to have the standard deviation in the numerator and the sample size in the denominator. (When I say "makes sense" I mean both mathematically and conceptually. In other words, why does it make sense that as the standard deviation increases the standard error also increases? And why does it make sense that as the sample size increases the standard error decreases?)

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
6
When using standard errors to calculate probabilities of events occurring by chance, how do you know when to use z scores and when to use t values?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
7
Suppose that I know that in the population of bees in California, the average number of flowers pollinated per day is 10 with a standard deviation of 4. I select a random sample of 25 California bees. What is the probability that my sample mean will be 11 or more flowers pollinated?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
8
Suppose that instead of having a sample of 25 in question #7, I had a sample of 100. Tell me how this would affect the results and why it makes sense that the results would be affected in this way.

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
9
Why does it make sense that a larger sample size will produce a smaller standard error of the mean?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
10
Suppose that in the population of American college students the average number of alcoholic drinks consumed per week is 12. What is the probability of randomly selecting a sample (n = 16) with a mean of 10 and a standard deviation of 4? (Hint: Tell me the two probabilities it will be between using a 2-tailed value)

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck


