Deck 19: Ordinary Differential Equations
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/135
Play
Full screen (f)
Deck 19: Ordinary Differential Equations
1
State the order of the following differential equation and whether it is linear or nonlinear:  + cos(x - y) = sin x.
+ cos(x - y) = sin x.
A) 2nd order; linear
B) 2nd order; nonlinear
C) 1st order; nonlinear
D) 1st order; linear
E) None of the above
 + cos(x - y) = sin x.
+ cos(x - y) = sin x.A) 2nd order; linear
B) 2nd order; nonlinear
C) 1st order; nonlinear
D) 1st order; linear
E) None of the above
2nd order; nonlinear
2
State the order of the following differential equation and whether it is linear or nonlinear:  +
+ 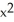 y = sin x
y = sin x
A) 1st order; nonlinear
B) 1st order; linear
C) 2nd order; nonlinear
D) 2nd order; linear
E) None of the above
 +
+ 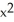 y = sin x
y = sin xA) 1st order; nonlinear
B) 1st order; linear
C) 2nd order; nonlinear
D) 2nd order; linear
E) None of the above
2nd order; linear
3
State the order of the following differential equation and whether it is linear or nonlinear: 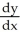 - x
- x  = 0.
= 0.
A) 2nd order; linear
B) 2nd order; nonlinear
C) 1st order; nonlinear
D) 1st order; linear
E) None of the above
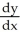 - x
- x  = 0.
= 0.A) 2nd order; linear
B) 2nd order; nonlinear
C) 1st order; nonlinear
D) 1st order; linear
E) None of the above
1st order; nonlinear
4
State the order of the following differential equation and whether it is linear or nonlinear:  = 3x.
= 3x.
A) 1st order; linear
B) 1st order; nonlinear
C) 6th order; linear
D) 6th order; nonlinear
E) None of the above
 = 3x.
= 3x.A) 1st order; linear
B) 1st order; nonlinear
C) 6th order; linear
D) 6th order; nonlinear
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
5
State the order of the following differential equation and whether it is linear or nonlinear: 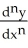 =
= 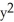 + 2.
+ 2.
A) nth order; linear
B) nth order; nonlinear
C) 1st order; linear
D) 1st order; nonlinear
E) None of the above
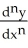 =
= 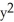 + 2.
+ 2.A) nth order; linear
B) nth order; nonlinear
C) 1st order; linear
D) 1st order; nonlinear
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
6
State the order of the following differential equation and whether it is linear or nonlinear:  + y =
+ y = 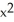
A) 2nd order; linear
B) 2nd order; nonlinear
C) 3/2 order; nonlinear
D) 3/2 order; linear
E) None of the above
 + y =
+ y = 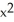
A) 2nd order; linear
B) 2nd order; nonlinear
C) 3/2 order; nonlinear
D) 3/2 order; linear
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
7
Find two values of r such that y = 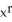 is a solution of
is a solution of 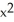
 - 9x
- 9x 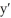 - 24y = 0, x > 0.
- 24y = 0, x > 0.
A) r = 6, 4
B) r = -8, 3
C) r = 12, -2
D) r = -12, 2
E) r = -6, -4
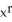 is a solution of
is a solution of 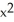
 - 9x
- 9x 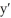 - 24y = 0, x > 0.
- 24y = 0, x > 0.A) r = 6, 4
B) r = -8, 3
C) r = 12, -2
D) r = -12, 2
E) r = -6, -4

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
8
State the order of the following differential equation and whether it is linear or nonlinear:y 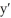 = 1.
= 1.
A) 1st order; linear
B) 1st order; nonlinear
C) 2nd order; nonlinear
D) 2nd order; linear
E) None of the above
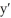 = 1.
= 1.A) 1st order; linear
B) 1st order; nonlinear
C) 2nd order; nonlinear
D) 2nd order; linear
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
9
State the order of the following differential equation and whether it is linear or nonlinear: 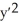 = xy.
= xy.
A) 1st order; nonlinear
B) 1st order; linear
C) 2nd order; nonlinear
D) 2nd order; linear
E) None of the above
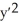 = xy.
= xy.A) 1st order; nonlinear
B) 1st order; linear
C) 2nd order; nonlinear
D) 2nd order; linear
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
10
Specify the interval where y = ln x is a solution of the differential equation x 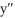 +
+ 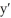 = 0.
= 0.
A) (- , 0)
B) (-1, 1)
C) (- , )
D) (0, )
E) None of the above
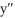 +
+ 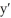 = 0.
= 0.A) (- , 0)
B) (-1, 1)
C) (- , )
D) (0, )
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
11
Solve the initial-value problem 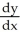 + 4y = 10, y(0) = 200.
+ 4y = 10, y(0) = 200.
A)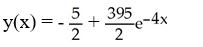
B)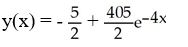
C)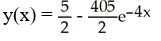
D)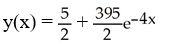
E)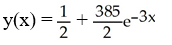
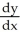 + 4y = 10, y(0) = 200.
+ 4y = 10, y(0) = 200.A)
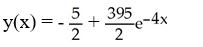
B)
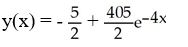
C)
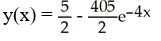
D)
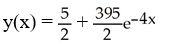
E)
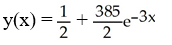

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
12
Solve the differential equation 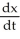 = 6x
= 6x 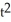 .
.
A) x = C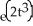
B) x = C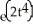
C) x = C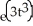
D) x = C
E) x= C
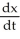 = 6x
= 6x 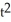 .
.A) x = C
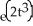
B) x = C
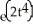
C) x = C
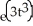
D) x = C

E) x= C


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
13
Find the solution of the initial-value problem  = 3
= 3 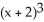 , y(0) = 0.
, y(0) = 0.
A)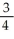
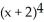 - 12
- 12
B) 9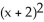 - 36
- 36
C)
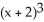 -
- 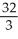
D) -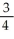
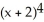 + 12
+ 12
E)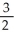
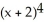 + 12
+ 12
 = 3
= 3 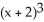 , y(0) = 0.
, y(0) = 0.A)
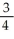
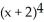 - 12
- 12B) 9
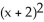 - 36
- 36C)

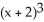 -
- 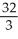
D) -
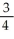
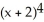 + 12
+ 12E)
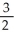
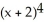 + 12
+ 12
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
14
Determine the solution of the differential equation 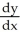 = (1 - 2x)
= (1 - 2x) 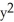 that satisfies the condition
that satisfies the condition 
A) y =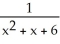
B) y =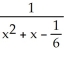
C) y =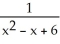
D) y =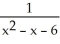
E) y =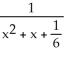
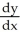 = (1 - 2x)
= (1 - 2x) 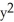 that satisfies the condition
that satisfies the condition 
A) y =
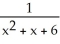
B) y =
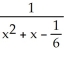
C) y =
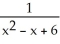
D) y =
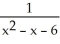
E) y =
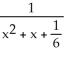

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
15
Find the general solution of the differential equation 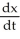 + 3tx = 2t.
+ 3tx = 2t.
A) x = -1 + C
B) x = + C
+ C 
C) x = 2 + C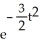
D) x = + C
+ C 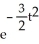
E) x = - + C
+ C 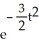
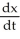 + 3tx = 2t.
+ 3tx = 2t.A) x = -1 + C

B) x =
 + C
+ C 
C) x = 2 + C
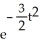
D) x =
 + C
+ C 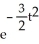
E) x = -
 + C
+ C 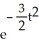

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
16
Find the general solution of the differential equation 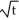
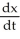 =
= 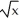
A) x = -2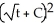
B) x = 2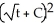
C) x = -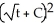
D) x =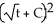
E) x =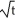 + C
+ C
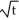
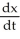 =
= 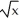
A) x = -2
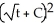
B) x = 2
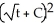
C) x = -
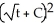
D) x =
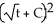
E) x =
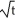 + C
+ C
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
17
Find the solution of the initial-value problem 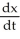 =
= 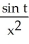 , x(0) = 4.
, x(0) = 4.
A) x =
B) x =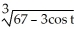
C) x =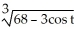
D) x =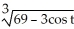
E) x =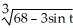
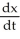 =
= 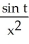 , x(0) = 4.
, x(0) = 4.A) x =

B) x =
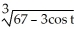
C) x =
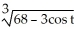
D) x =
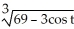
E) x =
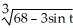

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
18
Find the solution of the initial-value problem  =
= 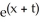 , x(0) = a.
, x(0) = a.
A) x = -ln(1 -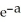 -
- 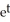 )
)
B) x = ln(1 + -
- 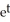 )
)
C) x = -ln(1 +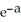 -
- 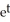 )
)
D) x = ln(1 -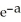 -
-  )
)
E) x = ln(1 -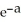 +
+ 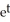 )
)
 =
= 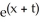 , x(0) = a.
, x(0) = a.A) x = -ln(1 -
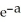 -
- 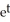 )
)B) x = ln(1 +
 -
- 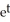 )
)C) x = -ln(1 +
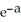 -
- 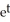 )
)D) x = ln(1 -
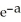 -
-  )
)E) x = ln(1 -
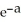 +
+ 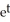 )
)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
19
Solve the initial-value problem 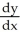 = -
= - 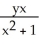 , y(0) = 1.
, y(0) = 1.
A) y(x) =
B) y(x) =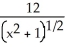
C) y(x) =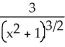
D) y(x) =
E) y(x) =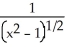
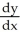 = -
= - 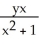 , y(0) = 1.
, y(0) = 1.A) y(x) =

B) y(x) =
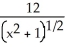
C) y(x) =
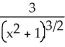
D) y(x) =

E) y(x) =
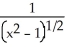

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
20
Find an integrating factor of the form μ(y) for the differential equation  and hence find its general solution.
and hence find its general solution.
 and hence find its general solution.
and hence find its general solution.
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
21
Find the function satisfying 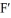 (x) = x
(x) = x 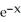 , F(0) = 1.
, F(0) = 1.
A) F(x) = -1 - (x + 1)
(x + 1)
B) F(x) = 1 - (x - 1)
(x - 1)
C) F(x) = 2 -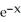 (x + 1)
(x + 1)
D) F(x) = 2 +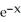 (x - 1)
(x - 1)
E) F(x) = 3 -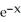 (x + 1)
(x + 1)
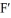 (x) = x
(x) = x 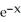 , F(0) = 1.
, F(0) = 1.A) F(x) = -1 -
 (x + 1)
(x + 1)B) F(x) = 1 -
 (x - 1)
(x - 1)C) F(x) = 2 -
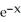 (x + 1)
(x + 1)D) F(x) = 2 +
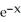 (x - 1)
(x - 1)E) F(x) = 3 -
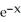 (x + 1)
(x + 1)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
22
Find the solution of the differential equation 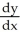 = 12
= 12 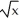 - 2x + 1 that satisfies y(1) = 3.
- 2x + 1 that satisfies y(1) = 3.
A) y =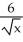 - 2
- 2
B) 8x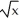 -
- 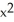 + x + C
+ x + C
C) 8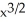 -
- 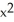 + x
+ x
D) 8x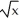 -
-  + x - 5
+ x - 5
E) 8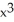
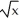 -
- 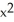 + x + 5
+ x + 5
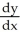 = 12
= 12 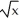 - 2x + 1 that satisfies y(1) = 3.
- 2x + 1 that satisfies y(1) = 3.A) y =
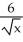 - 2
- 2B) 8x
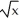 -
- 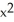 + x + C
+ x + CC) 8
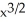 -
- 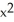 + x
+ xD) 8x
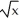 -
-  + x - 5
+ x - 5E) 8
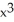
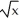 -
- 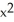 + x + 5
+ x + 5
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
23
Solve the initial-value problem x 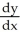 = y (1 + ln(y) - ln(x)), y(1) =
= y (1 + ln(y) - ln(x)), y(1) = 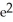 .
.
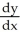 = y (1 + ln(y) - ln(x)), y(1) =
= y (1 + ln(y) - ln(x)), y(1) = 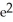 .
.
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
24
Find the general solution of the differential equation 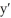 =
= 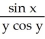 .
.
A) y =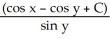
B) y =
C) y =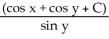
D) y =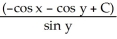
E) y =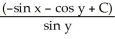
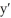 =
= 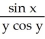 .
.A) y =
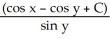
B) y =

C) y =
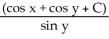
D) y =
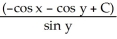
E) y =
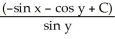

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
25
Find the general solution of the differential equation 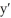 = -9
= -9 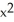
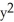 .
.
A)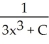
B) -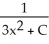
C) -
D) -
E)
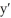 = -9
= -9 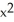
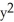 .
.A)
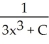
B) -
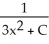
C) -

D) -

E)


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
26
The nonexact differential equation M (t, x)) dt + N(t, x) dx = 0 has an integration factor that depends only upon x, if the quantity 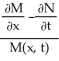 is independent of t.
is independent of t.
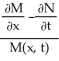 is independent of t.
is independent of t.
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
27
Given the differential equation (7 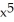 + k
+ k 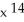
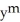 + 19y) dx + (19x + 2
+ 19y) dx + (19x + 2 
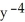 + 23
+ 23  )dy = 0, find the constant real numbers k, m, and n so that the differential equation is exact.
)dy = 0, find the constant real numbers k, m, and n so that the differential equation is exact.
A) k = 10, m = -3, n = -15
B) k = -26, m = 3, n = 13
C) k = -6, m = -5, n = 15
D) k = -10, m = -3, n = 15
E) k = 26, m = 3, n = -13
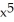 + k
+ k 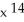
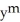 + 19y) dx + (19x + 2
+ 19y) dx + (19x + 2 
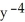 + 23
+ 23  )dy = 0, find the constant real numbers k, m, and n so that the differential equation is exact.
)dy = 0, find the constant real numbers k, m, and n so that the differential equation is exact.A) k = 10, m = -3, n = -15
B) k = -26, m = 3, n = 13
C) k = -6, m = -5, n = 15
D) k = -10, m = -3, n = 15
E) k = 26, m = 3, n = -13

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
28
Find all solutions to 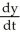 = 1 +
= 1 + 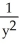 .
.
A) y -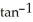 (y) = t + C
(y) = t + C
B) y -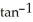 (y) = 2t + C
(y) = 2t + C
C) y +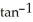 (y) = t + C
(y) = t + C
D) y + (y) = 2t + C
(y) = 2t + C
E) y + (y) = 2t + C
(y) = 2t + C
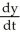 = 1 +
= 1 + 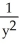 .
.A) y -
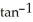 (y) = t + C
(y) = t + CB) y -
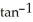 (y) = 2t + C
(y) = 2t + CC) y +
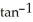 (y) = t + C
(y) = t + CD) y +
 (y) = 2t + C
(y) = 2t + CE) y +
 (y) = 2t + C
(y) = 2t + C
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
29
Solve the following initial-value problem: 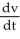 = 9.8 - 0.196v, v(0) = 48.
= 9.8 - 0.196v, v(0) = 48.
A) v = 50 -
B) v = 50 - 2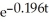
C) v = 50 + 2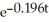
D) v = 100 - 4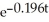
E) v = 50 +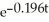
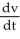 = 9.8 - 0.196v, v(0) = 48.
= 9.8 - 0.196v, v(0) = 48.A) v = 50 -

B) v = 50 - 2
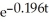
C) v = 50 + 2
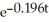
D) v = 100 - 4
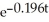
E) v = 50 +
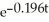

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
30
A motorboat is travelling with a velocity of 40 ft/sec when its motor shuts off at time t = 0. Thereafter, its deceleration due to water resistance is given by 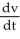 = -k
= -k 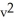 , where k is a positive constant. After 10 seconds, the boat's velocity is measured to be 20 ft/sec.
, where k is a positive constant. After 10 seconds, the boat's velocity is measured to be 20 ft/sec.
(a) What is the value of k?
(b) When will the boat slow to 5 ft/sec?
A) (a)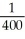 (b) 70 s
(b) 70 s
B) (a)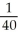 (b) 700 s
(b) 700 s
C) (a)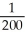 (b) 140 s
(b) 140 s
D) (a)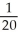 (b) 100 s
(b) 100 s
E) (a)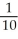 (b) 50 s
(b) 50 s
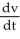 = -k
= -k 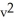 , where k is a positive constant. After 10 seconds, the boat's velocity is measured to be 20 ft/sec.
, where k is a positive constant. After 10 seconds, the boat's velocity is measured to be 20 ft/sec.(a) What is the value of k?
(b) When will the boat slow to 5 ft/sec?
A) (a)
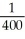 (b) 70 s
(b) 70 sB) (a)
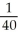 (b) 700 s
(b) 700 sC) (a)
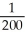 (b) 140 s
(b) 140 sD) (a)
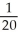 (b) 100 s
(b) 100 sE) (a)
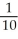 (b) 50 s
(b) 50 s
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
31
The change of dependent variable y =  transforms the equation x
transforms the equation x 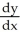 + y = x
+ y = x 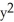 cos(x) into
cos(x) into
A)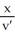 +
+  =
= 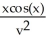
B) ln(v)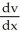 +
+  =
= 
C)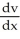 +
+  = cos(x)
= cos(x)
D)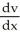 -
-  = cos(x)
= cos(x)
E)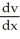 -
-  = -cos(x)
= -cos(x)
 transforms the equation x
transforms the equation x 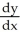 + y = x
+ y = x 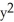 cos(x) into
cos(x) intoA)
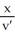 +
+  =
= 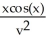
B) ln(v)
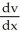 +
+  =
= 
C)
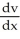 +
+  = cos(x)
= cos(x)D)
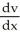 -
-  = cos(x)
= cos(x)E)
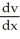 -
-  = -cos(x)
= -cos(x)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
32
Determine a multiplier (integrating factor) of the first order linear differential equation 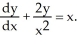
A) (x) = -
B) (x) =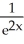
C) (x) =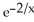
D) (x) = -
E) (x) =
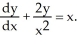
A) (x) = -

B) (x) =
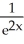
C) (x) =
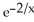
D) (x) = -

E) (x) =


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
33
Find a general solution of the first order linear differential equation 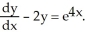
A) y = -
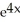 + C
+ C 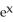
B) y =
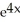 + C
+ C 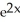
C) y = -
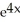 - C
- C 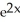
D) y =
 - C
- C 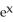
E) y =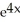 + C
+ C 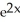
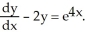
A) y = -

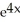 + C
+ C 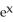
B) y =

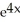 + C
+ C 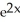
C) y = -

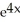 - C
- C 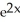
D) y =

 - C
- C 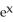
E) y =
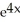 + C
+ C 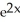

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
34
Find a general solution to the first order linear differential equation 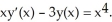
A) y(x) =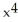 +
+ 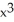 + C
+ C
B) y(x) = + C
+ C 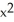
C) y(x) =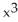 + C
+ C 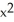
D) y(x) =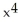 + C
+ C 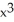
E) y(x) =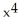 +
+ 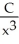
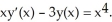
A) y(x) =
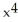 +
+ 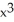 + C
+ CB) y(x) =
 + C
+ C 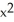
C) y(x) =
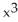 + C
+ C 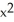
D) y(x) =
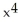 + C
+ C 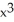
E) y(x) =
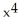 +
+ 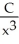

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
35
Find a general solution to the first order linear differential equation 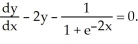
A) y = -
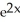 ln(1 +
ln(1 + 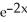 ) + C
) + C 
B) y =
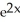 ln(1 +
ln(1 + 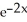 ) + C
) + C 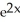
C) y =
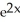 ln(1 -
ln(1 -  ) + C
) + C 
D) y = -
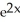 ln(1 -
ln(1 - 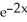 ) + C
) + C 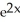
E) y = -
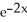 ln(1 +
ln(1 + 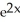 ) + C
) + C 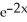
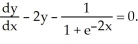
A) y = -

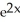 ln(1 +
ln(1 + 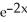 ) + C
) + C 
B) y =

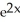 ln(1 +
ln(1 + 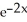 ) + C
) + C 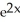
C) y =

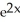 ln(1 -
ln(1 -  ) + C
) + C 
D) y = -

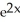 ln(1 -
ln(1 - 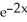 ) + C
) + C 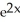
E) y = -

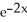 ln(1 +
ln(1 + 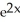 ) + C
) + C 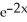

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
36
Find the general solution of the differential equation 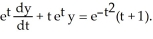
A) y = C +
+ 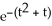
B) y = C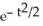 -
- 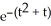
C) y = C -
- 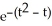
D) y = C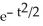 +
+ 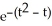
E) y = C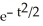 -
- 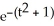
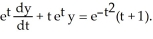
A) y = C
 +
+ 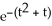
B) y = C
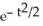 -
- 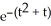
C) y = C
 -
- 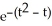
D) y = C
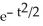 +
+ 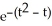
E) y = C
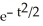 -
- 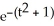

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
37
Solve the initial-value problem 
A) x =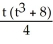
B) x =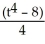
C) x =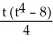
D) x =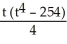
E) x =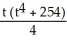

A) x =
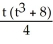
B) x =
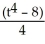
C) x =
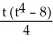
D) x =
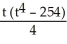
E) x =
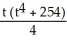

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
38
Solve the differential equation 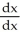 =
=  + 2
+ 2 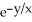 .
.
A) y = x ln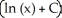
B) y = x ln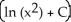
C) y = x ln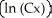
D) y =
E) y =
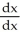 =
=  + 2
+ 2 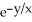 .
.A) y = x ln
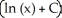
B) y = x ln
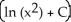
C) y = x ln
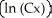
D) y =

E) y =


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
39
Solve the differential equation (3y + 8x 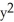 ) dx + (x + 4
) dx + (x + 4  y) dy = 0 by finding an integrating factor depending on x.
y) dy = 0 by finding an integrating factor depending on x.
A) 3xy + 4
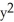 = C
= C
B)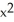 y + 3
y + 3 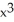
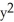 = C
= C
C)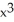 y + 2
y + 2 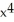
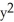 = C
= C
D)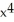 y + 4
y + 4 
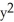 = C
= C
E) y + 2
y + 2 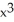
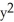 = C
= C
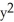 ) dx + (x + 4
) dx + (x + 4  y) dy = 0 by finding an integrating factor depending on x.
y) dy = 0 by finding an integrating factor depending on x.A) 3xy + 4

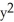 = C
= CB)
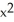 y + 3
y + 3 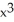
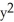 = C
= CC)
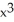 y + 2
y + 2 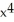
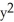 = C
= CD)
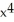 y + 4
y + 4 
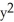 = C
= CE)
 y + 2
y + 2 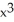
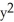 = C
= C
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
40
A tank contains 200 litres of fluid in which 60 grams of salt is dissolved. A brine solution of 2 grams of salt per litre is pumped into the tank at a rate of 2 litres/minute. The well-stirred solution is then pumped out at the same rate.(a) Write the differential equation in terms of Q(t) and t and write the initial condition to model this problem. (Q(t) should represent the amount of salt in the tank at time t.)(b) Solve the equation in part (a).(c) How much salt will be present in the tank after a long period of time?
A) (a)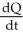 = 2 -
= 2 - 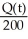 , Q(0) = 60 (b) Q(t) = 200 - 340
, Q(0) = 60 (b) Q(t) = 200 - 340 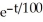 (c) 200 grams
(c) 200 grams
B) (a)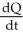 = 4 -
= 4 - 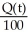 , Q(0) = 60 (b) Q(t) = 400 - 340
, Q(0) = 60 (b) Q(t) = 400 - 340 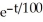 (c) 400 grams
(c) 400 grams
C) (a)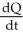 = 400 -
= 400 -  , Q(0) = 60 (b) Q(t) = 40 - 34
, Q(0) = 60 (b) Q(t) = 40 - 34  (c) 40 grams
(c) 40 grams
D) (a)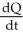 = 400 -
= 400 - 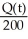 , Q(0) = 60 (b) Q(t) = 4 - 3.4
, Q(0) = 60 (b) Q(t) = 4 - 3.4 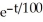 (c) 4 grams
(c) 4 grams
E) (a) = 200 -
= 200 - 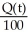 , Q(0) = 60 (b) Q(t) = 20 - 34
, Q(0) = 60 (b) Q(t) = 20 - 34 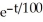 (c) 20 grams
(c) 20 grams
A) (a)
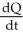 = 2 -
= 2 - 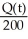 , Q(0) = 60 (b) Q(t) = 200 - 340
, Q(0) = 60 (b) Q(t) = 200 - 340 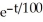 (c) 200 grams
(c) 200 gramsB) (a)
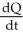 = 4 -
= 4 - 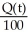 , Q(0) = 60 (b) Q(t) = 400 - 340
, Q(0) = 60 (b) Q(t) = 400 - 340 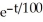 (c) 400 grams
(c) 400 gramsC) (a)
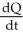 = 400 -
= 400 -  , Q(0) = 60 (b) Q(t) = 40 - 34
, Q(0) = 60 (b) Q(t) = 40 - 34  (c) 40 grams
(c) 40 gramsD) (a)
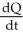 = 400 -
= 400 - 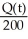 , Q(0) = 60 (b) Q(t) = 4 - 3.4
, Q(0) = 60 (b) Q(t) = 4 - 3.4 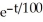 (c) 4 grams
(c) 4 gramsE) (a)
 = 200 -
= 200 - 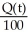 , Q(0) = 60 (b) Q(t) = 20 - 34
, Q(0) = 60 (b) Q(t) = 20 - 34 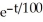 (c) 20 grams
(c) 20 grams
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
41
Solve the following initial-value problem:cos(x) 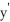 + sin(x)y = 2
+ sin(x)y = 2 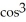 (x) sin(x) - 1, y(
(x) sin(x) - 1, y( 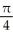 ) = 3
) = 3 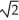 , 0 x
, 0 x 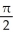 .
.
A) y = -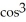 x - sin(x) +
x - sin(x) +  cos(x)
cos(x)
B) y = +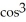 x - sin(x) +
x - sin(x) +  cos(x)
cos(x)
C) y = -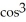 x - sin(x) + 7 cos(x)
x - sin(x) + 7 cos(x)
D) y = +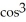 x - sin(x) + 7 cos(x)
x - sin(x) + 7 cos(x)
E) y =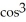 x - sin(x) +
x - sin(x) + 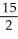 cos(x)
cos(x)
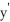 + sin(x)y = 2
+ sin(x)y = 2 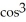 (x) sin(x) - 1, y(
(x) sin(x) - 1, y( 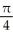 ) = 3
) = 3 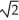 , 0 x
, 0 x 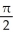 .
.A) y = -
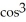 x - sin(x) +
x - sin(x) +  cos(x)
cos(x)B) y = +
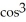 x - sin(x) +
x - sin(x) +  cos(x)
cos(x)C) y = -
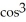 x - sin(x) + 7 cos(x)
x - sin(x) + 7 cos(x)D) y = +
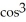 x - sin(x) + 7 cos(x)
x - sin(x) + 7 cos(x)E) y =
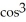 x - sin(x) +
x - sin(x) + 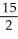 cos(x)
cos(x)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
42
Use Euler's method to determine an approximate value of the solution of the initial-value problem 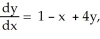
 using step size
using step size 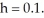
A) 45.6755
B) 43.6755
C) 34.4115
D) 24.5725
E) 64.8978
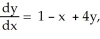
 using step size
using step size 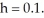
A) 45.6755
B) 43.6755
C) 34.4115
D) 24.5725
E) 64.8978

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
43
Use Euler's method to determine an approximate value of the solution of the initial-value problem 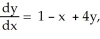
 using step size
using step size 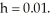 Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
A) 55.5074
B) 57.7279
C) 60.0371
D) 62.4386
E) 64.8978
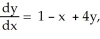
 using step size
using step size 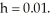 Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.A) 55.5074
B) 57.7279
C) 60.0371
D) 62.4386
E) 64.8978

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
44
Use the Euler method to determine an approximate value of the solution of the initial-value problem 
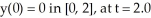 using step size
using step size 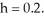
A) 110.4464
B) 120.6730
C) 115.2341
D) 112.3558
E) 145.2351

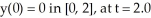 using step size
using step size 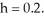
A) 110.4464
B) 120.6730
C) 115.2341
D) 112.3558
E) 145.2351

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
45
Use Euler's method with step size h = 0.1 to approximate the solution of the initial-value problem 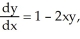
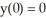 on the interval
on the interval 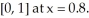
A) 0.5387
B) 0.5633
C) 0.5732
D) 0.5700
E) 0.5123
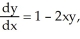
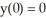 on the interval
on the interval 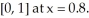
A) 0.5387
B) 0.5633
C) 0.5732
D) 0.5700
E) 0.5123

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
46
Use the improved Euler method to determine an approximate value of the solution of the initial-value problem 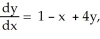
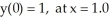 using step size
using step size 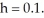
A) 27.3480
B) 40.9407
C) 59.9382
D) 88.7036
E) 64.8978
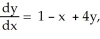
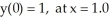 using step size
using step size 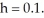
A) 27.3480
B) 40.9407
C) 59.9382
D) 88.7036
E) 64.8978

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
47
Use the improved Euler method to determine an approximate value of the solution of the initial-value problem 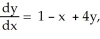
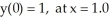 using step size
using step size 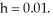 Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
A) 59.8473
B) 62.2892
C) 64.8307
D) 67.2433
E) 64.8978
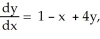
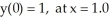 using step size
using step size 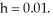 Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.A) 59.8473
B) 62.2892
C) 64.8307
D) 67.2433
E) 64.8978

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
48
Use the Euler method to determine an approximate value of the solution of the initial-value problem 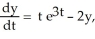
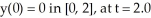 using step size
using step size 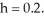
A) 633.8378
B) 316.6792
C) 156.6864
D) 76.6012
E) 164.3621
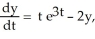
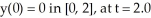 using step size
using step size 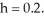
A) 633.8378
B) 316.6792
C) 156.6864
D) 76.6012
E) 164.3621

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
49
Use Euler's method with step size h = 0.1 to approximate the solution of the initial-value problem 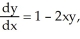
 on the interval
on the interval 
A) 0.4662
B) 0.5215
C) 0.5272
D) 0.4978
E) 0.9745
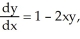
 on the interval
on the interval 
A) 0.4662
B) 0.5215
C) 0.5272
D) 0.4978
E) 0.9745

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
50
Use the Runge-Kutta method to determine an approximate value of the solution of the initial-value problem 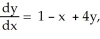
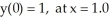 using step size
using step size 
A) 144.3001
B) 96.4752
C) 64.8581
D) 43.4739
E) 72.8576
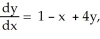
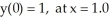 using step size
using step size 
A) 144.3001
B) 96.4752
C) 64.8581
D) 43.4739
E) 72.8576

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
51
Use the Runge-Kutta method to determine an approximate value of the solution of the initial-value problem 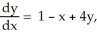
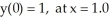 using step size
using step size  Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
A) 59.8473
B) 67.5463
C) 64.8978
D) 70.3027
E) 71.6654
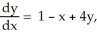
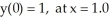 using step size
using step size  Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.
Use formula replication in a computer spreadsheet to carry out the calculations as you now need 100 iterations of the formula.A) 59.8473
B) 67.5463
C) 64.8978
D) 70.3027
E) 71.6654

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
52
Use the Euler method to determine an approximate value of the solution of the initial-value problem 
 using step size
using step size 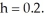
A) 154.9004
B) 151.8943
C) 146.0963
D) 121.3222
E) 138.5736

 using step size
using step size 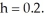
A) 154.9004
B) 151.8943
C) 146.0963
D) 121.3222
E) 138.5736

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
53
Use the Runge-Kutta method with step size h = 0.1 to approximate the solution of the initial-value problem 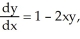
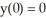 on the interval
on the interval 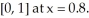
A) 0.5321
B) 0.5380
C) 0.5407
D) 0.5487
E) 0.5582
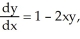
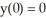 on the interval
on the interval 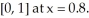
A) 0.5321
B) 0.5380
C) 0.5407
D) 0.5487
E) 0.5582

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
54
Solve the integral equation y(x) = 1 + 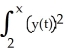 dt by reinterpreting it as an initial-value problem.
dt by reinterpreting it as an initial-value problem.
A) y =
B) y =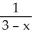
C) y =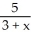
D) y =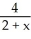
E) y =
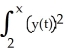 dt by reinterpreting it as an initial-value problem.
dt by reinterpreting it as an initial-value problem.A) y =

B) y =
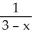
C) y =
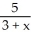
D) y =
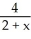
E) y =


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
55
Solve the initial-value problem 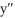 + 2 = 0,
+ 2 = 0, 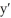 (2) = 3, y(-1) = -4.
(2) = 3, y(-1) = -4.
A) y = -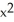 + 7x + 12
+ 7x + 12
B) y = -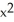 + 7x - 12
+ 7x - 12
C) y = -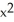 + x - 2
+ x - 2
D) y = - + 7x + 4
+ 7x + 4
E) y = -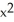 - x - 4
- x - 4
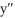 + 2 = 0,
+ 2 = 0, 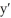 (2) = 3, y(-1) = -4.
(2) = 3, y(-1) = -4.A) y = -
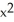 + 7x + 12
+ 7x + 12B) y = -
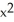 + 7x - 12
+ 7x - 12C) y = -
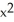 + x - 2
+ x - 2D) y = -
 + 7x + 4
+ 7x + 4E) y = -
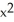 - x - 4
- x - 4
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
56
Solve the initial-value problem x  + 3
+ 3 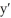 = 0,
= 0, 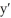 (1) = 2, y(1) = 3.
(1) = 2, y(1) = 3.
A) y = 4 -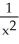
B) y = 2 +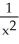
C) y = 5 -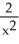
D) y = 1 +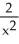
E) y = 4 +
 + 3
+ 3 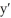 = 0,
= 0, 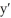 (1) = 2, y(1) = 3.
(1) = 2, y(1) = 3.A) y = 4 -
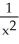
B) y = 2 +
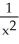
C) y = 5 -
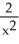
D) y = 1 +
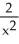
E) y = 4 +


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
57
Solve the initial-value problem  = 1 +
= 1 + 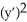 ,
, 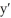 (0) = 1, y(0) = 0.
(0) = 1, y(0) = 0.
A) y = -ln(cos(x) - sin(x))
B) y = ln(cos(x) - sin(x))
C) y = -ln(sin(x) - cos(x))
D) y = ln(sin(x) - cos(x))
E) None of the above
 = 1 +
= 1 + 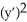 ,
, 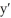 (0) = 1, y(0) = 0.
(0) = 1, y(0) = 0.A) y = -ln(cos(x) - sin(x))
B) y = ln(cos(x) - sin(x))
C) y = -ln(sin(x) - cos(x))
D) y = ln(sin(x) - cos(x))
E) None of the above

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
58
Solve the initial-value problem 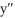 - 2y
- 2y 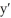 = 0,
= 0, 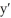 (3) = 1, y(3) = 1.
(3) = 1, y(3) = 1.
A) y =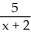
B) y =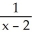
C) y =
D) y =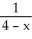
E) y =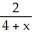
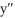 - 2y
- 2y 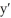 = 0,
= 0, 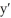 (3) = 1, y(3) = 1.
(3) = 1, y(3) = 1.A) y =
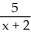
B) y =
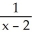
C) y =

D) y =
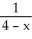
E) y =
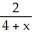

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
59
Given that y = x is a solution of the differential equation 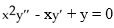 , find the solution of the equation that satisfies y(1) = 3 and
, find the solution of the equation that satisfies y(1) = 3 and 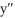 (1) = 5.
(1) = 5.
A) y = 3x + 2ln(x)
B) y = x +
C) y = 3x + 2xln(x)
D) y = x +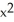
E) y = 5x -2
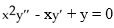 , find the solution of the equation that satisfies y(1) = 3 and
, find the solution of the equation that satisfies y(1) = 3 and 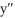 (1) = 5.
(1) = 5.A) y = 3x + 2ln(x)
B) y = x +

C) y = 3x + 2xln(x)
D) y = x +
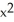
E) y = 5x -2

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
60
Verify that y = 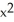 is a solution of the equation
is a solution of the equation 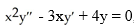 , and find the general solution of this equation
, and find the general solution of this equation
A) y = A + B
+ B 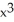
B) y = A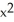 + B
+ B 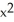 ln x
ln x
C) y = A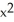 + Bx
+ Bx
D) y = A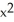 + B
+ B
E) y = A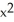 + Bx
+ Bx 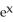
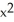 is a solution of the equation
is a solution of the equation 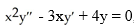 , and find the general solution of this equation
, and find the general solution of this equationA) y = A
 + B
+ B 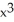
B) y = A
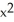 + B
+ B 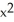 ln x
ln xC) y = A
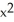 + Bx
+ BxD) y = A
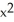 + B
+ BE) y = A
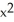 + Bx
+ Bx 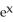

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
61
Find the general solution of the differential equation 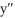 -
- 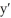 + 12y = 0.
+ 12y = 0.
A) y = A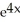 + B
+ B 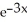
B) y = A + B
+ B 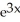
C) y = A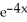 + B
+ B 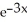
D) y = A + B
+ B 
E) y = A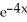 + Bx
+ Bx 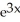
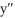 -
- 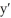 + 12y = 0.
+ 12y = 0.A) y = A
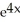 + B
+ B 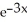
B) y = A
 + B
+ B 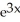
C) y = A
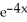 + B
+ B 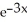
D) y = A
 + B
+ B 
E) y = A
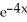 + Bx
+ Bx 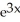

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
62
Find the general solution of the differential equation 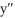 + 6
+ 6 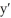 + 9y = 0.
+ 9y = 0.
A) y = A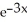 + B
+ B 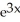
B) y = (A + Bx)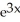
C) y = (A + Bx)
D) y = Ax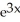 + Bx
+ Bx 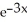
E) y = A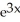 + Bx
+ Bx 
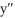 + 6
+ 6 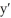 + 9y = 0.
+ 9y = 0.A) y = A
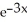 + B
+ B 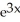
B) y = (A + Bx)
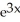
C) y = (A + Bx)

D) y = Ax
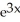 + Bx
+ Bx 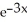
E) y = A
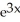 + Bx
+ Bx 

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
63
Find the general solution of the differential equation ![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_777e_ce77_a0f8_1fa3da00149f_TB9661_11.jpg) +
+ ![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_777e_ce78_a0f8_918af9b055d7_TB9661_11.jpg) + 17y = 0.
+ 17y = 0.
A) y = [Acos(4x) + Bsin(4x)]![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d99_a0f8_8191040b8799_TB9661_11.jpg)
B) y = [Acos(4x) - Bsin(4x)]![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9a_a0f8_bf5c03ed2c47_TB9661_11.jpg)
C) y = [Acos(4x) + Bsin(4x)]![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9b_a0f8_01cbf450a429_TB9661_11.jpg)
D) y = [Acos(4x) - Bsin(4x)]![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9c_a0f8_1fb34a107207_TB9661_11.jpg)
E) y = [Acos(x) + Bsin(x)]![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9d_a0f8_b353d69e4365_TB9661_11.jpg)
![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_777e_ce77_a0f8_1fa3da00149f_TB9661_11.jpg) +
+ ![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_777e_ce78_a0f8_918af9b055d7_TB9661_11.jpg) + 17y = 0.
+ 17y = 0.A) y = [Acos(4x) + Bsin(4x)]
![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d99_a0f8_8191040b8799_TB9661_11.jpg)
B) y = [Acos(4x) - Bsin(4x)]
![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9a_a0f8_bf5c03ed2c47_TB9661_11.jpg)
C) y = [Acos(4x) + Bsin(4x)]
![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9b_a0f8_01cbf450a429_TB9661_11.jpg)
D) y = [Acos(4x) - Bsin(4x)]
![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9c_a0f8_1fb34a107207_TB9661_11.jpg)
E) y = [Acos(x) + Bsin(x)]
![<strong>Find the general solution of the differential equation + + 17y = 0.</strong> A) y = [Acos(4x) + Bsin(4x)] B) y = [Acos(4x) - Bsin(4x)] C) y = [Acos(4x) + Bsin(4x)] D) y = [Acos(4x) - Bsin(4x)] E) y = [Acos(x) + Bsin(x)]](https://storage.examlex.com/TB9661/11ee77e1_7781_8d9d_a0f8_b353d69e4365_TB9661_11.jpg)

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
64
Find the general solution of the differential equation 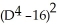 y= 0, where D stands for the differential operator
y= 0, where D stands for the differential operator  .
.
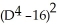 y= 0, where D stands for the differential operator
y= 0, where D stands for the differential operator  .
.
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
65
Find the general solution of the differential equation 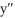 - 10
- 10 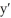 + 74y = 0.
+ 74y = 0.
A) y =
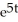 cos(7t) +
cos(7t) + 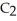
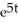 sin(7t)
sin(7t)
B) y =
 cos(7t) +
cos(7t) + 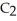
 sin(7t)
sin(7t)
C) y =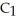
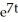 cos(5t) +
cos(5t) + 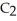
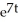 sin(5t)
sin(5t)
D) y =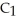
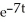 cos(5t) +
cos(5t) + 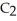
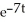 sin(5t)
sin(5t)
E) y =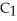
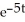 cos(7t) +
cos(7t) + 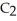
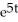 sin(7t)
sin(7t)
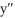 - 10
- 10 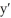 + 74y = 0.
+ 74y = 0.A) y =

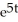 cos(7t) +
cos(7t) + 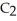
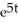 sin(7t)
sin(7t)B) y =

 cos(7t) +
cos(7t) + 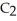
 sin(7t)
sin(7t)C) y =
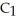
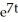 cos(5t) +
cos(5t) + 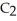
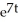 sin(5t)
sin(5t)D) y =
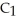
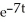 cos(5t) +
cos(5t) + 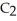
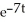 sin(5t)
sin(5t)E) y =
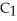
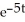 cos(7t) +
cos(7t) + 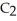
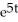 sin(7t)
sin(7t)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
66
Find the general solution of the differential equation  + 13
+ 13 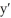 + 3y = 0.
+ 3y = 0.
A) y =
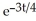 +
+ 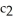
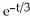
B) y =
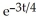 -
- 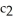
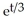
C) y =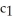
 +
+ 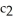
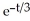
D) y =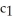
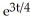 +
+ 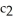
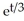
E) y =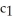
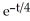 +
+ 

 + 13
+ 13 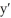 + 3y = 0.
+ 3y = 0.A) y =

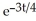 +
+ 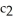
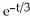
B) y =

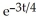 -
- 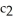
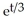
C) y =
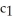
 +
+ 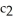
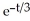
D) y =
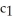
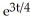 +
+ 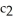
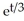
E) y =
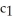
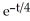 +
+ 


Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
67
Solve the initial-value problem 12 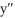 -
- 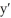 -6y = 0; y(0) = 2,
-6y = 0; y(0) = 2, 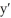 (0) = 10.
(0) = 10.
A) y(t) = 6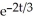 - 8
- 8 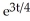
B) y(t) = 4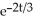 - 2
- 2 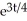
C) y(t) = -6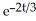 + 8
+ 8 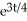
D) y(t) = -4 + 6
+ 6 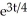
E) y(t) = -6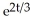 + 8
+ 8 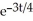
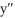 -
- 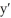 -6y = 0; y(0) = 2,
-6y = 0; y(0) = 2, 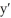 (0) = 10.
(0) = 10.A) y(t) = 6
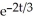 - 8
- 8 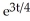
B) y(t) = 4
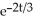 - 2
- 2 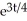
C) y(t) = -6
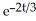 + 8
+ 8 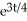
D) y(t) = -4
 + 6
+ 6 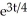
E) y(t) = -6
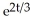 + 8
+ 8 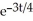

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
68
Solve the initial-value problem 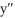 + 2
+ 2 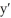 + 2y = 0; y(0) = 4,
+ 2y = 0; y(0) = 4, 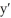 (0) = -2.
(0) = -2.
A) y(t) = 4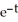 cos t - 6
cos t - 6 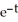 sin t
sin t
B) y(t) = 4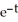 cos t - 2
cos t - 2  sin t
sin t
C) y(t) = 4 cos t + 2
cos t + 2 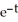 sin t
sin t
D) y(t) = 4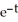 cos t + 6
cos t + 6 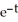 sin t
sin t
E) y(t) = 4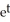 cos t + 2
cos t + 2  sin t
sin t
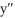 + 2
+ 2 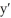 + 2y = 0; y(0) = 4,
+ 2y = 0; y(0) = 4, 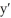 (0) = -2.
(0) = -2.A) y(t) = 4
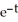 cos t - 6
cos t - 6 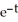 sin t
sin tB) y(t) = 4
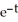 cos t - 2
cos t - 2  sin t
sin tC) y(t) = 4
 cos t + 2
cos t + 2 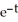 sin t
sin tD) y(t) = 4
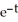 cos t + 6
cos t + 6 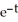 sin t
sin tE) y(t) = 4
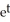 cos t + 2
cos t + 2  sin t
sin t
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
69
Solve the initial-value problem  + 8
+ 8 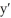 + 16y = 0; y(0) = 3,
+ 16y = 0; y(0) = 3, 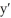 (0) = 1.
(0) = 1.
A) y = (13t + 3)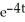
B) y = (13t - 3)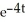
C) y = (13t + 3)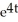
D) y = (7t + 1)
E) y = (7t - 1)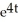
 + 8
+ 8 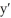 + 16y = 0; y(0) = 3,
+ 16y = 0; y(0) = 3, 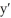 (0) = 1.
(0) = 1.A) y = (13t + 3)
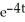
B) y = (13t - 3)
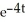
C) y = (13t + 3)
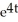
D) y = (7t + 1)

E) y = (7t - 1)
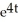

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
70
Solve the initial-value problem 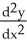 + 4
+ 4 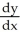 = 0, y(1)= 1,
= 0, y(1)= 1, 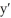 (1) = 2.
(1) = 2.
A) y = -
- 
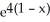
B) y = -
- 
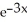
C) y = -
- 
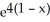
D) y = -
- 
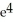
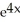
E) y = -
- 
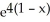
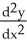 + 4
+ 4 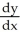 = 0, y(1)= 1,
= 0, y(1)= 1, 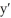 (1) = 2.
(1) = 2.A) y =
 -
- 
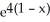
B) y =
 -
- 
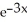
C) y =
 -
- 
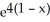
D) y =
 -
- 
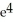
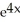
E) y =
 -
- 
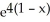

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
71
Find a homogeneous linear second-order differential equation with constant coefficient having general solution y = 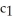
 +
+  t
t 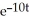 .
.
A)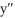 + 20
+ 20 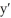 - 100y = 0
- 100y = 0
B)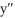 + 20
+ 20 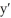 + 100y = 0
+ 100y = 0
C) - 20
- 20 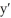 + 100y = 0
+ 100y = 0
D)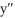 - 20
- 20 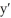 - 100y = 0
- 100y = 0
E) + 10
+ 10 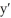 + 100y = 0
+ 100y = 0
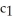
 +
+  t
t 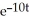 .
.A)
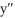 + 20
+ 20 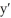 - 100y = 0
- 100y = 0B)
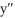 + 20
+ 20 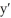 + 100y = 0
+ 100y = 0C)
 - 20
- 20 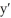 + 100y = 0
+ 100y = 0D)
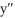 - 20
- 20 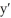 - 100y = 0
- 100y = 0E)
 + 10
+ 10 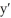 + 100y = 0
+ 100y = 0
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
72
Find a homogeneous linear second-order differential equation with constant coefficient having general solution y = . 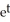
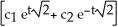
A)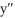 - 2
- 2 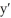 - y = 0
- y = 0
B)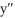 + 2
+ 2 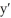 - y = 0
- y = 0
C)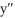 - 2
- 2 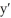 + y = 0
+ y = 0
D)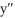 + 2
+ 2  + 2y = 0
+ 2y = 0
E)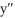 + 2
+ 2  + y = 0
+ y = 0
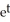
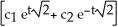
A)
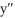 - 2
- 2 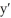 - y = 0
- y = 0B)
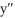 + 2
+ 2 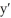 - y = 0
- y = 0C)
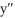 - 2
- 2 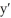 + y = 0
+ y = 0D)
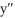 + 2
+ 2  + 2y = 0
+ 2y = 0E)
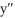 + 2
+ 2  + y = 0
+ y = 0
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
73
Find the general solution of the differential equation 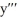 - 2y = 0.
- 2y = 0.
A) y = A expt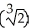 + exp
+ exp 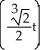
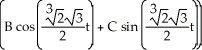
B) y = A expt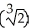 + exp
+ exp 

C) y = A exp(t) + exp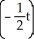

D) y = A expt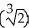 + exp
+ exp 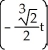
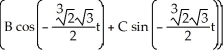
E) y = A expt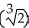 + exp
+ exp 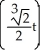
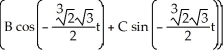
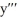 - 2y = 0.
- 2y = 0.A) y = A expt
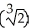 + exp
+ exp 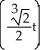
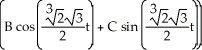
B) y = A expt
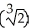 + exp
+ exp 

C) y = A exp(t) + exp
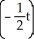

D) y = A expt
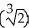 + exp
+ exp 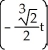
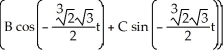
E) y = A expt
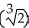 + exp
+ exp 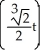
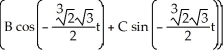

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
74
Find the general solution of the differential equation 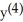 - 16y = 0.
- 16y = 0.
A) y = A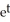 + B
+ B 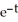 + C cos(2t) + D sin(2t)
+ C cos(2t) + D sin(2t)
B) y = A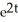 + B
+ B 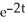 + C cos(2t) + D sin(2t)
+ C cos(2t) + D sin(2t)
C) y = A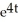 + B
+ B 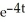 + C cos(4t) + D sin(4t)
+ C cos(4t) + D sin(4t)
D) y = A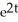 + B
+ B 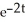 + C
+ C 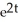 cos(2t) + D
cos(2t) + D 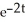 sin(2t)
sin(2t)
E) y = A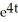 + B
+ B 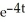 + C
+ C 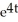 cos(4t) + D
cos(4t) + D 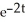 sin(4t)
sin(4t)
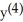 - 16y = 0.
- 16y = 0.A) y = A
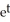 + B
+ B 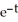 + C cos(2t) + D sin(2t)
+ C cos(2t) + D sin(2t)B) y = A
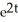 + B
+ B 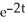 + C cos(2t) + D sin(2t)
+ C cos(2t) + D sin(2t)C) y = A
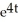 + B
+ B 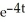 + C cos(4t) + D sin(4t)
+ C cos(4t) + D sin(4t)D) y = A
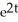 + B
+ B 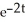 + C
+ C 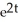 cos(2t) + D
cos(2t) + D 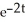 sin(2t)
sin(2t)E) y = A
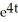 + B
+ B 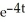 + C
+ C 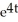 cos(4t) + D
cos(4t) + D 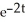 sin(4t)
sin(4t)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
75
Find the general solution of the differential equation 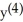 + 4
+ 4 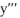 + 6
+ 6 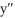 +4
+4 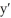 + y = 0.
+ y = 0.
A) y =
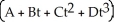
B) y = A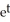 + B
+ B  + Ct
+ Ct 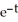 + Dt
+ Dt 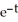
C) y = A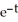 + B
+ B 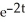 + C
+ C 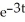 + D
+ D 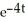
D) y =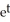
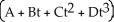
E) y = A + B
+ B 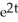 + C
+ C 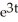 + D
+ D 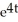
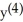 + 4
+ 4 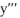 + 6
+ 6 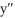 +4
+4 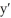 + y = 0.
+ y = 0.A) y =

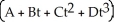
B) y = A
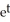 + B
+ B  + Ct
+ Ct 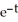 + Dt
+ Dt 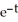
C) y = A
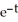 + B
+ B 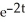 + C
+ C 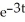 + D
+ D 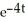
D) y =
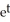
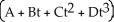
E) y = A
 + B
+ B 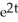 + C
+ C 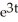 + D
+ D 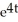

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
76
Find the general solution of the differential equation 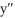 + 4y = 0.
+ 4y = 0.
A) y = cos(2x) + sin(2x)
B) y = cos(-2x) + sin(-2x)
C) y =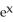
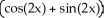
D) y =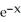
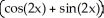
E) y =

 + 4y = 0.
+ 4y = 0.A) y = cos(2x) + sin(2x)
B) y = cos(-2x) + sin(-2x)
C) y =
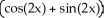
D) y =
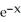
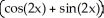
E) y =



Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
77
If the auxiliary equation of a certain linear, homogeneous, 7th order DE with constant coefficients factors to 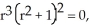 find the general solution of the DE.
find the general solution of the DE.
A) y = A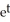 + Bt + C
+ Bt + C 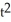 + (D + Et) cos(t) + (F + Gt) sin(t)
+ (D + Et) cos(t) + (F + Gt) sin(t)
B) y =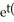 (A + Bt + C
(A + Bt + C 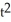 ) + (D + Et) cos(t) + (F + Gt) sin(t)
) + (D + Et) cos(t) + (F + Gt) sin(t)
C) y = A + Bt + C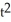 + (D + Et) cos(t/2) + (F + Gt) sin(t/2)
+ (D + Et) cos(t/2) + (F + Gt) sin(t/2)
D) y = A + Bt + C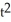 + (D + Et) cos(t) + (F + Gt) sin(t)
+ (D + Et) cos(t) + (F + Gt) sin(t)
E) y = A + Bt
+ Bt 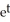 + C
+ C 
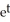 + (D + Et) cos(t) + (F + Gt) sin(t)
+ (D + Et) cos(t) + (F + Gt) sin(t)
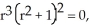 find the general solution of the DE.
find the general solution of the DE.A) y = A
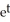 + Bt + C
+ Bt + C 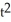 + (D + Et) cos(t) + (F + Gt) sin(t)
+ (D + Et) cos(t) + (F + Gt) sin(t)B) y =
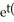 (A + Bt + C
(A + Bt + C 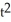 ) + (D + Et) cos(t) + (F + Gt) sin(t)
) + (D + Et) cos(t) + (F + Gt) sin(t)C) y = A + Bt + C
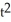 + (D + Et) cos(t/2) + (F + Gt) sin(t/2)
+ (D + Et) cos(t/2) + (F + Gt) sin(t/2)D) y = A + Bt + C
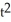 + (D + Et) cos(t) + (F + Gt) sin(t)
+ (D + Et) cos(t) + (F + Gt) sin(t)E) y = A
 + Bt
+ Bt 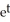 + C
+ C 
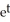 + (D + Et) cos(t) + (F + Gt) sin(t)
+ (D + Et) cos(t) + (F + Gt) sin(t)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
78
Find the general solution of the differential equation 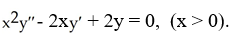
A) y = Ax + B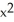
B) y = x(A cos(ln x) + B sin(ln x))
C) A + Bx
D) A + B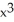
E) y = x(A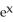 + B x
+ B x 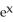 )
)
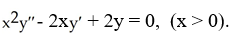
A) y = Ax + B
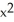
B) y = x(A cos(ln x) + B sin(ln x))
C) A + Bx
D) A + B
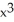
E) y = x(A
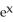 + B x
+ B x 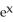 )
)
Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
79
Find the general solution of the differential equation 
A) y = Ax cos(ln x) + Bx sin(ln x)
B) y = A cos(2ln x) + B sin(2ln x)
C) y = A cos(ln x) + B sin(ln x)
D) y = Ax + Bx ln x
E) y = Ax cos x + Bx sin x

A) y = Ax cos(ln x) + Bx sin(ln x)
B) y = A cos(2ln x) + B sin(2ln x)
C) y = A cos(ln x) + B sin(ln x)
D) y = Ax + Bx ln x
E) y = Ax cos x + Bx sin x

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck
80
Solve the initial-value problem 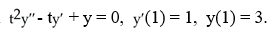
A) y = 3t - 2t ln t
B) y = 4t - 3t ln t
C) y = 3t + 2t ln t
D) y = 3t + 2 ln t
E) y = 3t - t ln t
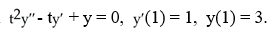
A) y = 3t - 2t ln t
B) y = 4t - 3t ln t
C) y = 3t + 2t ln t
D) y = 3t + 2 ln t
E) y = 3t - t ln t

Unlock Deck
Unlock for access to all 135 flashcards in this deck.
Unlock Deck
k this deck


