Deck 12: Risk Analysis
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/17
Play
Full screen (f)
Deck 12: Risk Analysis
1
Investment A has an expected value of 5 and a standard deviation of 2. Investment B has an expected value of 10 and a standard deviation of 5. Using the coefficient of variation approach to comparing these two investments,
A) Investment A would be selected because it has the larger coefficient of variation.
B) Investment B would be selected because it has the larger coefficient of variation.
C) Investment A would be selected because it has the smaller coefficient of variation.
D) Investment B would be selected because it has the smaller coefficient of variation.
A) Investment A would be selected because it has the larger coefficient of variation.
B) Investment B would be selected because it has the larger coefficient of variation.
C) Investment A would be selected because it has the smaller coefficient of variation.
D) Investment B would be selected because it has the smaller coefficient of variation.
Investment A would be selected because it has the smaller coefficient of variation.
2
When there is only one possible outcome to a decision, risk or uncertainty is present.
False
3
Risk refers to a situation in which the probability of each possible outcome to a decision is unknown or meaningless.
False
4
The Z value for a particular outcome is equal to the difference between the outcome and the expected outcome measured in terms of standard deviations.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
5
Z values cannot be negative or zero.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
6
A certainty equivalent coefficient of one is used if the decision maker is risk neutral.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
7
Branches coming out of circles on decision trees show alternative courses of action that can be selected by decision makers.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
8
Test marketing is an example of simulation.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
9
The maximin criterion is a method of dealing with uncertainty.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
10
A firm is considering two business projects. Project A will return a loss of $45 if conditions are poor, a profit of $35 if conditions are good, and a profit of $155 if conditions are excellent. Project B will return a loss of $100 if conditions are poor, a profit of $60 if conditions are good, and a profit of $300 if conditions are excellent. The probability distribution of conditions follows:
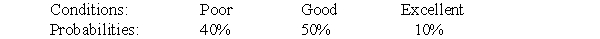 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(ii) Calculate the standard deviation of each project and identify the project that has the higher level of risk.
(iii) Calculate the coefficient of variation for each project and identify the preferred project according to this criterion.
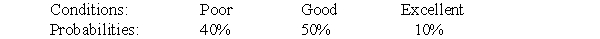 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.(ii) Calculate the standard deviation of each project and identify the project that has the higher level of risk.
(iii) Calculate the coefficient of variation for each project and identify the preferred project according to this criterion.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
11
A firm is considering two business projects. Project A will return a loss of $5 if conditions are poor, a profit of $35 if conditions are good, and a profit of $95 if conditions are excellent. Project B will return a loss of $15 if conditions are poor, a profit of $45 if conditions are good, and a profit of $135 if conditions are excellent. The probability distribution of conditions follows:
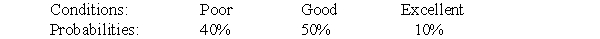 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(ii) Calculate the standard deviation of each project and identify the project that has the higher level of risk.
(iii) Calculate the coefficient of variation for each project and identify the preferred project according to this criterion.
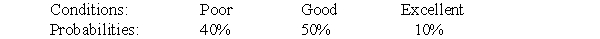 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.(ii) Calculate the standard deviation of each project and identify the project that has the higher level of risk.
(iii) Calculate the coefficient of variation for each project and identify the preferred project according to this criterion.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
12
A firm is considering two business projects. Project A will return a profit of zero if conditions are poor, a profit of $16 if conditions are good, and a profit of $49 if conditions are excellent. Project B will return a profit of $4 if conditions are poor, a profit of $9 if conditions are good, and a profit of $49 if conditions are excellent. The probability distribution of conditions follows:
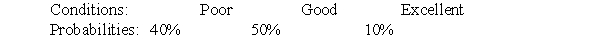 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(ii) Assume that the firm has determined that its utility function for profit is equal to the square root of profit. Calculate the expected utility of each project and identify the preferred project according to this criterion.
(iii) Is the firm risk averse, risk neutral, or risk seeking? How can you tell?
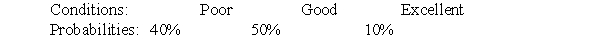 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.(ii) Assume that the firm has determined that its utility function for profit is equal to the square root of profit. Calculate the expected utility of each project and identify the preferred project according to this criterion.
(iii) Is the firm risk averse, risk neutral, or risk seeking? How can you tell?

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
13
A firm is considering two business projects. Project A will return a profit of zero if conditions are poor, a profit of $4 if conditions are good, and a profit of $8 if conditions are excellent. Project B will return a profit of $2 if conditions are poor, a profit of $3 if conditions are good, and a profit of $4 if conditions are excellent. The probability distribution of conditions follows:
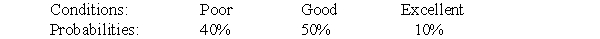 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(ii) Assume that the firm has determined that its utility function for profit is as follows:
U(X) = X - 0.05X2
Calculate the expected utility of each project and identify the preferred project according to this criterion.
(iii) Is the firm risk averse, risk neutral, or risk seeking? How can you tell?
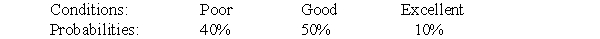 (i) Calculate the expected value of each project and identify the preferred project according to this criterion.
(i) Calculate the expected value of each project and identify the preferred project according to this criterion.(ii) Assume that the firm has determined that its utility function for profit is as follows:
U(X) = X - 0.05X2
Calculate the expected utility of each project and identify the preferred project according to this criterion.
(iii) Is the firm risk averse, risk neutral, or risk seeking? How can you tell?

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
14
A firm is considering three business projects. Project A will return a profit of $5 if conditions are poor, $10 if conditions are good, and $15 if conditions are excellent. Project B will return a profit of $12 if conditions are poor, $8 if conditions are good, and $4 if conditions are excellent. Project C will return a profit of $3 if conditions are poor, $20 if conditions are good, and $7 if conditions are excellent.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
15
A firm is considering three business projects. Project A will return a profit of $1 if conditions are poor, $5 if conditions are good, and $8 if conditions are excellent. Project B will return a profit of $2 if conditions are poor, $2 if conditions are good, and $6 if conditions are excellent. Project C will return a profit of $10 if conditions are poor, $1 if conditions are good, and $2 if conditions are excellent.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
16
A firm is considering three business projects. Project A will return a profit of $1 if conditions are poor, $7 if conditions are good, and $8 if conditions are excellent. Project B will return a profit of $1 if conditions are poor, $4 if conditions are good, and $11 if conditions are excellent. Project C will return a profit of $8 if conditions are poor, $3 if conditions are good, and $5 if conditions are excellent.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck
17
A firm is considering three business projects. Project A will return a loss of $5 if conditions are poor, a profit of $4 if conditions are good, and a profit of $8 if conditions are excellent. Project B will return a profit of zero if conditions are poor, a profit of $2 if conditions are good, and a profit of $7 if conditions are excellent. Project C will return a profit of $1 if conditions are poor, $3 if conditions are good, and $5 if conditions are excellent.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.
(i) Use the maximin criterion to determine the preferred project. Show how you arrived at your solution.
(ii) Calculate the regret matrix.
(iii) Use the minimax regret criterion to determine the preferred project. Show how you arrived at your solution.

Unlock Deck
Unlock for access to all 17 flashcards in this deck.
Unlock Deck
k this deck


