Deck 7: The Single-Sample Z Test
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/10
Play
Full screen (f)
Deck 7: The Single-Sample Z Test
1
A researcher is interested in whether students who are musicians have higher intelligence test scores than students in the general population. The researcher predicts that playing a musical instrument is associated with higher intelligence test scores. The researcher selects a sample of 75 musicians from local high schools and gives them an intelligence test. She compares their average intelligence to that of students in the general population and finds that zobt = +1.92. This is a _____ test and the researcher should conclude _____.
A) one-tailed; that students who are musicians score significantly higher on intelligence tests
B) two-tailed; that students who are musicians score significantly higher on intelligence tests
C) one-tailed; that students who are musicians do not score significantly higher on intelligence
Tests
D) two-tailed; that students who are musicians do not score significantly higher on intelligence
Tests
A) one-tailed; that students who are musicians score significantly higher on intelligence tests
B) two-tailed; that students who are musicians score significantly higher on intelligence tests
C) one-tailed; that students who are musicians do not score significantly higher on intelligence
Tests
D) two-tailed; that students who are musicians do not score significantly higher on intelligence
Tests
one-tailed; that students who are musicians score significantly higher on intelligence tests
2
Which of the following might one do in order to increase the power of a z test?
A) Increase the sample size.
B) Use a two-tailed test.
C) Decrease sample size.
D) None of the above.
A) Increase the sample size.
B) Use a two-tailed test.
C) Decrease sample size.
D) None of the above.
Increase the sample size.
3
Given: For one section of the SAT: = 500 and = 100. The SAT was given to a sample of N = 35 and the mean SAT for this sample was 536. Calculate the z test for this sample mean. Does this sample differ significantly from the population for a two-tailed test?
The z test score is 2.13. Thus, with zcv = 1.96, this sample mean differs significantly from the population mean.
4
A researcher is interested in whether students who attend private elementary schools do better or worse on a standard test of intelligence than the general population of elementary school children. A random sample of 75 students at a private elementary school is tested and has a mean intelligence test score of 103.5. The average for the general population of elementary school children is 100 ( = 15).
-Is this a one- or two-tailed test?
-Is this a one- or two-tailed test?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
5
A researcher is interested in whether students who attend private elementary schools do better or worse on a standard test of intelligence than the general population of elementary school children. A random sample of 75 students at a private elementary school is tested and has a mean intelligence test score of 103.5. The average for the general population of elementary school children is 100 ( = 15).
-What are H0 and Ha for this study?
-What are H0 and Ha for this study?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
6
A researcher is interested in whether students who attend private elementary schools do better or worse on a standard test of intelligence than the general population of elementary school children. A random sample of 75 students at a private elementary school is tested and has a mean intelligence test score of 103.5. The average for the general population of elementary school children is 100 ( = 15).
-Compute Zobt.
-Compute Zobt.

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
7
A researcher is interested in whether students who attend private elementary schools do better or worse on a standard test of intelligence than the general population of elementary school children. A random sample of 75 students at a private elementary school is tested and has a mean intelligence test score of 103.5. The average for the general population of elementary school children is 100 ( = 15).
-What is zcv?
-What is zcv?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
8
A researcher is interested in whether students who attend private elementary schools do better or worse on a standard test of intelligence than the general population of elementary school children. A random sample of 75 students at a private elementary school is tested and has a mean intelligence test score of 103.5. The average for the general population of elementary school children is 100 ( = 15).
-Should H0 be rejected? What should the researcher conclude?
-Should H0 be rejected? What should the researcher conclude?

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
9
A researcher is interested in whether students who attend private elementary schools do better or worse on a standard test of intelligence than the general population of elementary school children. A random sample of 75 students at a private elementary school is tested and has a mean intelligence test score of 103.5. The average for the general population of elementary school children is 100 ( = 15).
-Calculate the 95% confidence interval for the population mean based on the sample mean.
-Calculate the 95% confidence interval for the population mean based on the sample mean.

Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck
10
Explain why  decreases as the size of the samples in the sampling distribution increases. Your answer should include an explanation based on the formula for
decreases as the size of the samples in the sampling distribution increases. Your answer should include an explanation based on the formula for 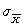 and an explanation of why this formula makes sense (in other words, why would increasing the size of each sample in the sampling distribution cause
and an explanation of why this formula makes sense (in other words, why would increasing the size of each sample in the sampling distribution cause 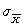 to decrease?).
to decrease?).
 decreases as the size of the samples in the sampling distribution increases. Your answer should include an explanation based on the formula for
decreases as the size of the samples in the sampling distribution increases. Your answer should include an explanation based on the formula for 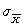 and an explanation of why this formula makes sense (in other words, why would increasing the size of each sample in the sampling distribution cause
and an explanation of why this formula makes sense (in other words, why would increasing the size of each sample in the sampling distribution cause 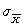 to decrease?).
to decrease?).
Unlock Deck
Unlock for access to all 10 flashcards in this deck.
Unlock Deck
k this deck


