Deck 3: Central Tendency
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/75
Play
Full screen (f)
Deck 3: Central Tendency
1
For the population of scores shown in the frequency distribution table,the mean is ____.?? ?
A)?15/5 = 3
B)?15/10 = 1.50
C)?29/5 = 5.80
D)?29/10 = 2.90
A)?15/5 = 3
B)?15/10 = 1.50
C)?29/5 = 5.80
D)?29/10 = 2.90
?29/10 = 2.90
2
A population of N = 10 scores has a mean of µ = 6.After one score is removed,the mean is found to be M = 5.What is the value of the score that was removed?
A)X = 3
B)X = 5
C)X = 10
D)X = 15
A)X = 3
B)X = 5
C)X = 10
D)X = 15
X = 15
3
A population has a mean of µ = 30.If 3 points are added to each score,what is the mean for the new distribution?
A)3
B)27
C)30
D)33
A)3
B)27
C)30
D)33
33
4
A set of N = 10 scores has a mean of µ = 50.If 20 points are added to one of the scores,what is the new value for the population mean?
A)50
B)51
C)52
D)70
A)50
B)51
C)52
D)70

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
5
A distribution of scores has a mean of µ = 50.One new score is added to the distribution and the new mean is found to be µ = 48.From this result,you can conclude that the new score was ____.
A)greater than 50
B)less than 50
C)equal to 48
D)between 50 and 52
A)greater than 50
B)less than 50
C)equal to 48
D)between 50 and 52

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
6
Which of the following actions will always change the value of the mean?
A)Changing the value of one score
B)Adding a new score to the distribution
C)Removing a score from the distribution
D)Adding a score equal to the mean.
A)Changing the value of one score
B)Adding a new score to the distribution
C)Removing a score from the distribution
D)Adding a score equal to the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
7
What is the mean for the following sample of scores? Scores: 1,4,5,10
A)20
B)10
C)5
D)4
A)20
B)10
C)5
D)4

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
8
A sample has a mean of M = 86.If one new person is added to the sample,and ΣX is unchanged,what effect will the addition have on the sample mean?
A)The sample mean will increase a little.
B)The sample mean will decrease.
C)The sample mean will remain the same.
D)The sample mean will increase substantially.
A)The sample mean will increase a little.
B)The sample mean will decrease.
C)The sample mean will remain the same.
D)The sample mean will increase substantially.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
9
A sample of n = 6 scores has a mean of M = 5.One person with a score of X = 12 is added to the distribution.What is the mean for the new set of scores?
A)M = 5
B)M = 6
C)M = 7
D)M = 8
A)M = 5
B)M = 6
C)M = 7
D)M = 8

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
10
One sample has n = 4 scores and M = 10.A second sample has n = 6 scores and M = 5.If the two samples are combined,then what is the weighted mean for the combined sample?
A)70/4 = 17.5
B)70/6 = 11.7
C)70/10 = 7.0
D)10/5=2.0
A)70/4 = 17.5
B)70/6 = 11.7
C)70/10 = 7.0
D)10/5=2.0

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
11
A sample of n = 8 scores has a mean of M = 10.After one score is removed from the sample,the mean for the remaining score is found to be M = 11.What was the score that was removed?
A)X = 3
B)X = 7
C)X = 8
D)X=10
A)X = 3
B)X = 7
C)X = 8
D)X=10

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
12
What is the mean for the population of scores shown in the frequency distribution table??
A)15/5 = 3?
B)?15/12 = 1.25
C)?32/5 = 6.60
D)?32/12 = 2.67
A)15/5 = 3?
B)?15/12 = 1.25
C)?32/5 = 6.60
D)?32/12 = 2.67

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
13
After every score in a sample is multiplied by 3,the mean is calculated and found to be M = 21.What was the mean for the original scores?
A)7
B)3
C)21
D)63
A)7
B)3
C)21
D)63

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
14
A sample has a mean of M = 40.If 10 points are added to one of the scores,what happens to the sample mean?
A)it is unchanged
B)it increases
C)it decreases a little
D)it decreases substantially
A)it is unchanged
B)it increases
C)it decreases a little
D)it decreases substantially

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
15
A population of N = 6 scores has ΣX = 30.What is the population mean?
A)180
B)30
C)15
D)5
A)180
B)30
C)15
D)5

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
16
A population with a mean of µ = 6 has ΣX = 42.How many scores are in the population?
A)N = 6/42 = 1/7
B)N = 42/6 = 7
C)N=42
D)N = 6*42 = 252
A)N = 6/42 = 1/7
B)N = 42/6 = 7
C)N=42
D)N = 6*42 = 252

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
17
In a sample of n = 6 scores,the smallest score is X = 3,the largest score is X = 10,and the mean is M = 6.If the largest score is changed from X = 10 to X = 22,then what is the value of the new mean?
A)The mean is still M = 6.
B)The mean is M = 7.
C)The mean is M = 8.
D)The mean is M=10.
A)The mean is still M = 6.
B)The mean is M = 7.
C)The mean is M = 8.
D)The mean is M=10.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
18
A sample of n = 5 scores has a mean of M = 12.What is ΣX for this sample?
A)12/5 = 2.40
B)5/12 = 0.417
C)5(12) = 60
D)52=25
A)12/5 = 2.40
B)5/12 = 0.417
C)5(12) = 60
D)52=25

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
19
What is the mean for the following scores? Scores: 1,6,14
A)3
B)6
C)7
D)10.5
A)3
B)6
C)7
D)10.5

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
20
One sample with n = 4 scores has a mean of M = 12,and a second sample with n = 6 scores has a mean of M = 8.If the two samples are combined,what is the weighted mean for the combined set of scores?
A)4.8
B)9.6
C)10.0
D)19.2
A)4.8
B)9.6
C)10.0
D)19.2

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
21
A researcher measures eye color for a sample of n = 50 people.Which measure of central tendency would be appropriate to summarize the measurements?
A)mean
B)median
C)mode
D)weighted mean
A)mean
B)median
C)mode
D)weighted mean

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
22
In a population of N = 6,five of the individuals all have scores that are exactly 1 point above the mean.From this information,what can you determine about the score for the sixth individual?
A)It is also above the mean by 1 point.
B)It is below the mean by 1 point.
C)It is below the mean by 5 points.
D)It is equal to the mean.
A)It is also above the mean by 1 point.
B)It is below the mean by 1 point.
C)It is below the mean by 5 points.
D)It is equal to the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
23
A researcher is measuring the amount of time needed to solve a set of anagrams for a sample of n = 15 students.However,one of the participants fails to solve the problems so the researcher has an undetermined score.What is the best measure of central tendency for these data?
A)mean
B)median
C)mode
D)weighted mean
A)mean
B)median
C)mode
D)weighted mean

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
24
A distribution is positively skewed.Which is the most probable order,from smallest to largest,for the three measures of central tendency?
A)mean, median, mode
B)mode, median, mean
C)mean, mode, median
D)median, mean, mode
A)mean, median, mode
B)mode, median, mean
C)mean, mode, median
D)median, mean, mode

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
25
A distribution with two modes is said to be:
A)two modal
B)minor modal
C)bimodal
D)main modal
A)two modal
B)minor modal
C)bimodal
D)main modal

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
26
For a bell-shaped distribution with µ = 30,what is the most probable value for the mode?
A)30
B)greater than 30
C)less than 30
D)0
A)30
B)greater than 30
C)less than 30
D)0

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
27
For a negatively skewed distribution with a mode of X = 25 and a median of 20,what is the most likely value for the mean?
A)greater than 25
B)equal to 25
C)between 20 and 25
D)less than 20
A)greater than 25
B)equal to 25
C)between 20 and 25
D)less than 20

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
28
Which of the following statements is true for a distribution of data points?
A)75% of the data points are above the median.
B)50% of the scores are above the median.
C)60% of the scores are below the median.
D)95% of the data points are above the median.
A)75% of the data points are above the median.
B)50% of the scores are above the median.
C)60% of the scores are below the median.
D)95% of the data points are above the median.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
29
What is the value of the median for the following set of scores? Scores: 1,3,4,6,7,8,12,23
A)6
B)6.5
C)7
D)8
A)6
B)6.5
C)7
D)8

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
30
A population of N = 7 scores has a mean of µ = 10.If one score with a value of X = 4 is removed from the population,what is the value for the new mean?
A)70/6=11.7
B)66/6=11.0
C)70/7=10.0
D)66/7=9.4
A)70/6=11.7
B)66/6=11.0
C)70/7=10.0
D)66/7=9.4

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
31
What is the mode for the following sample of n = 8 scores? Scores: 0,1,1,1,2,2,3,3
A)1
B)1.5
C)13/8 = 1.625
D)3
A)1
B)1.5
C)13/8 = 1.625
D)3

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
32
A sample of n = 4 scores has a mean of M = 8.If one new score with a value of X = 3 is added to the sample,what will be the value for the new mean?
A)M = 7.0
B)M = 8.0
C)M = 8.8
D)M=11.0
A)M = 7.0
B)M = 8.0
C)M = 8.8
D)M=11.0

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
33
What is the median for the following set of scores? Scores: 1,2,6,11,17
A)4
B)6
C)8.5
D)8
A)4
B)6
C)8.5
D)8

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
34
What is the mode for the population of scores shown in the frequency distribution table??
A)2?
B)?3
C)?3.5
D)?4
A)2?
B)?3
C)?3.5
D)?4

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
35
What is the median for the population of scores shown in the frequency distribution table??
A)2.5?
B)?3
C)?3.5
D)?4
A)2.5?
B)?3
C)?3.5
D)?4

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
36
One item on a questionnaire asks students how many times in a typical week they eat at a fast-food restaurant.The responses for a sample of n = 10 students are summarized in the frequency distribution.What is the best measure of central tendency for these data??? ?
A)mean?
B)?median
C)?mode
D)?weighted mean
A)mean?
B)?median
C)?mode
D)?weighted mean

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
37
Which of the following statements is true for a distribution of scores?
A)No score is exactly equal to the mean.
B)No score is exactly equal to the median.
C)At least one score is exactly equal to the mode.
D)At least one score is equal to the median.
A)No score is exactly equal to the mean.
B)No score is exactly equal to the median.
C)At least one score is exactly equal to the mode.
D)At least one score is equal to the median.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
38
A population of scores has a mean of µ = 26,a median of 23,and a mode of 22.What is the most likely shape for the population distribution?
A)symmetrical
B)positively skewed
C)negatively skewed
D)bimodal
A)symmetrical
B)positively skewed
C)negatively skewed
D)bimodal

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
39
For a perfectly symmetrical distribution with a median of 30,what is the value of the mean?
A)greater than 30
B)30
C)20 to 29.5
D)less than 20
A)greater than 30
B)30
C)20 to 29.5
D)less than 20

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
40
For a negatively skewed distribution with a mean of M = 20,what is the most probable value for the median?
A)greater than 20
B)20
C)less than 20
D)0
A)greater than 20
B)20
C)less than 20
D)0

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
41
For a 100-point exam,a score of X = 65 is definitely above the median.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
42
A sample has n = 7 scores with a mean of M = 7.If one individual with a score of X = 1 is removed from the sample,the new mean will be M = 8.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
43
A sample of n = 8 scores has a mean of M = 16.For this sample ΣX = 2.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
44
A sample of n = 10 scores has a mean of 50.A second sample has n = 5 scores and M = 60.If the two samples are combined,the sample mean for the combined sample will be greater than 55.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
45
A student takes a 10-point quiz each week in statistics class.If the student's quiz scores for the first three weeks are 2,6,and 10,then the mean score is M = 9.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
46
A sample with a mean of M = 20 has ΣX = 120.There are n = 6 scores in the sample.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
47
A sample has a mean of M = 40.If a new score of X = 55 is added to the sample,then the sample mean would increase.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
48
It is possible for a distribution to have more than one mode.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
49
A sample with modes of 4,8,and 10 is said to be multimodal.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
50
Changing the value of a score in a distribution will always change the value of the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
51
Every dataset will have a mode.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
52
For the scores in the following frequency distribution table,the mean is about M = 3.?

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
53
If a sample has an odd number of scores,at least one individual will have a score exactly equal to the median.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
54
A sample of n = 7 scores has a mean of M = 5.After one new score is added to the sample the new mean is calculated to be M = 6.The new score was X = 13.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
55
A sample of n = 6 scores has ΣX = 48.This sample has a mean of M = 8.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
56
A sample has n = 5 scores: 2,4,5,8,and 11.The median for the sample is 6.5.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
57
Adding a new score to a distribution will always change the value of the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
58
The major mode occurs with less frequency than the minor mode.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
59
The mean is considered to be the "balance point" for a distribution because exactly half of the scores are located above the mean and exactly half are below the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
60
A sample of n = 6 scores has a mean of M = 9.If one individual with a score of X = 4 is removed from the sample,the new mean will be M = 10.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
61
A distribution of scores has a mean of 50,a median of 53,and a mode of 56.Based on this information it appears that the distribution is negatively skewed.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
62
If a negatively skewed distribution has a mean of 50,then the median and the mode are both probably greater than 50.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
63
Means that are on an interval or ratio scale are best shown graphically on a line graph.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
64
For a set of scores measured on an ordinal scale,the median is the preferred measure of central tendency.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
65
For a distribution with one or two extreme scores,the median is usually a more representative value than the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
66
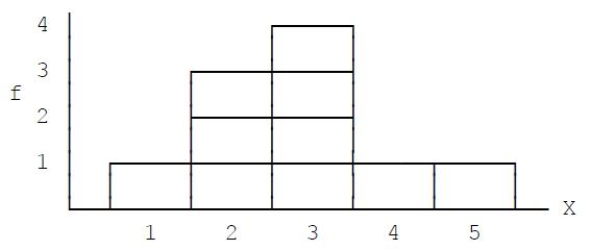 Figure 3.1
Figure 3.1Find the mean,the median,and the mode for the set of scores in the accompanying frequency distribution histogram,Figure 3.1.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
67
Explain why it is necessary to have more than one standard procedure for defining and measuring central tendency.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
68
For a negatively skewed distribution,the mean usually has a larger value than either the median or the mode.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
69
Compute the mean,median,and mode for the set of scores shown in the following frequency distribution table. 


Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
70
It is impossible for the value of the mode to be greater than the value of the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
71
What is the purpose for obtaining a measure of central tendency?

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
72
It is possible to have a distribution of scores where no individual has a score exactly equal to the mode.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
73
There are situations for which it is either impossible to compute a mean or the mean does not provide a central,representative value.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
74
A set of n = 6 scores has a mean of M = 10.Another set of scores has n = 4 and M = 15.If these two sets of scores are combined,what is the weighted mean for the combined group?

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck
75
For a severely skewed distribution,the median often provides a better measure of central tendency than the mean.

Unlock Deck
Unlock for access to all 75 flashcards in this deck.
Unlock Deck
k this deck


