Exam 7: Time to Event Data
Exam 1: An Overview of Statistical Concepts20 Questions
Exam 2: Continuous Data- Making Comparisons20 Questions
Exam 3: Continuous Data: Correlation and Regression20 Questions
Exam 4: Categorical Data: Comparisons and Associations20 Questions
Exam 5: A Dichotomous Outcome: Confounding and Regression23 Questions
Exam 6: Count Data20 Questions
Exam 7: Time to Event Data20 Questions
Select questions type
Identify the following events as right-censored, left-censored, or interval-censored. Provide a justification for your choice.
a.A person without an event who drops out of the study because he/she is too busy to participate.
b.Suppose the event of interest is the presence of tumors in rats. A rat dies during the study period but the death was before a tumor is detected.
c.Suppose the event of interest is menarche. A female entered the study and the menarche had already occurred.
d.Suppose the event of interest is dental caries in children. A healthy child entered the study with no cavities but came back one year later with a cavity.
Free
(Essay)
4.7/5  (29)
(29)
Correct Answer:
a.It is a right-censored observation because no event will be observed during the study period. An event is expected to occur after the study period.
b.It is a right-censored observation because an event will not be observed in this rat.
c.It is a left-censored observation where the event of interest happened before the study starts.
d.It is an interval-censored observation because an event happened between time of entry and one year after.
Which of the following statements is NOT true about hazard rates?
Free
(Multiple Choice)
4.9/5  (40)
(40)
Correct Answer:
B
The major assumption of proportional-hazards regression is that the hazards are proportional. Explain what this means.
Free
(Essay)
4.9/5  (43)
(43)
Correct Answer:
In order to use the proportional-hazards regression model, it is assumed that the difference in risks between any two comparison groups is constant over time.
Which of the following equations does NOT represent the proportional-hazards regression model?
(Multiple Choice)
4.7/5  (26)
(26)
Suppose an investigator is interested to study the relationship between hormone therapy treatments (treatment A, treatment B, and no treatment) and time to recurrence of breast cancer. Age (years), postmenopause (yes/no), and tumor size (millimeter) are also considered as possible predictors.
a.Using proportional-hazards regression to investigate this research question, what is the statistical model?
b.Provide appropriate global null and research hypotheses for the model in (a). What would you conclude if the null hypothesis is rejected?
c.Provide appropriate null and research hypotheses for the specific effect of each hormone therapy. What does a rejected null hypothesis imply?
d.If the estimate HRT A is -0.70 (95% CI: -0.84, -0.56) and the control is used as the reference, provide an estimate of the hazard ratio and its 95% confidence interval and interpret.
(Essay)
4.9/5  (34)
(34)
Describe how an interaction with time in a proportional-hazards model can be used to check the proportional hazards assumption.
(Essay)
4.8/5  (44)
(44)
Describe the difference between a survival function and a hazard function?
(Essay)
4.9/5  (35)
(35)
Suppose the hazard ratio of cancer recurrence is 1.45 (95% CI: 1.32, 1.58) when comparing the hazard rates of male patients to female patients. Interpret this result.
(Essay)
4.9/5  (33)
(33)
Can the log-rank test be used to compare survival curves at a specific time point? Why or why not?
(Essay)
4.9/5  (39)
(39)
How does the Kaplan-Meier estimator of the survival function differ from the percentage of subjects with the event?
(Essay)
4.8/5  (42)
(42)
Suppose a study is conducted to investigate the risk factors associated with the time to receive treatment after the start of stroke symptoms. The proportional-hazards model is fitted. Estimate the adjusted hazard ratio for every 5 units of body mass index (BMI) and interpret the result. Include the 95% confidence interval and interpret.

(Essay)
4.9/5  (42)
(42)
In the presence of tied event times (i.e., events that occur at the same time), what methods can be used to compute the likelihood? When would each method be preferred?
(Essay)
4.9/5  (38)
(38)
The survival function plot for time until hip fractures are healed is produced using the Kaplan-Meier method. Based on the plot, which gender has a better recovery experience? Describe the plot of each gender at time point 3.
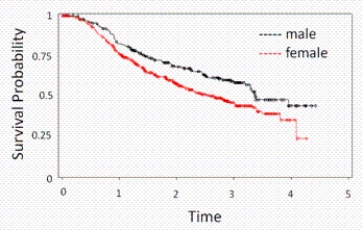
(Essay)
4.7/5  (38)
(38)
Describe in what scenario you might observe this hazard function plot.
(Essay)
4.8/5  (39)
(39)
What is the difference between a life table method and Kaplan-Meier method in estimating the survival probability? Provide an example when the life table method is preferred to the Kaplan-Meier method.
(Essay)
4.8/5  (27)
(27)
Which of the following methods is used to estimate the b's (coefficient of parameters) in a proportional hazards regression?
(Multiple Choice)
4.8/5  (37)
(37)
How does the Kaplan-Meier method estimate the survival probability in the presence of censoring?
(Essay)
4.8/5  (42)
(42)
Which of the following statements is NOT true about the survival function?
(Multiple Choice)
4.8/5  (44)
(44)
Describe the types of outcomes appropriate for time-to-event analyses. Provide a specific example.
(Essay)
4.8/5  (33)
(33)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)