Exam 3: Functions and Graphs
Exam 1: Review of Algebra470 Questions
Exam 2: Applications and More Algebra229 Questions
Exam 3: Functions and Graphs237 Questions
Exam 4: Lines parabolas and Systems218 Questions
Exam 5: Exponential and Logarithmic Functions258 Questions
Exam 6: Mathematics of Finance205 Questions
Exam 7: Matrix Algebra173 Questions
Exam 8: Linear Programming44 Questions
Exam 9: Introduction to Probability and Statistics126 Questions
Exam 10: Additional Topics in Probability45 Questions
Exam 11: Limits and Continuity241 Questions
Exam 12: Differentiation283 Questions
Exam 13: Additional Differentiation Topics191 Questions
Exam 14: Curve Sketching161 Questions
Exam 15: Integration261 Questions
Exam 16: Methods and Applications of Integration152 Questions
Exam 17: Continuous Random Variables88 Questions
Exam 18: Multivariable Calculus108 Questions
Select questions type
If f(x)= 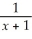 and g(x)= x + 1,find: (a)f(g(x))and (b)g(f(x)).
and g(x)= x + 1,find: (a)f(g(x))and (b)g(f(x)).
Free
(Essay)
4.7/5  (35)
(35)
Correct Answer:
(a) 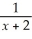
(b) 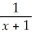 + 1 =
+ 1 = 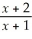
Julie lives 32 miles from the city.She drove home from the city at a constant rate of 60 mph along the highway.At the exit 2 miles from her home,she realized she had left her purse at the department store.She immediately returned to the department store at a rate of 60 mph.Graph the absolute-value function to represent Julie's distance from home as she drove home from the city over the appropriate domain.
(Essay)
4.8/5  (36)
(36)
Suppose the weekly supply function for a large pizza at a local pizza parlor is p = 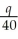 .
(a)How many large pizzas will be supplied if the price is $12.50 per pizza?
(b)How many large pizzas will be supplied if the price is $18.75 per pizza?
(c)How does the amount supplied change as the price increases?
.
(a)How many large pizzas will be supplied if the price is $12.50 per pizza?
(b)How many large pizzas will be supplied if the price is $18.75 per pizza?
(c)How does the amount supplied change as the price increases?
(Essay)
4.8/5  (28)
(28)
In June Gail decided to save $20.00 a week.She saved for 14 weeks and then for 14 weeks she spent $20.00 a week on gifts.Write an absolute-value function to represent the amount of money Gail had in savings.
(Essay)
4.7/5  (31)
(31)
Use a graphing calculator to find all real roots of the equation.Round answers to two decimal places,if necessary: 
(Short Answer)
4.7/5  (37)
(37)
Determine whether the graph of y = 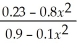 is symmetric about the x-axis,the y-axis,the origin,or the line y = x.
is symmetric about the x-axis,the y-axis,the origin,or the line y = x.
(Short Answer)
4.8/5  (32)
(32)
A coat costs x wholesale.The price the store pays is given by the function s(x)= 1.2x where x is the wholesale price.The price the customer pays is c(x)= 2x + 50 where x is the price the store pays.Write a composite function to find the customer's price as a function of the wholesale price.
(Short Answer)
4.8/5  (34)
(34)
If f(x)= 5 - x and g(x)= 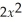 - 3x + 4,find:
(a)(f + g)(x)
(b)(f - g)(x)
(c)(f - g)(2)
(d)(fg)(x)
(e)(fg)(0)
(f)
- 3x + 4,find:
(a)(f + g)(x)
(b)(f - g)(x)
(c)(f - g)(2)
(d)(fg)(x)
(e)(fg)(0)
(f) 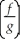 (x)
(g)f(g(x))
(h)g(f(x))
(i)g(f(1))
(x)
(g)f(g(x))
(h)g(f(x))
(i)g(f(1))
(Essay)
4.8/5  (33)
(33)
Showing 1 - 20 of 237
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)