Exam 13: Vector Calculus
Exam 1: Functions and Limits51 Questions
Exam 2: Derivatives50 Questions
Exam 3: Inverse Functions: Exponential, Logarithmic, and Inverse Trigonometric Functions56 Questions
Exam 4: Applications of Differentiation29 Questions
Exam 5: Integrals41 Questions
Exam 6: Techniques of Integration43 Questions
Exam 7: Applications of Integration61 Questions
Exam 8: Series50 Questions
Exam 9: Parametric Equations and Polar Coordinates30 Questions
Exam 10: Ectors and the Geometry of Space68 Questions
Exam 11: Partial Derivatives71 Questions
Exam 12: Multiple Integrals54 Questions
Exam 13: Vector Calculus56 Questions
Select questions type
Find the work done by the force field 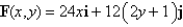 in moving an object along an arch of the cycloid
in moving an object along an arch of the cycloid 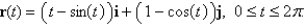
(Short Answer)
4.8/5  (41)
(41)
Find the area of the surface S where S is the part of the surface 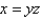 that lies inside the cylinder
that lies inside the cylinder 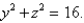
(Short Answer)
4.8/5  (39)
(39)
Let f be a scalar field.Determine whether the expression is meaningful.If so,state whether the expression represents a scalar field or a vector field. 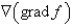
(Short Answer)
4.8/5  (29)
(29)
The flow lines (or streamlines)of a vector field are the paths followed by a particle whose velocity field is the given vector field.Thus,the vectors in a vector field are tangent to the flow lines.The flow lines of the vector field 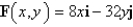 satisfy the differential equations
satisfy the differential equations 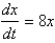 and
and 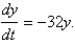 Solve these differential equations to find the equations of the family of flow lines.
Solve these differential equations to find the equations of the family of flow lines.
(Short Answer)
4.8/5  (37)
(37)
Use Gauss's Law to find the charge contained in the solid hemisphere 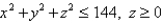 ,if the electric field is
,if the electric field is 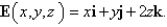
(Short Answer)
4.8/5  (37)
(37)
Let f be a scalar field.Determine whether the expression is meaningful.If so,state whether the expression represents a scalar field or a vector field.
curl f
(Short Answer)
4.9/5  (35)
(35)
Find an equation of the tangent plane to the parametric surface represented by r at the specified point. 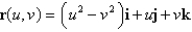 ;
; 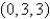
(Short Answer)
4.8/5  (38)
(38)
Let D be a region bounded by a simple closed path C in the xy.Then the coordinates of the centroid 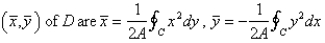 where A is the area of D. Find the centroid of the triangle with vertices (0,0),(
where A is the area of D. Find the centroid of the triangle with vertices (0,0),( 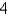 ,0)and (0,
,0)and (0, 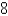 ).
).
(Multiple Choice)
4.8/5  (34)
(34)
Suppose that 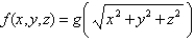 where g is a function of one variable such that
where g is a function of one variable such that  . Evaluate
. Evaluate 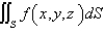 where S is the sphere
where S is the sphere 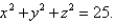
(Multiple Choice)
4.9/5  (27)
(27)
Use Stokes' Theorem to evaluate 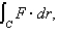 where
where 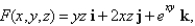
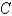 is the circle
is the circle 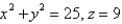 .
. 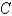 is oriented counterclockwise as viewed from above.
is oriented counterclockwise as viewed from above.
(Multiple Choice)
4.8/5  (32)
(32)
Use Green's Theorem to evaluate the line integral along the positively oriented closed curve C. 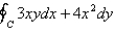 ,where C is the triangle with vertices
,where C is the triangle with vertices 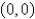 ,
, 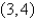 ,and
,and  .
.
(Multiple Choice)
4.9/5  (41)
(41)
A particle starts at the point 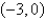 ,moves along the x-axis to (3,0)and then along the semicircle
,moves along the x-axis to (3,0)and then along the semicircle  to the starting point.Use Green's Theorem to find the work done on this particle by the force field
to the starting point.Use Green's Theorem to find the work done on this particle by the force field 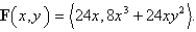
(Multiple Choice)
5.0/5  (34)
(34)
Use the Divergence Theorem to calculate the surface integral  ; that is,calculate the flux of
; that is,calculate the flux of 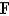 across
across 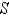 .
.  S is the surface of the box bounded by the coordinate planes and the planes
S is the surface of the box bounded by the coordinate planes and the planes 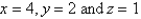 .
.
(Multiple Choice)
5.0/5  (30)
(30)
Showing 21 - 40 of 56
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)