Exam 11: Partial Derivatives
Exam 1: Functions and Limits51 Questions
Exam 2: Derivatives50 Questions
Exam 3: Inverse Functions: Exponential, Logarithmic, and Inverse Trigonometric Functions56 Questions
Exam 4: Applications of Differentiation29 Questions
Exam 5: Integrals41 Questions
Exam 6: Techniques of Integration43 Questions
Exam 7: Applications of Integration61 Questions
Exam 8: Series50 Questions
Exam 9: Parametric Equations and Polar Coordinates30 Questions
Exam 10: Ectors and the Geometry of Space68 Questions
Exam 11: Partial Derivatives71 Questions
Exam 12: Multiple Integrals54 Questions
Exam 13: Vector Calculus56 Questions
Select questions type
Use Lagrange multipliers to find the maximum value of the function subject to the given constraints. 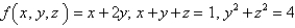
Free
(Short Answer)
4.9/5  (36)
(36)
Correct Answer:
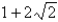
Use partial derivatives to find the implicit derivative 
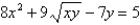
Free
(Short Answer)
4.9/5  (28)
(28)
Correct Answer:
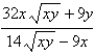
Use Lagrange multipliers to find the maximum value of the function subject to the given constraint. 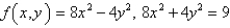
(Multiple Choice)
4.8/5  (35)
(35)
The radius of a right circular cone is increasing at a rate of 5 in/s while its height is decreasing at a rate of 3.6 in/s.At what rate is the volume of the cone changing when the radius is 108 in.and the height is 132 in.?
(Multiple Choice)
4.9/5  (30)
(30)
The length l,width w and height h of a box change with time.At a certain instant the dimensions are 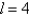 and
and 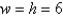 ,and l and w are increasing at a rate of 10 m/s while h is decreasing at a rate of 1 m/s.At that instant find the rates at which the surface area is changing.
,and l and w are increasing at a rate of 10 m/s while h is decreasing at a rate of 1 m/s.At that instant find the rates at which the surface area is changing.
(Short Answer)
5.0/5  (37)
(37)
The ellipsoid 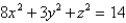 intersects the plane
intersects the plane 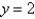 in an ellipse.Find parametric equations for the tangent line to this ellipse at the point (1,2,2).
in an ellipse.Find parametric equations for the tangent line to this ellipse at the point (1,2,2).
(Multiple Choice)
4.9/5  (32)
(32)
Find the absolute minimum value of the function 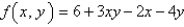 on the set D.D is the region bounded by the parabola
on the set D.D is the region bounded by the parabola  and the line
and the line 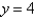
(Multiple Choice)
4.9/5  (34)
(34)
Find the points on the surface 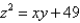 that are closest to the origin.
that are closest to the origin.
(Multiple Choice)
4.7/5  (49)
(49)
Determine the largest set on which the function is continuous. 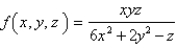
(Short Answer)
4.7/5  (31)
(31)
Suppose that over a certain region of space the electrical potential V is given by 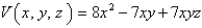 . Find the rate of change of the potential at
. Find the rate of change of the potential at 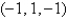 in the direction of the vector
in the direction of the vector  .
.
(Multiple Choice)
4.8/5  (39)
(39)
Suppose (1,1)is a critical point of a function f with continuous second derivatives. In the case of 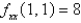 ,
, 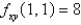 ,
, 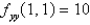 what can you say about f ?
what can you say about f ?
(Multiple Choice)
4.8/5  (41)
(41)
Find the local maximum,and minimum value and saddle points of the function. 
(Short Answer)
4.9/5  (31)
(31)
Showing 1 - 20 of 71
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)