Solved

Graphically Approximate the Limit (If It Exists)by Using a Graphing lim⁡x→36+6−xx−36\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 }

Question 60

Multiple Choice

Graphically approximate the limit (if it exists) by using a graphing utility to graph the function. lim⁡x→36+6−xx−36\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 }


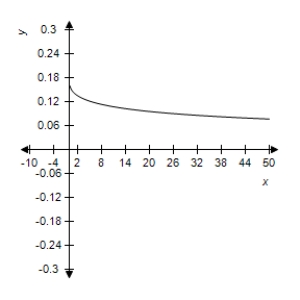
A) lim⁡x→36+6−xx−36=−113\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 13 } Graphically approximate the limit (if it exists) by using a graphing utility to graph the function. \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } A) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 13 } B) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 13 } C) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 12 } D) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 12 } E) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 14 }
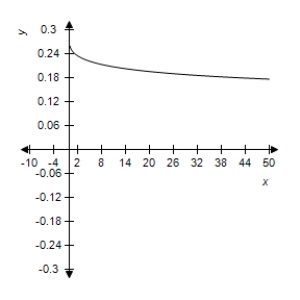
B) lim⁡x→36+6−xx−36=113\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 13 } Graphically approximate the limit (if it exists) by using a graphing utility to graph the function. \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } A) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 13 } B) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 13 } C) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 12 } D) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 12 } E) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 14 }
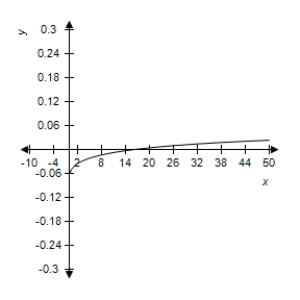
C) lim⁡x→36+6−xx−36=112\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 12 } Graphically approximate the limit (if it exists) by using a graphing utility to graph the function. \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } A) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 13 } B) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 13 } C) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 12 } D) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 12 } E) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 14 }
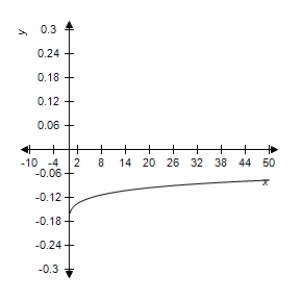
D) lim⁡x→36+6−xx−36=−112\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 12 } Graphically approximate the limit (if it exists) by using a graphing utility to graph the function. \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } A) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 13 } B) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 13 } C) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 12 } D) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 12 } E) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 14 }
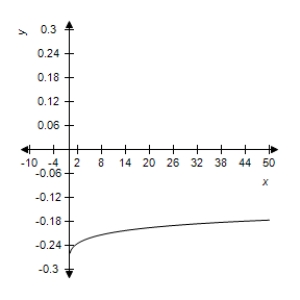
E) lim⁡x→36+6−xx−36=114\lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 14 } Graphically approximate the limit (if it exists) by using a graphing utility to graph the function. \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } A) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 13 } B) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 13 } C) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 12 } D) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = - \frac { 1 } { 12 } E) \lim _ { x \rightarrow 36 ^ { + } } \frac { 6 - \sqrt { x } } { x - 36 } = \frac { 1 } { 14 }

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q55: Use the limit process to find

Q56: Find <span class="ql-formula" data-value="\lim _

Q57: Select the graph of the derivative

Q58: Find <span class="ql-formula" data-value="\lim _

Q59: Algebraically evaluate the limit (if it

Q61: Find the limit by direct substitution.

Q62: Find a formula for the slope

Q63: Find <span class="ql-formula" data-value="\lim _

Q64: Evaluate <span class="ql-formula" data-value="\sum _

Q65: Find the limit (if it exists).