The Shoe Company Plans the Number of Shoe Pairs of Each

Multiple Choice

The shoe company plans the number of shoe pairs of each size before the release of a new men's collection. To do this, sales data from several outlets in the last month were taken. Minitab was used to perform a chi-square goodness-of-fit test for these data. The proportion of each size which was used for the last collection is in the "Test proportion" column. Interpret the Minitab output to determine whether the size proportions for the new collection should be changed. Use a significance level of 0.01. ​ ​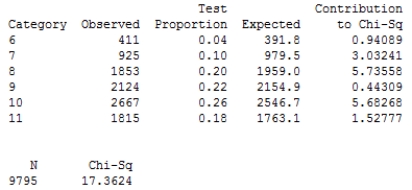

A) 0.001 < P-value < 0.005, there is convincing evidence that the size proportions should be changed.
B) 0.005 < P-value < 0.010, there is convincing evidence that the size proportions should be changed.
C) 0.015 < P-value < 0.020, there is no convincing evidence that the size proportions should be changed.
D) 0.020 < P-value < 0.025, there is no convincing evidence that the size proportions should be changed.
E) 0.025 < P-value < 0.030, there is no convincing evidence that the size proportions should be changed.