Find and Simplify the Difference Quotient of F $$\frac { f ( x + h ) - f ( x ) } { h }$$

Multiple Choice

Find and simplify the difference quotient of f, $$\frac { f ( x + h ) - f ( x ) } { h }$$ $\mathbf { h } \neq 0$ , for the function.
- A)
$\begin{array}{l}\begin{array}{l}\text { function } \\\text { domain: }\{x \mid x \leq 0\} \\\text { range: }\{y \mid y \geq-2\} \\\text { intercepts: }(-2,0) ,(0,-2) ,(2,0) \end{array}\\\text { symmetry: } y \text {-axis }\end{array}$

B)
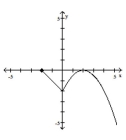
function
domain: $\{x \mid x \geq-2\}$
range: $\{y \mid y \leq 0\}$
intercepts: $(-2,0) ,(0,-2) ,(2,0)$
symmetry: none

C)
function
domain: all real numbers
range: all real numbers
intercepts: $(-2,0) ,(0,-2) ,(2,0)$
symmetry: none

D) not a function