Multiple Choice
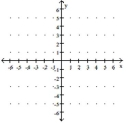
Decide whether the relation is a function.
- 
A) g shifts the graph of f vertically
down 2 units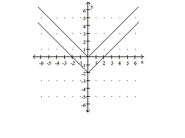
B) shifts the graph of vertically
up 2 units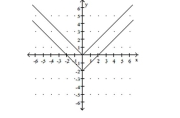
C) shifts the graph of f vertically
up 2 units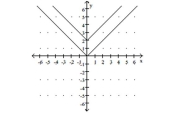
D) shifts the graph of vertically
down 2 units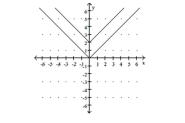
Correct Answer:

Verified
Correct Answer:
Verified
Related Questions
Q67: Graph the equation in the rectangular
Q68: Use intercepts and a checkpoint to
Q69: Find the indicated function value.<br>- <span
Q70: Decide whether the relation is a
Q71: Rewrite the given equation in slope-intercept
Q73: Use the vertical line test to determine
Q74: Find the slope of the line
Q75: For the pair of functions, determine
Q76: Use the vertical line test to determine
Q77: Find the requested value.<br>- <span class="ql-formula"