Multiple Choice
On January 1, 1879, records show that 500 of a fish called Atlantic striped bass were introduced into the San Francisco Bay. In 1899, the first year fishing for bass was allowed, 100,000 of these bass were caught, representing 10% of the population at the start of 1899. Owing to reproduction, at any time the bass population is growing at a rate proportional to the population at that moment. Assume that when fishing starts in 1899, the rate at which bass are caught is proportional to the square of the population with constant of proportionality 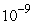 . What happens to the bass population in the long run?
. What happens to the bass population in the long run?
A) It approaches 0.
B) It grows without bound.
C) It approaches 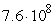 .
.
D) It approaches 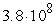 .
.
Correct Answer:

Verified
Correct Answer:
Verified
Q12: Given that <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB8190/.jpg" alt="Given that
Q13: A lake with constant volume V, in
Q14: According to Newton, the rate at which
Q15: Carbon-14 decays at a rate proportional to
Q16: If no more pollutants are dumped into
Q18: What is the solution to the differential
Q19: Consider the differential equation for the logistic
Q20: For <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB8190/.jpg" alt="For to
Q21: The solution to the differential equation <img
Q22: A cup of green tea contains 32