Use a Graphing Utility to Graph the Function, Approximate the Relative

Multiple Choice

Use a graphing utility to graph the function, approximate the relative minimum or maximum of the function, and estimate the open intervals on which the function is increasing or decreasing. $f ( x ) = x ^ { 2 } - 4 x + 1$

A) 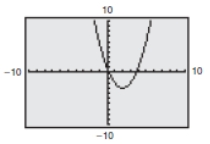Decreasing on $( - \infty , 2 )$ Increasing on $( 2 , \infty )$ Relative minimum: $( 2 , - 3 )$
B) 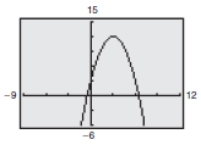Decreasing on $( 3 , \infty )$ Increasing on $( - \infty , 3 )$ Relative maximum: $( 3,12 )$
C) 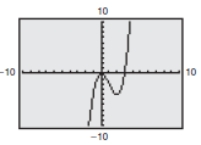Decreasing on $( 0,2 )$ Increasing on $( - \infty , 0 ) , ( 2 , \infty )$ Relative minimum: $( 0,0 )$ Relative maximum: $( 2 , - 4 )$
D) 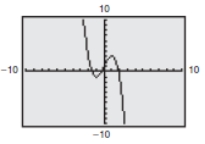Decreasing on $( - \infty , - 1 ) , ( 1 , \infty )$ Increasing on $( - 1,1 )$ Relative minimum: $( - 1 , - 1 )$ Relative maximum: $( 1,3 )$
E) 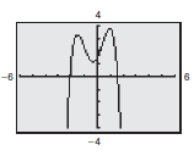Decreasing on $( 1 , \infty )$ Increasing on $( - \infty , 1 )$ Relative minimum: $( - 1,1 )$ Relative maximum: $( 1,2 )$