Multiple Choice
Verify that the vector field F = (2x y2z2 - sin(x) sin(y) ) i + (2 x2y z2+ cos(x) cos(y) ) j + (2x2y2 z + ) k is conservative and find a scalar potential f(x, y, z) for it that satisfies f(0, 0, 0) = 1.
A) 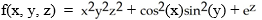
B) f(x, y, z) = 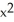
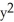
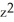 + cos(x) sin(y) +
+ cos(x) sin(y) + 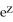 + 1
+ 1
C) f(x, y, z) = 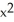
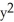
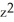 + sin(x) cos(y) +
+ sin(x) cos(y) + 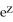 + 1
+ 1
D) f(x, y, z) = 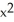
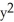
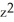 + cos(x) sin(y) +
+ cos(x) sin(y) + 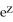
E) f(x, y, z) = xyz + cos(x) sin(y) + 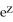
Correct Answer:

Verified
Correct Answer:
Verified
Q7: Show that div ( <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB9661/.jpg" alt="Show
Q8: Use Green's Theorem to compute the
Q9: Compute the gradient of the function f(x,
Q10: Find the flux of F =
Q11: If F = x i +
Q13: Compute div F for F = (2x
Q14: If r = x i + y
Q15: Evaluate <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB9661/.jpg" alt="
Q16: Use Stokes's Theorem to evaluate the line
Q17: Calculate the divergence of the vector field