Solved

Use Lagrange Multipliers to Find the Point P

Question 3

Multiple Choice

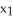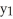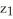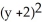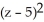Use Lagrange multipliers to find the point P( Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. 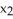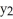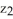A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units , Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units , Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units ) on the sphere Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units + Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units + Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units = 9 and the point Q ( Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units , Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units , Use Lagrange multipliers to find the point P( , , ) on the sphere + + = 9 and the point Q ( , , ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance. A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units ) on the plane x + 2y - 2z = 7 where the distance between P and Q is minimum and determine this minimum distance.


A) P (2, -4, 7) , Q (5, 2, 1) ; minimum distance 9 units
B) P (4, 0, 3) , Q (3, -2, 5) ; minimum distance 3 units
C) P (4, 0, 3) , Q (5, 2, 1) ; minimum distance 3 units
D) P (2, -4, 7) , Q (-1, -10, 13) ; minimum distance 9 units
E) P (2, -4, 7) , Q (1, -6, 9) ; minimum distance 3 units

Correct Answer:

verifed

Verified

Related Questions

Q1: Find an optimal binary compression for a

Q2: Solve the integral equation f(x) = 3

Q4: Find the value of the constant m

Q5: Find the critical points of f(x, y)

Q6: Find the maximum and minimum values of

Q7: Evaluate F(x,y) = <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB9661/.jpg" alt="Evaluate F(x,y)

Q8: Find and classify the critical points of

Q9: A rectangular box with no top has

Q10: Every one-parameter family of curves in the

Q11: Find the points closest to the origin