Suppose a 128-Lb Object Stretches a Spring 3 Feet While

Short Answer

Suppose a 128-lb object stretches a spring 3 feet while in equilibrium, and a dashpot provides a damping force of 5 lbs for every foot per second of velocity. The form of the equation of unforced motion of the object in such a spring-mass system is 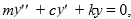where m is the mass of the object, c is the damping constant, and k is the spring constant. Assume that the spring starts at a height of 5/2 feet below the horizontal with an upward velocity of - 3 feet per second.
For what values of the arbitrary constants C₁ and C₂ does a general solution of the form y(t) = 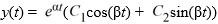satisfy the initial conditions? Provide the exact values, not decimal approximations.
C₁__________, C₂____________