A Two-Sample Z-Test for Two Population Proportions Is to Be

Multiple Choice

A two-sample z-test for two population proportions is to be performed using the P-value approach.The null hypothesis is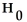
:
=
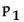
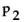
and the alternative is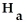
:
≠
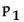
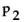
.Use the given sample data to find the P-value for the hypothesis test.Give an interpretation of the p-value.
-n₁ = 200 n₂ = 100
X₁ = 11 x₂ = 8

A) P-value = 0.2005;There is about a 20.05% chance that the two proportions are equal
B) P-value = 0.401;There is about a 40.1% chance that the two proportions are equal.
C) P-value = 0.401;If there is no difference in the proportions,there is about a 40.1% chance of seeing the observed difference or larger by natural sampling variation.
D) P-value = 0.2005;If there is no difference in the proportions,there is about a 20.05% chance of seeing the observed difference or larger by natural sampling variation.
E) P-value = 0.401;If there is a difference in the proportions,there is a 40.1% chance of seeing the observed difference by natural sampling variation.