Solved

Suppose That a Marksman Hits the Bull's-Eye 1 Time in 10

Question 3

Multiple Choice

Suppose that a marksman hits the bull's-eye 1 time in 10 shots. If the next 4 shots are independent, find the probability that exactly two of the next 4 shots hit the bull's-eye. ​


A) Pr(two bull's-eyes) = Suppose that a marksman hits the bull's-eye 1 time in 10 shots. If the next 4 shots are independent, find the probability that exactly two of the next 4 shots hit the bull's-eye. ​ A) Pr(two bull's-eyes) = B) Pr(two bull's-eyes) = C) Pr(two bull's-eyes) = D) Pr(two bull's-eyes) = E) Pr(two bull's-eyes) =
B) Pr(two bull's-eyes) = Suppose that a marksman hits the bull's-eye 1 time in 10 shots. If the next 4 shots are independent, find the probability that exactly two of the next 4 shots hit the bull's-eye. ​ A) Pr(two bull's-eyes) = B) Pr(two bull's-eyes) = C) Pr(two bull's-eyes) = D) Pr(two bull's-eyes) = E) Pr(two bull's-eyes) =
C) Pr(two bull's-eyes) = Suppose that a marksman hits the bull's-eye 1 time in 10 shots. If the next 4 shots are independent, find the probability that exactly two of the next 4 shots hit the bull's-eye. ​ A) Pr(two bull's-eyes) = B) Pr(two bull's-eyes) = C) Pr(two bull's-eyes) = D) Pr(two bull's-eyes) = E) Pr(two bull's-eyes) =
D) Pr(two bull's-eyes) = Suppose that a marksman hits the bull's-eye 1 time in 10 shots. If the next 4 shots are independent, find the probability that exactly two of the next 4 shots hit the bull's-eye. ​ A) Pr(two bull's-eyes) = B) Pr(two bull's-eyes) = C) Pr(two bull's-eyes) = D) Pr(two bull's-eyes) = E) Pr(two bull's-eyes) =
E) Pr(two bull's-eyes) = Suppose that a marksman hits the bull's-eye 1 time in 10 shots. If the next 4 shots are independent, find the probability that exactly two of the next 4 shots hit the bull's-eye. ​ A) Pr(two bull's-eyes) = 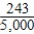B) Pr(two bull's-eyes) = 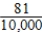C) Pr(two bull's-eyes) = 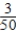D) Pr(two bull's-eyes) = 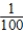E) Pr(two bull's-eyes) =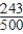

Correct Answer:

verifed

Verified

Related Questions

Q1: A Markov chain has transition matrix <img

Q2: If the probability of rain today is

Q4: Compute <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB4005/.jpg" alt="Compute .

Q6: A ball is drawn from a bag

Q7: Suppose that a person groups 18 objects

Q8: Compute <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB4005/.jpg" alt="Compute .

Q9: The local community-service funding organization in a

Q10: A red ball and 19 white balls

Q11: Compute <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB4005/.jpg" alt="Compute .

Q125: The probability that daughters of a mother