Multiple Choice
Use a graphing utility to graph the equation.Use the graph to approximate the values of x that satisfy the inequality.
Equation:
Inequality:
A) 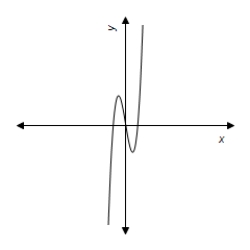
B) 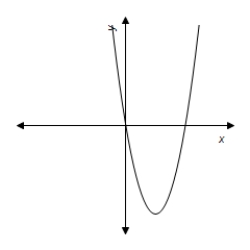
C) 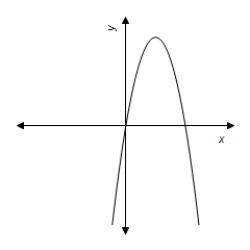
D) 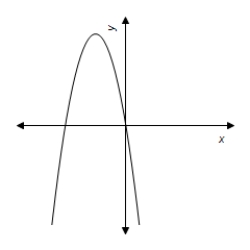
E) 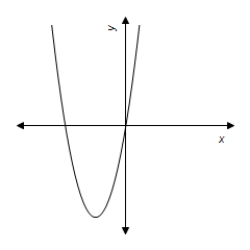
Correct Answer:

Verified
Correct Answer:
Verified
Related Questions
Q1: Solve the inequality and graph the
Q3: Use a graphing utility to graph
Q4: Solve the inequality <span class="ql-formula"
Q5: Determine whether the statement is true
Q6: Use a graphing utility to graph
Q7: Solve the inequality and graph the
Q8: Solve the inequality and graph the
Q9: Find the interval(s)for b such that
Q10: Find the key numbers of the
Q11: Find the interval(s)for b such that