Multiple Choice
The following data show the demand for an airline ticket dependent on the price of this ticket. 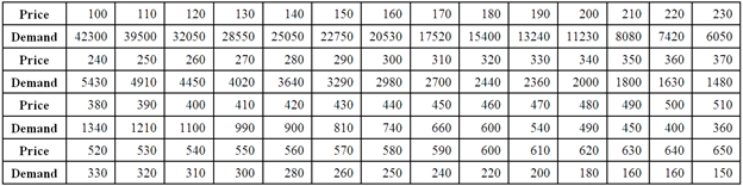 For the assumed cubic and log-log regression models,Demand = β0 + β1Price + β2Price2 + β3Price3 + ε and ln(Demand) = β0 + β1ln(Price) + ε,the following regression results are available.
For the assumed cubic and log-log regression models,Demand = β0 + β1Price + β2Price2 + β3Price3 + ε and ln(Demand) = β0 + β1ln(Price) + ε,the following regression results are available. 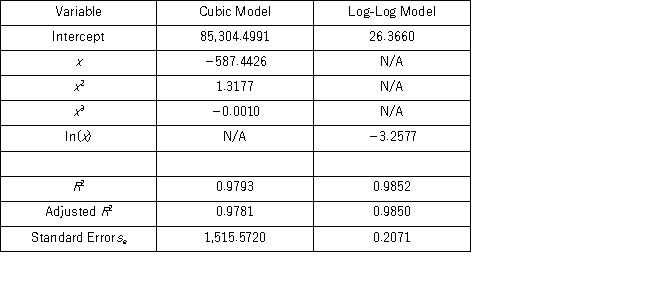 Assuming that the sample correlation coefficient between Demand and
Assuming that the sample correlation coefficient between Demand and 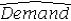 = exp(26.3660 - 3.2577 ln(Price) + (0.2071) 2/2) is 0.956,what is the predicted demand for a price of $250 found by the model with better fit?
= exp(26.3660 - 3.2577 ln(Price) + (0.2071) 2/2) is 0.956,what is the predicted demand for a price of $250 found by the model with better fit?
A) 4,447.88
B) 3,914.38
C) 4,029.38
D) 5,137.60
Correct Answer:

Verified
Correct Answer:
Verified
Q13: Thirty employed single individuals were randomly selected
Q14: Many nonlinear regression models can be studied
Q15: Typically,the sales volume declines with an increase
Q16: In which of the following models does
Q17: Thirty employed single individuals were randomly selected
Q19: Typically,the sales volume declines with an increase
Q20: The equation y = β<sub>0</sub> + β<sub>1</sub>x
Q21: The following Excel scatterplot with the fitted
Q22: Which of the following regression models is
Q56: An inverted U-shaped curve is also known