Solved

If We Want to Relate a Random Variable Y to Two

Question 31

True/False

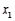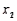If we want to relate a random variable y to two independent variables If we want to relate a random variable y to two independent variables and , a regression hyperplane is the three-dimensional equivalent of a regression line that minimizes the sum of the squared vertical deviations between the sample points suspended in y vs. vs. space and their associated multiple regression estimates, all of which lie on this hyperplane. 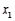and If we want to relate a random variable y to two independent variables and , a regression hyperplane is the three-dimensional equivalent of a regression line that minimizes the sum of the squared vertical deviations between the sample points suspended in y vs. vs. space and their associated multiple regression estimates, all of which lie on this hyperplane. , a regression hyperplane is the three-dimensional equivalent of a regression line that minimizes the sum of the squared vertical deviations between the sample points suspended in y vs. 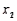If we want to relate a random variable y to two independent variables and , a regression hyperplane is the three-dimensional equivalent of a regression line that minimizes the sum of the squared vertical deviations between the sample points suspended in y vs. vs. space and their associated multiple regression estimates, all of which lie on this hyperplane. vs. If we want to relate a random variable y to two independent variables and , a regression hyperplane is the three-dimensional equivalent of a regression line that minimizes the sum of the squared vertical deviations between the sample points suspended in y vs. vs. space and their associated multiple regression estimates, all of which lie on this hyperplane. space and their associated multiple regression estimates, all of which lie on this hyperplane.

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q26: Personal Spending and Personal Income<br>Is personal spending

Q27: Life Expectancy Narrative<br>An actuary wanted to develop

Q28: In multiple regression analysis, the coefficient of

Q30: Life Expectancy Narrative<br>An actuary wanted to develop

Q32: Demographic Variables and TV Narrative<br>A statistician wanted

Q33: Chemical Comparisons Narrative<br>A chemist was interested in

Q34: For which of the following quantities is

Q35: College Textbook Sales Narrative<br>A publisher of college

Q36: If a multiple regression model includes ten

Q176: The coefficient of multiple determination is calculated