Exam 9: Infinte Series
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
Determine whether or not the following series converges. 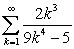
Free
(Multiple Choice)
4.9/5  (34)
(34)
Correct Answer:
A
Determine whether or not the following series converges. 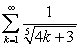
Free
(Multiple Choice)
4.8/5  (41)
(41)
Correct Answer:
B
Determine whether or not the following sequence converges. If it does converge, what is its limit? Enter "Yes" if the sequence converges, and "No" if it does not. 
Free
(Short Answer)
4.7/5  (35)
(35)
Correct Answer:
Yes, 0
Find the Taylor polynomial of order 4 for the function 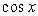 centered at
centered at 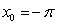 .
.
(Short Answer)
4.8/5  (35)
(35)
Find the Taylor polynomial of order 2 for 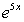 , centered about
, centered about 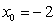 .
.
(Multiple Choice)
4.8/5  (38)
(38)
Use a suitable Maclaurin Series to estimate 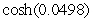 to five decimal place accuracy.
to five decimal place accuracy.
(Multiple Choice)
4.7/5  (25)
(25)
Determine whether or not the following series converges. If it converges, find its sum. 
(Multiple Choice)
4.7/5  (30)
(30)
Use a suitable Maclaurin Series to estimate  to five decimal place accuracy.
to five decimal place accuracy.
(Multiple Choice)
5.0/5  (30)
(30)
Determine whether or not the following series converges. If it converges, find its sum. 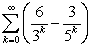
(Short Answer)
4.8/5  (38)
(38)
Determine whether or not the following series converges. Enter 'Yes' if the series converges, and 'No' if it diverges. 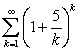
(Short Answer)
4.8/5  (37)
(37)
Classify the following series as absolutely convergent, conditionally convergent, or divergent. 
(Short Answer)
4.8/5  (31)
(31)
Determine whether or not the following series converges. If it converges, find its sum. 
(Multiple Choice)
4.7/5  (33)
(33)
Find the first three nonzero terms of the Maclaurin series for the function 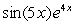 by multiplying the Maclaurin series for the factors.
by multiplying the Maclaurin series for the factors.
(Multiple Choice)
4.9/5  (38)
(38)
Showing 1 - 20 of 288
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)