Exam 15: Topics in Vector Calculus
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
Evaluate 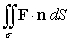 where F(x, y, z) = 18 i - 2z j + 2y k and is that portion of the paraboloid x = 4 - y2 - z2 to the right of x = 0 oriented by forward unit normals.
where F(x, y, z) = 18 i - 2z j + 2y k and is that portion of the paraboloid x = 4 - y2 - z2 to the right of x = 0 oriented by forward unit normals.
Free
(Multiple Choice)
4.8/5  (33)
(33)
Correct Answer:
E
Verify Stokes' Theorem if is the portion of the sphere x2 + y2 + z2 = 1 for which z 0 and F(x, y, z) = (2x - y)i - yz2 j - y2z k.
Free
(Essay)
4.9/5  (27)
(27)
Correct Answer:
If is oriented by upward normals, then C is the intersection of the sphere x2 + y2 + z2 = 1 with z = 0, thus, C is the circle x2 + y2 = 1 which can be parameterized as r(t) = cos t i + sin t j for 0 t 2 , so, 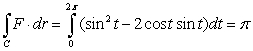 ;Curl F = k,
;Curl F = k, 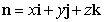 , and R is the circular region in the xy-plane enclosed by C, so
, and R is the circular region in the xy-plane enclosed by C, so 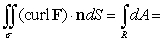 area of the circle of radius 1 = (1)2 = .
area of the circle of radius 1 = (1)2 = .
Evaluate the surface integral 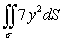 where is that portion of the plane x + y + z = 1 that lies in the first octant.
where is that portion of the plane x + y + z = 1 that lies in the first octant.
Free
(Essay)
4.8/5  (34)
(34)
Correct Answer:
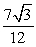
Find the flux of the vector field F(x, y, z) = 5z k across the sphere 5x2 + 5y2 + 5z2 = 20 oriented outward.
(Multiple Choice)
4.7/5  (34)
(34)
Evaluate C 2x2y dx + 2xy dy, where C is the triangle with vertices (0, 0), (1, 0), and (1, 3).
(Multiple Choice)
4.8/5  (38)
(38)
The work done by the force F(x, y) = 6x2 i + 12y2 j acting on a particle that moves along the circle x = cos t, y = sin t, from (1, 0) to (0, 1).
(Multiple Choice)
4.8/5  (27)
(27)
For F(x, y) = 2i + 2j the work done by the force field on a particle moving along an arbitrary smooth curve from P(0, 0) to Q(8, 5) is
(Multiple Choice)
4.9/5  (34)
(34)
Is F(x, y) = 2cos x i + 2cos y j is a conservative vector field?
(Short Answer)
4.8/5  (33)
(33)
Use Green's Theorem to evaluate C 10(2xy - y2)dx + 10x2dy where C is the boundary of the region enclosed by y = x + 1 and y = x2 + 1, traversed in a counterclockwise manner.
(Short Answer)
4.8/5  (38)
(38)
Use Green's Theorem to evaluate C 4(y - sin x)dx + 4cos x dy where C is the boundary of the region with vertices (0, 0),  , and
, and 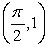 traversed in a counterclockwise manner.
traversed in a counterclockwise manner.
(Essay)
4.8/5  (42)
(42)
Evaluate 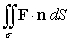 where F(x, y, z) = 4y i + 8x j + 4xy k and is that portion of the cylinder x2 + y2 = 9 in the first octant between z = 1 and z = 4. The surface is oriented by right unit normals.
where F(x, y, z) = 4y i + 8x j + 4xy k and is that portion of the cylinder x2 + y2 = 9 in the first octant between z = 1 and z = 4. The surface is oriented by right unit normals.
(Multiple Choice)
4.9/5  (25)
(25)
Evaluate 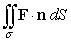 where F(x, y, z) = 12x i + 24j + 24x2 k and is that portion of the paraboloid z = x2 + y2 which lies above the xy-plane enclosed by the parabolas y = 1 - x2 and y = x2 - 1. The surface is oriented by downward unit normals.
where F(x, y, z) = 12x i + 24j + 24x2 k and is that portion of the paraboloid z = x2 + y2 which lies above the xy-plane enclosed by the parabolas y = 1 - x2 and y = x2 - 1. The surface is oriented by downward unit normals.
(Short Answer)
4.9/5  (33)
(33)
Is F(x, y) = 12cos y i + 12cos x j is a conservative vector field?
(Short Answer)
4.8/5  (33)
(33)
F (x, y, z) = 5xyz i + 5xyz j + 5xyz k. Find the outward flux of the vector field F across the unit cube in the first octant and including the origin as a vertex.
(Essay)
4.8/5  (27)
(27)
Evaluate the line integral. 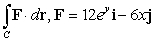 along y = ln x from the point (1, 0) to the point (3, ln 3).
along y = ln x from the point (1, 0) to the point (3, ln 3).
(Short Answer)
4.8/5  (37)
(37)
Determine whether F(x, y) = 4(3 cos y + 2 sin x)i + 4(3y2 - 3x sin y)j is conservative. If it is, find a potential function for it.
(Essay)
4.9/5  (34)
(34)
Showing 1 - 20 of 149
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)