Question 1

Determine how to minimize the company's total cost during the next four quarters.

Accepted Answer

The answer of Determine how to minimize the company's total...

Question 2

The flow balance constraint for each transshipment node,in a minimum cost network flow model,takes which form?

Accepted Answer

A) Net Outflow = Capacity 
B) Net Outflow < Capacity 
C) Net Inflow=0 (equivalent to Net Outflow=0) 
D) Net Inflow ≥ Demand 
A) Net Outflow = Capacity 
B) Net Outflow < Capacity 
C) Net Inflow=0 (equivalent to Net Outflow=0) 
D) Net Inflow ≥ Demand

Question 3

The flows in a general minimum cost network flow model (MCNFM)all have to be from "left to right"; that is,from supply points to demand points.

Accepted Answer

A) True 
 B)False

Question 4

During the next four quarters,an automobile company must meet (on time)the following demands for cars: 4000 in quarter 1; 2000 in quarter 2; 5000 in quarter 3; 1000 in quarter 4.At the beginning of quarter 1,there are 300 autos in stock.The company has the capacity to produce at most 3000 cars per quarter.At the beginning of each quarter,the company can change production capacity.It costs $100 to increase quarterly production capacity by 1 unit.For example,it would cost $20,000 to increase capacity from 3000 to 3200.It also costs $60 per quarter to maintain each unit of production capacity (even if it is unused during the current quarter).The variable cost of producing a car is $2200.A holding cost of $160 per car is assessed against each quarter's ending inventory.It is required that at the end of quarter 4,plant capacity must be at least 4000 cars.Determine how to minimize the total cost incurred during the next 4 quarters.

Accepted Answer

The answer of During the next four quarters,an automobile company...

Question 5

Transportation costs for a given route are nearly always linear and smooth,with the main cost differences occurring between routes.

Accepted Answer

A) True 
 B)False

Question 6

You should always look at the Solver solution for signs of implausibility.This can often help you spot an error in your model.

Accepted Answer

A) True 
 B)False

Question 7

Many organizations must determine how to schedule employees to provide adequate service.If we assume that an organization faces the same situation each week,this is referred to as

Accepted Answer

A) a static scheduling problem. 
B) a dynamic scheduling problem. 
C) a transportation scheduling problem. 
D) all of these choices. 
A) a static scheduling problem. 
B) a dynamic scheduling problem. 
C) a transportation scheduling problem. 
D) all of these choices.

Question 8

Your parents are discussing their retirement portfolio with you so that you can be informed not only about their holdings,but learn how to manage your own.The risk index of an investment can be obtained by taking the absolute values of percentage changes in the value of the investment for each year and averaging them.Suppose your dad asks you to determine what percentage of his money he should have invested in T-bills,gold,and stocks in the 1980s and 1990s based on data he provides for the years 1968-1988.The table below lists the annual returns (percentage changes in value)for these investments during these years. 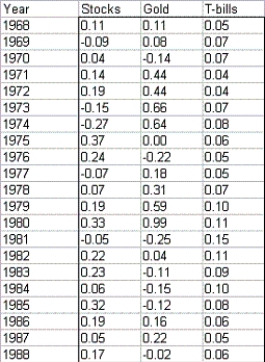  Let the risk index of a portfolio be the weighted average of the risk indexes of these investments,where the weights are the fractions of your parents' money assigned to the investments.Suppose that the amount of each investment must be between 20% and 50% of the total invested.You would like the risk index of your portfolio to equal 0.15,and your goal is to maximize the expected return on your portfolio.Determine the maximum expected return on their portfolio,subject to the stated constraints.Use the average return earned by each investment during the years 1968-1988 as their estimate of expected return.

Accepted Answer

The answer of Your parents are discussing their retirement portfolio...

Question 9

When we solve a linear programming problem with Solver,we cannot guarantee that the solution obtained is an optimal solution.

Accepted Answer

A) True 
 B)False

Question 10

The transportation model is a special case of the minimum cost network flow model (MCNFM).

Accepted Answer

A) True 
 B)False

Question 11

A product can be produced on four different machines.Each machine has a fixed setup cost,variable production cost per unit processed,and a production capacity,as shown below: 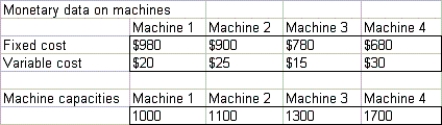  A total of 2000 units of the product must be produced.What is the minimum total cost that will meet these constraints? How many units must each machine produce?

Accepted Answer

The answer of A product can be produced on four...

Question 12

In an optimized network flow model (MCNFM),all the available capacity will be used.

Accepted Answer

A) True 
 B)False

Question 13

Any integer programming problem involving 0-1 variables with only one constraint is called a knapsack problem.

Accepted Answer

A) True 
 B)False

Question 14

Which of the following statements are false?

Accepted Answer

A) Solver does not offer a sensitivity report for models with integer constraints. 
B) Solver's sensitivity report is not suited for questions about multiple input changes. 
C) Solver's sensitivity report is used primarily for questions about one-at-a time changes to input. 
D) None of these statements are false. 
A) Solver does not offer a sensitivity report for models with integer constraints. 
B) Solver's sensitivity report is not suited for questions about multiple input changes. 
C) Solver's sensitivity report is used primarily for questions about one-at-a time changes to input. 
D) None of these statements are false.

Question 15

In all optimization models,the objective cell has to be a function of the decision variable cells.It is perfectly consistent with this requirement to have the _____ be one of the _____.

Accepted Answer

A) range name,optimization models 
B) input range,output cells 
C) decision variable cell,objective cells 
D) objective cell,decision variable cells 
A) range name,optimization models 
B) input range,output cells 
C) decision variable cell,objective cells 
D) objective cell,decision variable cells

Question 16

Which of these statements are true of multiple optimal solutions?

Accepted Answer

A) All solutions have the same values for the decision variables. 
B) All solutions have the same value for the objective function. 
C) All solutions have the same shadow prices. 
D) All of these statements are true. 
A) All solutions have the same values for the decision variables. 
B) All solutions have the same value for the objective function. 
C) All solutions have the same shadow prices. 
D) All of these statements are true.

Question 17

In a set-covering model,each member of a given set (set 1)must be "covered" by an acceptable member of another set (set 2).The objective of such problems is to minimize the number of elements in set 2 that are needed to cover all the elements in set 1.

Accepted Answer

A) True 
 B)False

Question 18

Workforce scheduling problems are often integer programming models,which means that they have

Accepted Answer

A) an integer objective function. 
B) integer decision variables. 
C) integer constraints. 
D) integer output. 
A) an integer objective function. 
B) integer decision variables. 
C) integer constraints. 
D) integer output.

Question 19

Linear programming models are used by many financial firms to select a desirable bond portfolio.The following is a simplified version of such a model.Abby is considering investing in four bonds; $1.5 million is available for investment.The expected annual return,the worst-case annual return on each bond,and the "duration" of each bond are given below (The duration of a bond is a measure of the bond's sensitivity to interest rates.)   Abby wants to maximize the expected return from her bond investments,subject to the following three constraints:
∙ The worst-case return of the bond portfolio must be at least 8%.
∙ The average duration of the portfolio must be at most 6.For example,a portfolio that invests $600,000 in bond 1 and $400,000 in bond 4 has an average duration of [600,000(3)+ 400,000 (9)]/1,000,000 = 5.4.
∙ Because of diversification requirements,at most 40% of the total amount invested can be invested in a single bond.
Determine how Abby can maximize the expected return on her investment.

Accepted Answer

The answer of Linear programming models are used by many...

Question 20

A knapsack problem is any integer program involving 0 - 1 variable with _____ constraints.

Accepted Answer

A) three 
B) two 
C) one 
D) zero 
A) three 
B) two 
C) one 
D) zero

Determine how to minimize the company's total cost during the next four quarters.

The flow balance constraint for each transshipment node,in a minimum cost network flow model,takes which form?

The flows in a general minimum cost network flow model (MCNFM)all have to be from "left to right"; that is,from supply points to demand points.

Transportation costs for a given route are nearly always linear and smooth,with the main cost differences occurring between routes.

You should always look at the Solver solution for signs of implausibility.This can often help you spot an error in your model.

Many organizations must determine how to schedule employees to provide adequate service.If we assume that an organization faces the same situation each week,this is referred to as

When we solve a linear programming problem with Solver,we cannot guarantee that the solution obtained is an optimal solution.

The transportation model is a special case of the minimum cost network flow model (MCNFM).

In an optimized network flow model (MCNFM),all the available capacity will be used.

Any integer programming problem involving 0-1 variables with only one constraint is called a knapsack problem.

Which of the following statements are false?

In all optimization models,the objective cell has to be a function of the decision variable cells.It is perfectly consistent with this requirement to have the _ be one of the _.

Which of these statements are true of multiple optimal solutions?

In a set-covering model,each member of a given set (set 1)must be "covered" by an acceptable member of another set (set 2).The objective of such problems is to minimize the number of elements in set 2 that are needed to cover all the elements in set 1.

Workforce scheduling problems are often integer programming models,which means that they have

A knapsack problem is any integer program involving 0 - 1 variable with _____ constraints.

Introduction to Business Analytics

Describing the Distribution of a Variable

Finding Relationships Among Variables

Business Intelligence Bifor Data Analysis

Probability and Probability Distributions

Decision Making Under Uncertainty

Sampling and Sampling Distributions

Confidence Interval Estimation

Hypothesis Testing

Regression Analysis: Estimating Relationships

Regression Analysis: Statistical Inference

Time Series Analysis and Forecasting

Introduction to Optimization Modeling

Introduction to Simulation Modeling

Simulation Models

Data Mining

Analysis of Variance and Experimental Design

Statistical Process Control

Filters

Exam 14: Optimization Models

Determine how to minimize the company's total cost during the next four quarters.

The flow balance constraint for each transshipment node,in a minimum cost network flow model,takes which form?

The flows in a general minimum cost network flow model (MCNFM)all have to be from "left to right"; that is,from supply points to demand points.

Transportation costs for a given route are nearly always linear and smooth,with the main cost differences occurring between routes.

You should always look at the Solver solution for signs of implausibility.This can often help you spot an error in your model.

Many organizations must determine how to schedule employees to provide adequate service.If we assume that an organization faces the same situation each week,this is referred to as

When we solve a linear programming problem with Solver,we cannot guarantee that the solution obtained is an optimal solution.

The transportation model is a special case of the minimum cost network flow model (MCNFM).

In an optimized network flow model (MCNFM),all the available capacity will be used.

Any integer programming problem involving 0-1 variables with only one constraint is called a knapsack problem.

Which of the following statements are false?

In all optimization models,the objective cell has to be a function of the decision variable cells.It is perfectly consistent with this requirement to have the _____ be one of the _____.

Which of these statements are true of multiple optimal solutions?

In a set-covering model,each member of a given set (set 1)must be "covered" by an acceptable member of another set (set 2).The objective of such problems is to minimize the number of elements in set 2 that are needed to cover all the elements in set 1.

Workforce scheduling problems are often integer programming models,which means that they have

A knapsack problem is any integer program involving 0 - 1 variable with _____ constraints.

Introduction to Business Analytics

Describing the Distribution of a Variable

Finding Relationships Among Variables

Business Intelligence Bifor Data Analysis

Probability and Probability Distributions

Decision Making Under Uncertainty

Sampling and Sampling Distributions

Confidence Interval Estimation

Hypothesis Testing

Regression Analysis: Estimating Relationships

Regression Analysis: Statistical Inference

Time Series Analysis and Forecasting

Introduction to Optimization Modeling

Introduction to Simulation Modeling

Simulation Models

Data Mining

Analysis of Variance and Experimental Design

Statistical Process Control

Filters

In all optimization models,the objective cell has to be a function of the decision variable cells.It is perfectly consistent with this requirement to have the _ be one of the _.