Exam 11: Rolling, Torque, and Angular Momentum
Exam 1: Measurement37 Questions
Exam 2: Motion Along a Straight Line90 Questions
Exam 3: Vector32 Questions
Exam 4: Motion in Two and Three Dimensions53 Questions
Exam 5: Force and Motion I73 Questions
Exam 6: Force and Motion II74 Questions
Exam 7: Kinetic Energy and Work72 Questions
Exam 8: Potential Energy and Conservation of Energy62 Questions
Exam 9: Center of Mass and Linear Momentum98 Questions
Exam 10: Rotation99 Questions
Exam 11: Rolling, Torque, and Angular Momentum65 Questions
Exam 12: Equilibrium and Elasticity57 Questions
Exam 13: Gravitation54 Questions
Exam 14: Fluids87 Questions
Exam 15: Oscillations75 Questions
Exam 16: Waves I80 Questions
Exam 17: Waves II70 Questions
Exam 18: Temperature, Heat, and the First Law of Thermodynamics96 Questions
Exam 19: The Kinetic Theory of Gases111 Questions
Exam 20: Entropy and the Second Law of Thermodynamics61 Questions
Exam 21: Electric Charge51 Questions
Exam 22: Electric Fields52 Questions
Exam 23: Gauss Law39 Questions
Exam 24: Electric Potential50 Questions
Exam 25: Capacitance59 Questions
Exam 26: Current and Resistance54 Questions
Exam 27: Circuits73 Questions
Exam 28: Magnetic Fields51 Questions
Exam 29: Magnetic Fields Due to Currents48 Questions
Exam 30: Induction and Inductance90 Questions
Exam 31: Electromagnetic Oscillations and Alternating Current86 Questions
Exam 32: Maxwells Equations; Magnetism of Matter81 Questions
Exam 33: Electromagnetic Waves81 Questions
Exam 34: Images78 Questions
Exam 35: Interference45 Questions
Exam 36: Diffraction77 Questions
Exam 37: Relativity68 Questions
Exam 38: Photons and Matter Waves57 Questions
Exam 39: More About Matter Waves41 Questions
Exam 40: All About Atoms76 Questions
Exam 41: Conduction of Electricity in Solids49 Questions
Exam 42: Nuclear Physics68 Questions
Exam 43: Energy From the Nucleus50 Questions
Exam 44: Quarks, Leptons, and the Big Bang55 Questions
Select questions type
A particle is located on the x axis at x = 2.0 m from the origin.A force of 25 N, directed 30° above the x axis in the x-y plane, acts on the particle.What is the torque about the origin on the particle?
Free
(Multiple Choice)
4.8/5  (30)
(30)
Correct Answer:
B
Which of the following is a vector quantity?
Free
(Multiple Choice)
4.8/5  (41)
(41)
Correct Answer:
E
When we apply the energy conversation principle to a cylinder rolling down an incline without sliding, we exclude the work done by friction because:
Free
(Multiple Choice)
4.8/5  (41)
(41)
Correct Answer:
D
A particle moves along the x axis.In order to calculate the angular momentum of the particle, you need to know:
(Multiple Choice)
4.9/5  (35)
(35)
A forward force acting on the axle accelerates a rolling wheel on a horizontal surface.If the wheel does not slide the frictional force of the surface on the wheel is:
(Multiple Choice)
4.9/5  (34)
(34)
A cylinder of radius R = 6.0 cm is on a rough horizontal surface.The coefficient of kinetic friction between the cylinder and the surface is 0.30 and the rotational inertia for rotation about the axis is given by MR2/2, where M is its mass.Initially it is not rotating but its center of mass has a speed of 7.0 m/s.After 2.0 s the speed of its center of mass and its angular velocity about its center of mass, respectively, are:
(Multiple Choice)
4.9/5  (34)
(34)
A particle moves along the x axis.In order to calculate the torque on the particle, you need to know:
(Multiple Choice)
4.7/5  (37)
(37)
A hoop (I = MR2)of mass 2.0 kg and radius 0.50 m is rolling at a center-of-mass speed of 15 m/s.An external force does 750 J of work on the hoop.What is the new speed of the center of mass of the hoop?
(Multiple Choice)
4.8/5  (38)
(38)
A 2.0-kg block starts from rest on the positive x axis 3.0 m from the origin and thereafter has an acceleration given by in m/s2.The torque, relative to the origin, acting on it at the end of 2.0 s is:
(Multiple Choice)
4.8/5  (42)
(42)
A solid wheel with mass M, radius R, and rotational inertia MR2/2, rolls without sliding on a horizontal surface.A horizontal force F is applied to the axle and the center of mass has an acceleration a.The magnitudes of the applied force F and the frictional force f of the surface, respectively, are:
(Multiple Choice)
4.7/5  (31)
(31)
A hoop, a uniform disk, and a uniform sphere, all with the same mass and outer radius, start with the same speed and roll without sliding up identical inclines.Rank the objects according to how high they go, least to greatest.
(Multiple Choice)
4.8/5  (39)
(39)
When a woman on a frictionless rotating turntable extends her arms out horizontally, her angular momentum:
(Multiple Choice)
4.8/5  (37)
(37)
A single force acts on a particle P.Rank each of the orientations of the force shown below according to the magnitude of the time rate of change of the particle's angular momentum about the point O, least to greatest. 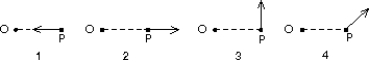
(Multiple Choice)
5.0/5  (32)
(32)
A man, holding a weight in each hand, stands at the center of a horizontal frictionless rotating turntable.The effect of the weights is to double the rotational inertia of the system.As he is rotating, the man opens his hands and drops the two weights.They fall outside the turntable.Then:
(Multiple Choice)
4.9/5  (32)
(32)
A 2.0-kg stone is tied to a 0.50-m long string and swung around a circle at a constant angular velocity of 12 rad/s.The net torque on the stone about the center of the circle is:
(Multiple Choice)
4.7/5  (41)
(41)
A 5.0-kg ball rolls without sliding from rest down an inclined plane.A 4.0-kg block, mounted on roller bearings totaling 100 g, rolls from rest down the same plane.At the bottom, the block has:
(Multiple Choice)
4.8/5  (33)
(33)
A phonograph record is dropped onto a freely spinning turntable.Then:
(Multiple Choice)
4.8/5  (31)
(31)
Two objects are moving in the x, y plane as shown.If a net torque of 44 N∙m acts on them for 5.0 seconds, what is the change in their angular momentum? 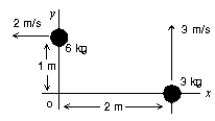
(Multiple Choice)
4.9/5  (38)
(38)
A uniform disk has radius R and mass M.When it is spinning with angular velocity about an axis through its center and perpendicular to its face its angular momentum is I .When it is spinning with the same angle velocity about a parallel axis a distance h away its angular momentum is:
(Multiple Choice)
4.9/5  (32)
(32)
Showing 1 - 20 of 65
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)