Exam 5: Discrete Probability Distributions

SCENARIO 5-9 A major hotel chain keeps a record of the number of mishandled bags per 1,000 customers.In a recent year,the hotel chain had 4.06 mishandled bags per 1,000 customers.Assume that the number of mishandled bags has a Poisson distribution. -Referring to Scenario 5-9,what is the probability that in the next 1,000 customer,the hotel chain will have no more than three mishandled bags?

Suppose that history shows that 60% of college students prefer Brand C cola.A sample of 5 students is to be selected.The variance of the number that prefer brand C is_____ .

Another name for the mean of a probability distribution is its expected value.

In a game called Taxation and Evasion,a player rolls a pair of dice.If on any turn the sum is 7,11,or 12,the player gets audited.Otherwise,she avoids taxes.Suppose a player takes 5 turns at rolling the dice.The probability that she gets audited no more than 2 times is _____.

SCENARIO 5-4 The following table contains the probability distribution for X = the number of traffic accidents reported in a day in a small city in the Midwest. 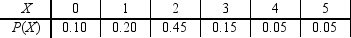-Referring to Scenario 5-4,the mean or expected value of the number of accidents is _____.

SCENARIO 5-9 A major hotel chain keeps a record of the number of mishandled bags per 1,000 customers.In a recent year,the hotel chain had 4.06 mishandled bags per 1,000 customers.Assume that the number of mishandled bags has a Poisson distribution. -Referring to Scenario 5-9,what is the probability that in the next 1,000 customers,the hotel chain will have more than ten mishandled bags?

Whenever $\pi$ = 0.1 and n is small,the binomial distribution will be

SCENARIO 5-8 Two different designs on a new line of winter jackets for the coming winter are available for your manufacturing plants.Your profit (in thousands of dollars)will depend on the taste of the consumers when winter arrives.The probability of the three possible different tastes of the consumers and the corresponding profits are presented in the following table. 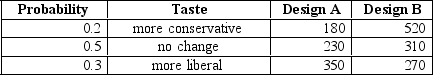-Referring to Scenario 5-8,what is your expected profit when Design B is chosen?

If $\pi$ remains constant in a binomial distribution,an increase in n will not change the mean.

SCENARIO 5-9 A major hotel chain keeps a record of the number of mishandled bags per 1,000 customers.In a recent year,the hotel chain had 4.06 mishandled bags per 1,000 customers.Assume that the number of mishandled bags has a Poisson distribution. -Referring to Scenario 5-9,what is the probability that in the next 1,000 customers,the hotel chain will have less than two or more than eight mishandled bags?

SCENARIO 5-8 Two different designs on a new line of winter jackets for the coming winter are available for your manufacturing plants.Your profit (in thousands of dollars)will depend on the taste of the consumers when winter arrives.The probability of the three possible different tastes of the consumers and the corresponding profits are presented in the following table. -Referring to Scenario 5-8,if your investment preference is to maximize your expected profit while exposing yourself to the minimal amount of risk,will you choose a production mix that will consist of 10%,30%,50%,70%,or 90% of your production lines for Design A and the remaining for Design B?

SCENARIO 5-9 A major hotel chain keeps a record of the number of mishandled bags per 1,000 customers.In a recent year,the hotel chain had 4.06 mishandled bags per 1,000 customers.Assume that the number of mishandled bags has a Poisson distribution. -Referring to Scenario 5-9,what is the probability that in the next 1,000 customers,the hotel chain will have between two and four inclusive mishandled bags?

SCENARIO 5-8 Two different designs on a new line of winter jackets for the coming winter are available for your manufacturing plants.Your profit (in thousands of dollars)will depend on the taste of the consumers when winter arrives.The probability of the three possible different tastes of the consumers and the corresponding profits are presented in the following table. -Referring to Scenario 5-8,what is your expected profit when Design A is chosen?

In a Poisson distribution,the mean and variance are equal.

SCENARIO 5-7 There are two houses with almost identical characteristics available for investment in two different neighborhoods with drastically different demographic composition.The anticipated gain in value when the houses are sold in 10 years has the following probability distribution: 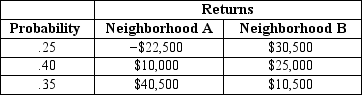-Referring to Scenario 5-7,if you can invest 10% of your money on the house in neighborhood A and the remaining on the house in neighborhood B,what is the portfolio risk of your investment?

In a game called Taxation and Evasion,a player rolls a pair of dice.If on any turn the sum is 7,11,or 12,the player gets audited.Otherwise,she avoids taxes. Suppose a player takes 5 turns at rolling the dice.The probability that she gets audited once is .