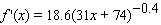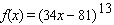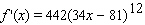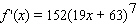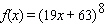Exam 4: Techniques of Differentiation With Applications
Exam 1: Functions and Linear Models100 Questions
Exam 2: Nonlinear Functions and Models88 Questions
Exam 3: Introduction to the Derivative140 Questions
Exam 4: Techniques of Differentiation With Applications106 Questions
Exam 5: Further Applications of the Derivative85 Questions
Exam 6: The Integral71 Questions
Exam 7: Further Integration Techniques and Applications of the Integral117 Questions
Exam 8: Functions of Several Variables133 Questions
Exam 9: Trigonometric Models66 Questions
Select questions type
Find the equation of the straight line, tangent to 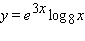 at the point (1, 0).
at the point (1, 0).
(Multiple Choice)
4.8/5  (35)
(35)
Your Porche s gas mileage (in miles per gallon)is given as a function 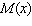 of speed
of speed 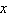 in miles per hour.
in miles per hour. 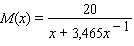 Calculate
Calculate 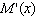 .
.
(Multiple Choice)
4.9/5  (41)
(41)
The cost of producing x teddy bears per day at the Cuddly Companion Company is calculated by their marketing staff to be given by the formula 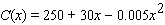 . Evaluate the average cost
. Evaluate the average cost 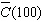 .
.
(Multiple Choice)
4.8/5  (33)
(33)
Your company is planning to air a number of television commercials during the ABC television network s presentation of the Academy Awards. ABC is charging your company $795,000 per 30-second spot. Additional fixed costs (development and personnel costs)amount to $400,000, and the network has agreed to provide a discount of 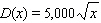 for x television spots. Compute marginal cost
for x television spots. Compute marginal cost 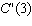 and average cost
and average cost 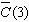 .
.
(Multiple Choice)
4.9/5  (37)
(37)
The oxygen consumption of a bird embryo increases from the time the egg is laid through the time the chick hatches. In a typical galliform bird, the oxygen consumption (in milliliters per hour)can be approximated by 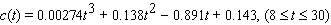 where t is the time (in days)since the egg was laid. (An egg will typically hatch at around
where t is the time (in days)since the egg was laid. (An egg will typically hatch at around 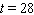 .)Find
.)Find 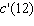 .
.
(Multiple Choice)
4.8/5  (38)
(38)
The Thoroughbred Bus Company finds that its monthly costs for one particular year were given by 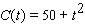 dollars after
dollars after 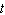 months. After
months. After 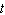 months, the company had
months, the company had  passengers per month. How fast was its cost per passenger changing after
passengers per month. How fast was its cost per passenger changing after  months?
months?
(Multiple Choice)
4.9/5  (38)
(38)
Given. 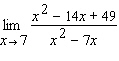 Say whether L Hospital s rule applies. It is does, use it to evaluate the given limit. If not, use some other method.
Say whether L Hospital s rule applies. It is does, use it to evaluate the given limit. If not, use some other method.
(Multiple Choice)
4.9/5  (32)
(32)
Calculate the derivatives of the functions. Match each function with the corresponding derivative.
Correct Answer:
Premises:
Responses:
(Matching)
5.0/5  (34)
(34)
Calculate the derivative of the function.  Please enter your answer as an expression.
Please enter your answer as an expression.
(Essay)
4.8/5  (40)
(40)
The cost of controlling emissions at a firm goes up rapidly as the amount of emissions reduced goes up. Here is a possible model: 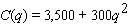 where q is the reduction in emissions (in pounds of pollutant per day)and C is the daily cost (in dollars)of this reduction. Government clean-air subsidies to the firm are based on the formula
where q is the reduction in emissions (in pounds of pollutant per day)and C is the daily cost (in dollars)of this reduction. Government clean-air subsidies to the firm are based on the formula 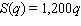 where q is again the reduction in emissions (in pounds per day)and S is the subsidy (in dollars). Calculate the net cost function
where q is again the reduction in emissions (in pounds per day)and S is the subsidy (in dollars). Calculate the net cost function  given the cost and subsidy above, and find the value of q that gives the lowest net cost. What is this lowest net cost?
given the cost and subsidy above, and find the value of q that gives the lowest net cost. What is this lowest net cost?
(Multiple Choice)
4.9/5  (34)
(34)
The cost of controlling emissions at a firm goes up rapidly as the amount of emissions reduced goes up. Here is a possible model:  where q is the reduction in emissions (in pounds of pollutant per day)and C is the daily cost (in dollars)of this reduction. If a firm is currently reducing its emissions by 7 pounds each day, what is the marginal cost of reducing emissions even further?
where q is the reduction in emissions (in pounds of pollutant per day)and C is the daily cost (in dollars)of this reduction. If a firm is currently reducing its emissions by 7 pounds each day, what is the marginal cost of reducing emissions even further?
(Multiple Choice)
4.7/5  (33)
(33)
If a stone is dropped from a height of 300 feet, its height s after t seconds is given by 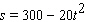 with s in feet. a. Find the stone s velocity at
with s in feet. a. Find the stone s velocity at 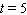 seconds. b. When does it reach the ground, and how fast is it travelling when it hits the ground? Round your answers to the nearest hundredth.
seconds. b. When does it reach the ground, and how fast is it travelling when it hits the ground? Round your answers to the nearest hundredth.
(Multiple Choice)
4.7/5  (32)
(32)
Showing 81 - 100 of 106
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)