Exam 8: Orthogonality
Exam 1: Systems of Linear Equations57 Questions
Exam 2: Euclidean Space48 Questions
Exam 3: Matrices76 Questions
Exam 4: Subspaces60 Questions
Exam 5: Determinants48 Questions
Exam 6: Eigenvalues and Eigenvectors75 Questions
Exam 7: Vector Spaces45 Questions
Exam 8: Orthogonality75 Questions
Exam 9: Linear Transformations60 Questions
Exam 10: Inner Product Spaces45 Questions
Exam 11: Additional Topics and Applications75 Questions
Select questions type
If S is a nonzero subspace of  , then for every vector u in
, then for every vector u in 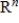 ,
, 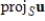 belongs to S.
belongs to S.
Free
(True/False)
4.7/5  (36)
(36)
Correct Answer:
True
If 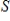 is a nonzero subspace of
is a nonzero subspace of 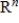 , and u belongs to
, and u belongs to 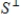 , then
, then 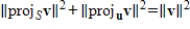 for every vector
for every vector  .
.
Free
(True/False)
4.8/5  (37)
(37)
Correct Answer:
False
If  is an invertible (square) matrix with singular value decomposition
is an invertible (square) matrix with singular value decomposition 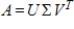 , then a singular value decomposition for
, then a singular value decomposition for 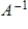 is given by
is given by 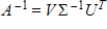 .
.
Free
(True/False)
4.9/5  (29)
(29)
Correct Answer:
False
If a system of equations has more equations than variables, then the system has a unique least squares solution.
(True/False)
4.9/5  (39)
(39)
Determine 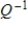 from the given matrix with orthogonal columns without using row operations.
from the given matrix with orthogonal columns without using row operations.
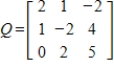
(Essay)
4.7/5  (31)
(31)
The eigenvalues and corresponding eigenvectors for a symmetric matrix A are given. Find matrices D and P of an orthogonal diagonalization of A. 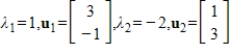
(Essay)
4.9/5  (41)
(41)
If A is an 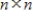 diagonalizable matrix, then there exists a diagonal matrix D and an orthogonal matrix P such that
diagonalizable matrix, then there exists a diagonal matrix D and an orthogonal matrix P such that 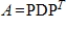 .
.
(True/False)
4.8/5  (40)
(40)
Showing 1 - 20 of 75
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)