Exam 4: Exponential Functions

A population is 100,000 in year t = 0 and declines at a continuous rate of 8% per year. What is the formula for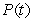 , the population in year t?

The price of an item increases due to inflation. Let give the price of the item as a function of time in years, with t = 0 in 2004. Estimate 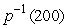to 2 decimal places.

The amount of pollution in a harbor t hours after it was contaminated by illegal dumping is given by 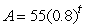tons. What percentage of the pollution leaves the harbor each hour?

The price of an item increases due to inflation. Let 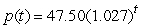give the price of the item as a function of time in years, with t = 0 in 2004. At what continuous annual rate is the price increasing? Round to 2 decimal places.

A biologist measures the amount of contaminant in a lake 5 hours after a chemical spill and again 8 hours after the spill. She sets up two possible models to determine Q, the amount of the chemical remaining in the lake as a function of t, the time in hours since the spill. The first model assumes the contaminant is leaving the lake at a constant rate, which she determines to be 3 tons/hour. Using this model, she estimates that the lake will be free from the contaminant 30 hours after the spill. Thus, 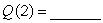and 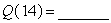. The second model assumes that the amount of contaminant decreases exponentially. In this model, she finds that 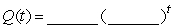. Round both answers to 3 decimal places.

In the following figure, the functions f, g, h, and p can all be written in the form . Which one has the smallest value for b?

The following table gives values from an exponential or a linear function. Determine which, and find values for a and b so that 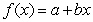if the function is linear, or 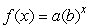if the function is exponential. a = ______, b = ______.

?

The US population in 2005 was approximately 296.4 million. Assume the population increases at a rate of 1.32% per year. What is the formula for 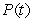, the population for the United States t years after 2005?

The populations of 4 species of animals are given by the following equations: 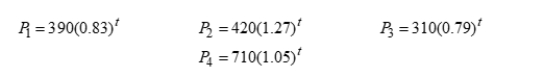Which species are growing in size?

Taylor has $12,000 which she would like to invest. She can either invest in a CD earning 3.3% annual interest compounded quarterly, or she can deposit the money in a savings account earning 3.25% annual interest compounded monthly. If she chooses the investment with the maximum return, how much interest will she have earned at the end of 5 years?

?

Which of the following formulas are exponential?

Use the formula to answer the following questions about the investment it describes. Units are dollars and years. A) Is the investment increasing or decreasing? B) What is the initial value of the investment? C) What is the effective annual rate of the account?

A biologist measures the amount of contaminant in a lake 2 hours after a chemical spill and again 11 hours after the spill. She sets up two possible models to determine Q, the amount of the chemical remaining in the lake as a function of t, the time in hours since the spill. The first model assumes the contaminant is leaving the lake at a constant rate, which she determines to be 3 tons/hour. Using this model, she estimates that the lake will be free from the contaminant 25 hours after the spill. The second model assumes that the amount of contaminant decreases exponentially. She measures the spill a third time after 18 hours and finds that 29 tons remain. Which model seems best?

What is the growth factor if a bacteria colony decreases by 27% each hour?

The formula for the exponential function f such that f(3) = 12 and f(15) = 14 is given by f(t) = _____ ( _____ )^t. Give both answers to 2 decimal places.

The number of books in a library tends to increase by the same percentage each year. Should a linear or an exponential function be used to model this scenario?

If you start with $2000, how much money will you have after a 15% increase followed by a 20% decrease?

A biologist measures the amount of contaminant in a lake 3 hours after a chemical spill and again 11 hours after the spill. She sets up a possible model to determine Q, the amount of the chemical remaining in the lake as a function of t, the time in hours since the spill. The model assumes the contaminant is leaving the lake at a constant rate, which she determines to be 6 tons/hour. She estimates that the lake will be free from the contaminant 30 hours after the spill. How many tons of the contaminant were in the lake at the 3 hour reading?