Exam 5: Growth and Decay: An Introduction to Exponential Functions
Exam 1: An Introduction to Data and Functions149 Questions
Exam 2: Rates of Change and Linear Functions215 Questions
Exam 3: When Lines Meet: Linear Systems81 Questions
Exam 4: The Laws of Exponents and Logarithms: Measuring the Universe201 Questions
Exam 5: Growth and Decay: An Introduction to Exponential Functions146 Questions
Exam 6: Logarithmic Links: Logarithmic and Exponential Functions108 Questions
Exam 7: Power Functions109 Questions
Exam 8: Quadratics and the Mathematics of Motion127 Questions
Exam 9: New Functions From Old137 Questions
Select questions type
You have $4,000 to invest for 4 years. Two investment options are presented to you: I). 5.5% interest compounded monthly, or II). 6.8% interest compounded annually.
A) Which option will give a better return?
B) How much more interest will be earned under this option?
(Round the answer to the nearest penny.)
(Short Answer)
4.8/5  (38)
(38)
Find the equation of the given function.
?  Give your answer in
Give your answer in 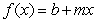 or
or 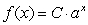 form. Round values to 2 decimal places.
form. Round values to 2 decimal places.
(Short Answer)
4.8/5  (45)
(45)
Complete the description for the exponential function, 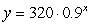 .
For every unit increase in the input, the output increases by ______ percent.
.
For every unit increase in the input, the output increases by ______ percent.
(Short Answer)
4.8/5  (32)
(32)
Alice invests $8,000 in an account offering 6% interest compounded continuously.
How many years until the account value reaches $10,000? Round your answer to the nearest year.
(Short Answer)
4.9/5  (36)
(36)
Determine the doubling time for the graph. Assume each marking on the x-axis represents one unit of time. Round the answer to 2 decimal places.
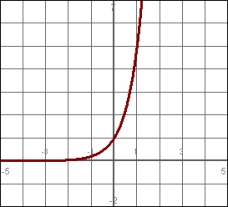
(Short Answer)
4.8/5  (33)
(33)
If the half-life of francium is 21 minutes, how long would it take for a 2,000 gram sample to decay to less than 60 grams? (Round the answer to the nearest minute.)
(Short Answer)
4.8/5  (37)
(37)
Determine the half-life of the graph. Assume each marking on the x-axis represents one unit of time. Round the answer to 2 decimal places.
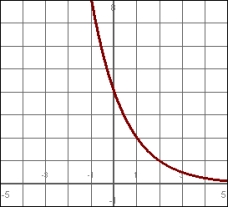
(Short Answer)
4.8/5  (36)
(36)
Consider:
F(x) = 93.8 - 0.77x
G(x) = 93.8 (0.77)x
Compare f(x) to g(x) as 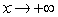 .
.
(Multiple Choice)
4.8/5  (29)
(29)
Below are functions that give the population, P, in year, t, for four towns.
Choose the function for the town that has the largest initial population.
(Multiple Choice)
4.8/5  (39)
(39)
An investment is originally worth $17,000 and its worth increases by a factor of 1.006 each month.
A) Find a formula that gives the investment's worth , W, as a function of the months, m, since the original investment.
B) Use the formula to find the worth of the investment after 16 months.Round your answer to the nearest cent.
(Essay)
4.7/5  (37)
(37)
Consider:
F(x) = 59.4 + 6.2x
G(x) = 55.7 (4.5)x
Compare f(x) to g(x) as 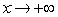 .
.
(Multiple Choice)
4.7/5  (40)
(40)
The function 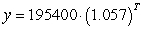 represents the growth of the deer population in Wisconsin where T represents 5-year intervals. Create an equivalent function where T represents 1-year intervals. Round the growth factor to 3 decimal places.
represents the growth of the deer population in Wisconsin where T represents 5-year intervals. Create an equivalent function where T represents 1-year intervals. Round the growth factor to 3 decimal places.
(Essay)
4.8/5  (38)
(38)
If $3,571 is invested at 2.9%, how much more would you have after 14 years if the interest was compounded monthly compared to compounded quarterly?
(Short Answer)
4.8/5  (37)
(37)
Given the functions:
F(x) = 119 (1.5)x
G(x) = 69 + 0.41x
H(x) = 82 (0.89)x
K(x) = 13.7 - 4.5x
As 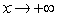 , which function(s) approach
, which function(s) approach 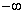 ? Select all that apply.
? Select all that apply.
(Multiple Choice)
4.8/5  (34)
(34)
Alice invests $5,000 in an account offering 2% interest compounded continuously.
Find the value of the account after 6 years. Round your answer to the nearest cent.
(Short Answer)
4.8/5  (28)
(28)
Showing 41 - 60 of 146
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)