Exam 9: One-Sample Tests of Hypothesis
Exam 1: What Is Statistics79 Questions
Exam 2: Describing Data: Frequency Tables, Frequency Distributions, and Graphic Presentation87 Questions
Exam 3: Describing Data: Numerical Measures191 Questions
Exam 4: A Survey of Probability Concepts130 Questions
Exam 5: Discrete Probability Distributions121 Questions
Exam 6: Continuous Probability Distributions143 Questions
Exam 7: Sampling Methods and the Central Limit Theorem78 Questions
Exam 8: Estimation and Confidence Intervals134 Questions
Exam 9: One-Sample Tests of Hypothesis139 Questions
Exam 10: Two-Sample Tests of Hypothesis103 Questions
Exam 11: Analysis of Variance97 Questions
Exam 12: Linear Regression and Correlation166 Questions
Exam 13: Multiple Regression and Correlation Analysis128 Questions
Exam 14: Chi-Square Applications126 Questions
Exam 15: Index Numbers93 Questions
Exam 16: Time Series and Forecasting90 Questions
Exam 17: An Introduction to Decision Theory54 Questions
Select questions type
What are the two rejection areas in using a two-tailed test and the 0.01 level of significance when the population standard deviation is known?
(Multiple Choice)
4.8/5  (45)
(45)
i. If the critical values of the test statistic z are 1.96, they are the dividing points between the areas of rejection and non-rejection. ii. The probability of a Type I error is also referred to as alpha.
iii. A Type I error is the probability of rejecting a true null hypothesis.
(Multiple Choice)
4.9/5  (39)
(39)
A manufacturer claims that less than 1% of all his products do not meet the minimum government standards. A survey of 500 products revealed ten did not meet the standard.
(Multiple Choice)
4.8/5  (37)
(37)
Based on the Nielsen ratings, the local CBS affiliate claims its 11:00 PM newscast reaches 41% of the viewing audience in the area. In a survey of 100 viewers, 36% indicated that they watch the late evening news on this local CBS station. What is the null hypothesis?
(Multiple Choice)
4.8/5  (31)
(31)
Which of the following does NOT hold true for the t distribution?
(Multiple Choice)
4.7/5  (34)
(34)
It is claimed that in a bushel of peaches less than ten percent are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the z-statistic?
(Multiple Choice)
4.8/5  (29)
(29)
The mean gross annual incomes of certified tack welders are normally distributed with the mean of $50,000 and a standard deviation of $4,000. The ship building association wishes to find out whether their tack welders earn more or less than $50,000 annually. The alternate hypothesis is that the mean is not $50,000. Which of the following is the alternate hypothesis?
(Multiple Choice)
4.9/5  (31)
(31)
i. If we do not reject the null hypothesis based on sample evidence, we have proven beyond doubt that the null hypothesis is true. ii. A test statistic is a value determined from sample information collected to test the null hypothesis.
iii. The region or area of rejection defines the location of all those values that are so large or so small that the probability of their occurrence under a true null hypothesis is rather remote.
(Multiple Choice)
4.8/5  (35)
(35)
The Jamestown Steel Company manufactures and assembles desks and other office equipment at several plants. The weekly production of the Model A325 desk follows a normal probability distribution, with a mean of 200 and a standard deviation of 16. Recently, due to market expansion, new production methods have been introduced and new employees hired. The vice president of manufacturing would like to investigate whether there has been a change in the weekly production of the Model A325 desk. The mean number of desks produced last year (50 weeks, because the plant was shut down two weeks for vacation) is 203.5. Is the mean number of desks produced different from 200? Test using the.01 significance level. i. The alternate hypothesis is 200.
ii. It is appropriate to use the z-test.
iii. The decision rule is: if the computed value of the test statistic is not between -2.58 and 2.58, reject the null hypothesis.
(Multiple Choice)
4.9/5  (44)
(44)
To conduct a test of hypothesis with a small sample, we need to be able to make the following assumption that:
(Multiple Choice)
4.8/5  (24)
(24)
The printout below refers to the weekly closing stock prices for Air Canada on 20 randomly selected weeks in 2000. Using a 5% level of significance, can you say that the average Air Canada stock price was less than $19.00?
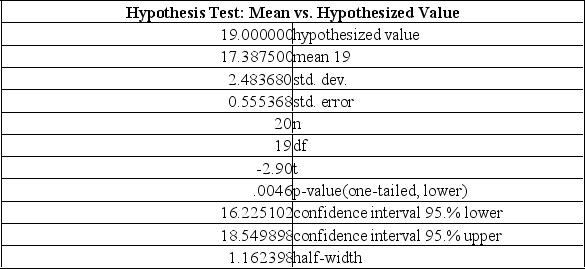
(Multiple Choice)
4.8/5  (36)
(36)
The mean weight of newborn infants at a community hospital is said to be 6.6 pounds. A sample of seven infants is randomly selected and their weights at birth are recorded as 9.0, 7.3, 6.0, 8.8, 6.8, 8.4, and 6.6 pounds. If = 0.05, what is the critical t value?
(Multiple Choice)
4.9/5  (35)
(35)
i. An alternate hypothesis is a statement about a population parameter that is accepted when the null hypothesis is rejected. ii. The level of significance is the risk we assume of rejecting the null hypothesis when it is actually true.
iii. There is only one level of significance that is applied to all studies involving sampling.
(Multiple Choice)
4.7/5  (33)
(33)
i. The probability of a Type I error is also referred to as alpha. ii. A Type I error is the probability of accepting a true null hypothesis.
iii. A Type I error is the probability of rejecting a true null hypothesis.
(Multiple Choice)
4.8/5  (32)
(32)
A restaurant that bills its house account monthly is concerned that the average monthly bill exceeds $200 per account. A random sample of twelve accounts is selected, resulting in a sample mean of $220 and a standard deviation of $12. The t-test is to be conducted at the 5% level of significance. The t-value is calculated to be 5.77. At the 0.01 level of significance, what is your decision?
(Multiple Choice)
4.8/5  (31)
(31)
i. Two examples of a hypothesis are: 1) mean monthly income from all sources for senior citizens is $841 and
2) twenty percent of juvenile offenders ultimately are caught and sentenced to prison.
ii. Since there is more variability in sample means computed from smaller samples, we have more confidence in the resulting estimates and are less apt to reject null hypothesis.
iii. The test statistic for a problem where the population standard deviation is unknown is the Student's t distribution.
(Multiple Choice)
4.9/5  (31)
(31)
i. Two examples of a hypothesis are: 1) mean monthly income from all sources for senior citizens is $841 and
2) twenty percent of juvenile offenders ultimately are caught and sentenced to prison.
ii. Hypothesis testing is a procedure based on sample evidence and probability theory to decide whether the hypothesis is a reasonable statement.
iii. Since there is more variability in sample means computed from smaller samples, we have more confidence in the resulting estimates and are less apt to reject null hypothesis.
(Multiple Choice)
4.8/5  (40)
(40)
What is the critical value for a one-tailed hypothesis test in which a null hypothesis is tested at the 5% level of significance based on a sample size of 25 and an unknown population standard deviation?
(Multiple Choice)
4.9/5  (35)
(35)
A random sample of size 15 is selected from a normal population. The population standard deviation is unknown. Assume that a two-tailed test at the 0.10 significance level is to be used. For what value of t will the null hypothesis be rejected?
(Multiple Choice)
4.8/5  (36)
(36)
Showing 21 - 40 of 139
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)