The Following Scatterplot Shows the Percentage of the Vote a Candidate

Multiple Choice

The following scatterplot shows the percentage of the vote a candidate received in the 2004 senatorial elections according to the voter's income level based on an exit poll of voters conducted by CNN. The income levels 1-8 correspond to the following income classes: $1 =$ Under $\$ 15,000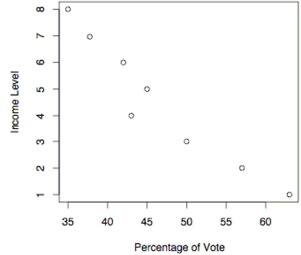 ; 2 = \$ 15 - 30,000 ; 3 = \$ 30 - 50,000 ; 4 = \$ 50 - 75,000 ; 5 = \$ 75 - 100,000 ; 6 = \$ 100 - 150,000$ ; $7 = \$ 150 - 200,000 ; 8 = \$ 200,000$ or more.


-Use the election scatterplot to determine whether there is a correlation between percentage of vote and income level at the 0.01 significance level with a null hypothesis of $\mathrm { Q } _ { \mathrm { s } } = 0 .$

A) The test statistic is between the critical values, so we fail to reject the null hypothesis . There is no evidence to support a claim of correlation between percentage of vote and income level.
B) The test statistic is between the critical values, so we reject the null hypothesis . There is sufficient evidence to support a claim of correlation between percentage of vote and income level.
C) The test statistic is not between the critical values, so we fail to reject the null hypothesis . There is no evidence to support a claim of correlation between percentage of vote and income level.
D) The test statistic is not between the critical values, so we reject the null hypothesis . There is sufficient evidence to support a claim of correlation between percentage of vote and income level.