Solved

If Is an Eigenvalue of an Invertible Matrix

Question 24

True/False

If If is an eigenvalue of an invertible matrix A, then is an eigenvalue of the matrix . is an eigenvalue of an invertible If 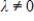is an eigenvalue of an invertible matrix A, then is an eigenvalue of the matrix . matrix A, then If is an eigenvalue of an invertible matrix A, then is an eigenvalue of the matrix . is an eigenvalue of the matrix If is an eigenvalue of an invertible 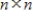matrix A, then 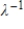is an eigenvalue of the matrix .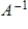
.

Correct Answer:

verifed

Verified

Unlock this answer now
Get Access to more Verified Answers free of charge

Related Questions

Q19: Determine the rotation and dilation that result

Q20: Determine which of <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="Determine which

Q21: The matrix <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="The matrix

Q22: Suppose that A is an <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg"

Q23: The Power Method applied to the matrix

Q25: Factor the matrix <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg" alt="Factor the

Q26: If A is diagonalizable, then <img src="https://d2lvgg3v3hfg70.cloudfront.net/TBMC1039/.jpg"

Q27: Find the characteristic polynomial, the eigenvalues, and

Q28: Diagonalize the matrix A, if possible.<br> <img

Q29: Compute the first two iterations of the