Researchers Investigated the Effects of Acute Stress on Emotional Picture

Multiple Choice

Researchers investigated the effects of acute stress on emotional picture processing and recall by randomly assigning 40 adult males to receive either a stressful cold stimulus or a neutral warm stimulus before viewing pictures. The researchers computed the relative brainwave amplitude (in microvolts, μV) for each subject viewing unpleasant versus neutral pictures. They also recorded how many pictures the subjects were able to recall 24 hours later. The data are displayed in the following scatterplot.
μ 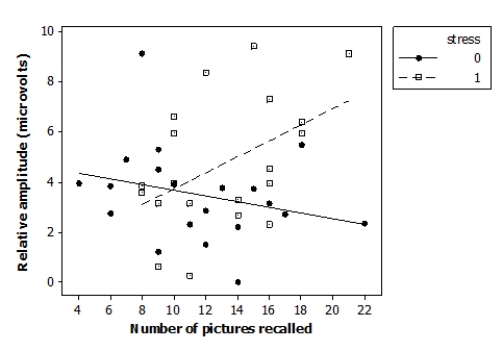The following model is proposed for predicting the brainwave relative amplitude ("amplitude") from the number of images recalled ("recall") , the indicator variable reflecting the nature of the stimulus ("stress") , and an interaction term ("recall*stress") :
Amplitude_i = β₀ + β₁ (recall_i) + β₂ (stress_i) + β₃ (recall*stress_i) + ε_i
Where the deviations ε_i are assumed to be independent and Normally distributed with mean 0 and standard deviation σ. This model was fit to the sample of 40 adult males. The following results summarize the least-squares regression fit of this model:
​
$\begin{array}{lrr}\text { Predictor } & \text { Coef } & \text { SE Coef } \\\text { Constant } & 4.795 & 1.329 \\\text { recall } & -0.1139 & 0.1069 \\\text { stress } & -4.262 & 2.205 \\\text { recall } & 0.4337 & 0.1677 \\\mathrm{~S}=2.13946 & \text { R-Sq }=22.8 \% &\end{array}$

$\begin{array}{l}\text { Analysis of Variance }\\\begin{array}{lrrr}\text { Source } & \text { DF } & \text { SS } & \text { MS } \\\text { Regression } & 3 & 48.788 & 16.263 \\\text { Residual Error } & 36 & 164.783 & 4.577 \\\text { Total } & 39 & 213.571 &\end{array}\end{array}$ Suppose we wish use the ANOVA F test to test the following hypotheses:
H₀: β₁ = β₂ = β₃ = 0
H_α: At least one of β₁ or β₂ or β₃ is not 0
What is the value of the F statistic for these hypotheses?

A) 2.14
B) 3.55
C) 4.58
D) 5.42